A = {a,b,p}, B = {2,3}, C = {p,q,r,s} then n[(A U C) x B] is

Let f and g be two functions given by
f = {(0,1), (2,0), (3,-4), (4,2), (5,7)}
g = {(0,2), (1,0), (2,4), (-4,2), (7,0)} then the  range of f o g is

If A = 2⁶⁵ and B = 2⁶⁴ + 2⁶³ + 2⁶² +...+ 2⁰ Which of the following is true?

For the given matrix A = \(\left( \begin{matrix} 1 \\ 2 \\ 9 \end{matrix}\begin{matrix} 3 \\ 4 \\ 11 \end{matrix}\begin{matrix} 5 \\ 6 \\ 13 \end{matrix}\begin{matrix} 7 \\ 8 \\ 15 \end{matrix} \right) \) the order of the matrix A^T is

The two tangents from an external points P to a circle with centre at O are PA and PB. If \(\angle APB\) = 70^o then the value of \(\angle AOB\) is

In figure if PR is tangent to the circle at P and O is the centre of the circle, then \(\angle PQR\) is

(2, 1) is the point of intersection of two lines.

The value of \(si{ n }^{ 2 }\theta +\frac { 1 }{ 1+ta{ n }^{ 2 }\theta } \) is equal to

tan \(\theta \) cosec²\(\theta \) - tan\(\theta \) is equal to 

\(\frac { tan\theta }{ sec\theta } +\frac { tan\theta }{ sec\theta +1 } \) is equal to

The height of a right circular cone whose radius is 5 cm and slant height is 13 cm will be

When Karuna divided surface area of a sphere by the sphere's volume, he got the answer as \(\frac { 1 }{ 3 } \). What is the radius of the sphere?

If the standard deviation of x, y, z is p then the standard deviation of 3x + 5, 3y + 5, 3z + 5 is

A box contains some milk chocalates and some coco chocolates and there are 60 choolates in the box. If the probability of taking a milk chocolate is \(\frac { 2 }{ 3 } \) then the number of coco chocolates is ___________

Find the value of k, such that f o g = g o f
f(x) = 2x - k, g(x) = 4x + 5

Find the sum of first n terms of the G.P
256, 64, 16,........

Show that any positive odd integer is of the form 4q + 1 or 4q + 3, where q is some integer.

Simplify
\(\frac { x+2 }{ x+3 } +\frac { x-1 }{ x-2 } \)

Prove that the equation x²(a²+b²)+2x(ac+bd)+(c²+ d²) = 0 has no real root if ad≠bc.

Is \(\triangle\)ABC ~ \(\triangle\)PQR?

In figure the line segment xy is parallel to side AC of \(\Delta ABC\) and it divides the triangle int two parts of equal areas. Find the ratio \(\cfrac { AX }{ AB } \)

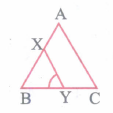
What is the inclination of a line whose slope is \(\sqrt { 3 } \) ?

Find a relation between x and y such that the point (x, y) is equidistant from the points (7, 1) and (3, 5).

prove the following identities
\(\sqrt { \frac { 1+sin\theta }{ 1-sin\theta } } +\sqrt { \frac { 1+sin\theta }{ 1-sin\theta } } =2sec\theta \)

Find the diameter of a sphere whose surface area is 154 m².

If the radii of the circular ends of a conical bucket which is 45 cm high are 28 cm and 7 cm, find the capacity of the bucket. (Use π = \(\frac{22}{7}\))

If the standard deviation of a data is 4.5 and if each value of the data is decreased by 5, then find the new standard deviation.

The marks scored by 5 students in a test for 50 marks are 20, 25, 30, 35, 40. Find the S.D for the marks. If the marks are converted for 100 marks, find the S.D. for newly obtained marks.

A graph representing the function f (x) is given in Fig it is clear that f (9) = 2.
(i) Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
(ii) For what value of x is f (x) = 1?
(iii) Describe the following (i) Domain (ii) Range.
(iv) What is the image of 6 under f ?

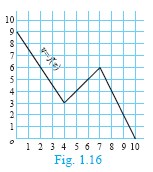
Let A = {1, 2, 3, 4, 5}, B = N and f: A \(\rightarrow\)B be defined by f(x) = x². Find the range of f. Identify the type of function.

How many terms of the series 1³ + 2³ + 3³ +....Should be taken to get the sum 14400?

Determine the AP whose 3rd term is 5 and the 7^th term is 9.

Simplyfy
\(\frac { 4{ x }^{ 2 }y }{ 2{ x }^{ 2 } } \times \frac { 6x{ z }^{ 3 } }{ 20{ y }^{ 4 } } \)

In \(AD\bot BC\) prove that AB² + CD² = BD² + AC². 

A quadrilateral has vertices A(- 4, - 2), B(5, - 1), C(6, 5) and D(- 7, 6). Show that the mid-points of its sides form a parallelogram.

Find the area of a triangle vertices are(1, -1), (-4, 6) and (-3, -5).

The angles of elevation and depression of the top and bottom of a lamp post from the top of a 66 m high apartment are 60° and 30° respectively. Find
The height of the lamp post.

The shadow of a tower, when the angle of elevation of the sum is 45^o is found to be 10 metres, longer than when it is 60^o. find the height of the tower

A hemispherical section is cut out from one face of a cubical block  such that the diameter l of the hemisphere is equal to side length of the cube. Determine the surface area of the remaining solid.

What is the ratio of the volume of a cylinder, a cone, and a sphere. If each has the same diameter and same height?

Find the standard deviation of the following data 7, 4, 8, 10, 11. Add 3 to all the values then find the standard deviation for the new values.

Σx = 99, n = 9, Σ(x - 10)² = 79, then find,
(i) Σx²
(ii) Σ(x - \(\bar { x } \))²