A function f: [-7,6) \(\rightarrow\) R is defined as follows.

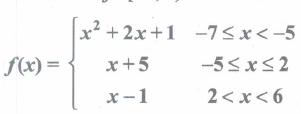
find 2f(-4) + 3f(2)

A function f: [-7,6) \(\rightarrow\) R is defined as follows.

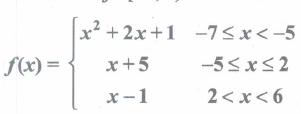
\(\cfrac { 4f(-3)+2f(4) }{ f(-6)-3f(1) } \)

Let A = {1, 2, 3, 4, 5}, B = N and f: A \(\rightarrow\)B be defined by f(x) = x². Find the range of f. Identify the type of function.

A function f: (1,6) \(\rightarrow\)R is defined as follows:

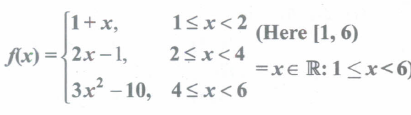
Find the value of f(3),

Which of the following list of numbers form an AP? If they form an AP, write the next two terms:
-2, 2, -2, 2, -2

Determine the AP whose 3rd term is 5 and the 7^th term is 9.

If the sum of the first 14 terms of an AP is 1050 and its first term is 10, find the 20th term.

Find the sum of first 24 terms of the list of numbers whose nth term is given by a_n = 3 + 2n.

A two digit number is such that the product of its digits is 12. When 36 is added to the number the digits interchange their places. Find the number.

A chess board contains 64 equal squares and the area of each square is 6.25 cm², A border round the board is 2 cm wide.

A two digit number is such that the product of its digits is 18, when 63 is subtracted from the number, the digits interchange their places. Find the number.

BL and CM are medians of a triangle ABC right angled at A.
Prove that 4(BL² + CM²) = 5BC².

A ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall and its top reaches a window 6 m above the ground. Find the length of the ladder.

In \(\angle ACD={ 90 }^{ 0 }\) and \(CD\bot AB\) Prove that \(\cfrac { { BC }^{ 2 } }{ { AC }^{ 2 } } =\cfrac { BD }{ AD } \)

Find the coordinates at the points of trisection (i.e. points dividing in three equal parts) of the line segment joining the points A(2, -2) and B(-7, 4).

Find the area of a triangle vertices are(1, -1), (-4, 6) and (-3, -5).

Find the area of the quadrilateral whose vertices, taken in order, are (-4, -2), (-3, -5), (3, -2) and (2, 3).

Find the value of k if the points A(2, 3), B(4, k) and (6, -3) are collinear.

Express the ratios cos A, tan A and sec A in terms of sin A.

If sin 3A = cos (A - 26°), where 3A is an acute angle, find the value at A.

From a point on a bridge across a river, the angles of depression of the banks on opposite sides at the river are 30° and 45°, respectively. If the bridge is at a height at 3 m from the banks, find the width at the river.

P.T (1+tan∝tan∝tanβ)² +(tan∝-tanβ)² =sec² ∝sec²β.

\(P.T\left( \frac { 1+{ tan }^{ 2 }A }{ 1+{ cot }^{ 2 }A } \right) ={ \left( \frac { 1-tan\quad A }{ 1-cot\quad A } \right) }^{ 2 }={ tan }^{ 2 }A\)

The angle of elevation of a tower at a point is 45^o, After going 20 meters towards the foot of the tower the angle of elevation of the tower becomes 60^o calculate the height of the tower.

A spherical ball of iron has been melted and made into small balls. If the radius of each smaller ball is one-fourth of the radius of the original one, how many such balls can be made?