If f(x) = 2 - 3x, then f o f(1 - x) = ?

How many terms are there in the G.P : 5, 20, 80, 320,..., 20480

Which of the following are linear equation in three variables ___________

If triangle PQR is similar to triangle LMN such that 4PQ = LM and QR = 6 cm then MN is equal to ____________

The ratio of the areas of two similar triangles is equal to ____________

Find the value of 'a' if the lines 7y = ax + 4 and 2y = 3 - x are parallel

If tan θ + cot θ = 3 then tan²θ + cot²θ is equal to ___________

The height of a cone is 60 cm. A small cone is cut off at the top by plane parallel to the base and its volume is \(\left[ \frac { 1 }{ 64 } \right] ^{ th }\) the volume of the original cone. Then the height of the smaller cone is ___________

A solid is hemispherical at the bottom and conical above. If the curved surface areas of the two parts are equal, then the ratio of its radius and the height of its conical part is ___________

The mean of first first 10 odd natural number is ___________

Let A = {0, 1, 2, 3} and B = {1, 3, 5, 7, 9} be two sets. Letf: A \(\rightarrow\)B be a function given by  f(x) = 2x + 1. Represent this function as a graph.

Prove that \(\sqrt { 3 } \) is irrational

Find the values of k for which the following equation has equal roots.
(k - 12)r + 2(k - 12)x + 2 = 0

In figure OA· OB = OC·OD
Show that \(\angle A=\angle C\ and\ \angle B=\angle D\)

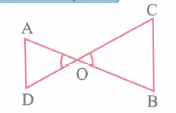
Find a relation between x and y such that the point (x, y) is equidistant from the points (7, 1) and (3, 5).

(sin ∝+cos ∝)(tan ∝+cot ∝)=sec ∝+cosec ∝

If the radii of the circular ends of a conical bucket which is 45 cm high are 28 cm and 7 cm, find the capacity of the bucket. (Use π = \(\frac{22}{7}\))

Find the standard deviation for the following data. 5, 10, 15, 20, 25. And also find the new S.D. if three is added to each value.

f(x) = (1+ x)
g(x) = (2x - 1)
Show that fo(g(x)) = gof(x)

Which of the following list of numbers form an AP? If they form an AP, write the next two terms:
1,-1,-3, -5, ...

Seven years ago, Varun's age was five times the square of swati's age. Three years hence Swati's age will be two fifth of Varun's age. Find their present ages.

BL and CM are medians of a triangle ABC right angled at A.
Prove that 4(BL² + CM²) = 5BC².

If the points A(6, 1), B(8, 2), C(9, 4) and D(P, 3) are the vertices of a parallelogram, taken in order. Find the value of P.

If 15tan² θ+4 sec² θ=23 then find the value of (secθ+cosecθ)² -sin² θ

What is the ratio of the volume of a cylinder, a cone, and a sphere. If each has the same diameter and same height?

Σx = 99, n = 9, Σ(x - 10)² = 79, then find,
(i) Σx²
(ii) Σ(x - \(\bar { x } \))²

If R = {(a, -2), (-5, b), (8, c), (d, -1)} represents the identity function, find the values of a, b, c and 

Find the sum of first 24 terms of the list of numbers whose nth term is given by a_n = 3 + 2n.

Find two consecutive natural numbers whose product is 20.

In figure 0 is any point inside a rectangle ABCD. Prove that OB² + OD² = OA² + OC²

Find the area of the quadrilateral whose vertices, taken in order, are (-4, -2), (-3, -5), (3, -2) and (2, 3).

From a point on a bridge across a river, the angles of depression of the banks on opposite sides at the river are 30° and 45°, respectively. If the bridge is at a height at 3 m from the banks, find the width at the river.

A wooden article was made by scooping out a hemisphere from each end of a cylinder as shown in figure. If the height of the cylinder is 10 cm and its base is of radius 3.5 cm find the total surface area of the article.

Which team is more consistent?