If there are 1024 relations from a set A = {1, 2, 3, 4, 5} to a set B, then the number of elements in B is

If the ordered pairs (a + 2, 4) and (5, 2a + b) are equal then (a,b) is

If the order pairs (a, -1) and (5, b) blongs to {(x, y) | y = 2x + 3}, then a and b are __________

If f(x) = x + 1 then f(f(f(y + 2))) is ___________

If f(x) = 2 - 3x, then f o f(1 - x) = ?

If f is identify function, then the value of f(1) - 2f(2) + f(3) is: 

7^4k \(\equiv \) ________ (mod 100)

The next term of the sequence \(\frac { 3 }{ 16 } ,\frac { 1 }{ 8 } ,\frac { 1 }{ 12 } ,\frac { 1 }{ 18 } \), ..... is

If a and b are the two positive intergs when a > b and b is a factor of a then HCF (a, b) is ____________

Sum of infinite terms of G.P is 12 and the first term is 8. What is the fourth term of the G.P?

If (x - 6) is the HCF of x² - 2x - 24 and x² - kx - 6 then the value of k is

Which of the following can be calculated from the given matrices A  = \(\left( \begin{matrix} 1 & 2 \\ 3 & 4 \\ 5 & 6 \end{matrix} \right) \), B = \(\left( \begin{matrix} 1 & 2 & 3 \\ 4 & 5 & 6 \\ 7 & 8 & 9 \end{matrix} \right) \),
(i) A²
(ii) B²
(iii) AB
(iv) BA

Consider the following statements:
(i) The HCF of x+y and x⁸-y⁸ is x+y
(ii) The HCF of x+y and x⁸+y⁸ is x+y
(iii) The HCF of x-y nd x⁸+y⁸ is x-y
(iv) The HCF of x-y and x⁸-y⁸ is x-y

A Quadratic polynomial whose one zero is 5 and sum of the zeroes is 0 is given by ___________

Axis of symmetry in the term of vertical line seperates parabola into ___________

Two poles of heights 6 m and 11 m stand vertically on a plane ground. If the distance between their feet is 12 m, what is the distance between their tops?

A tangent is perpendicular to the radius at the

If the angle between two radio of a circle is ^o, the angle between the tangents at the end of the radii is ____________

In the given figure if OC = 9 cm and OB = 15 cm then OB + BD is equal to ____________

Two concentric circles if radii a and b where a>b are given. The length of the chord of the circle which touches the smaller circle is ____________

The point of intersection of 3x − y = 4 and x + y = 8 is

If A is a point on the Y axis whose ordinate is 8 and B is a point on the X axis whose abscissae is 5 then the equation of the line AB is

The area of triangle formed by the points (a, b+c), (b, c+a) and (c, a+b) is ____________

Find the value of P, given that the line  \(\frac { y }{ 2 } =x-p\) passes through the point (-4, 4) is ____________

If 5x = sec\(\theta \) and \(\frac { 5 }{ x } \) = tan\(\theta \), then x² - \(\frac { 1 }{ { x }^{ 2 } } \) is equal to 

If the ratio of the height of a tower and the length of its shadow is \(\sqrt{3}: 1\), then the angle of elevation of the sun has measure

If m cos θ + n sin θ = a and m sin θ - n cos θ = b then a² + b² is equal to ___________

The value of \(\cfrac { 3 }{ cot^{ 2 }\theta } -\cfrac { 3 }{ { cos }^{ 2 }\theta } \) is equal to ___________

If x = a sec θ and = b tan θ, then b²x² - a²y² is equal to ___________

A frustum of a right circular cone is of height 16 cm with radii of its ends as 8 cm and 20 cm. Then, the volume of the frustum is

A shuttle cock used for playing badminton has the shape of the combination of

The volume of a frustum if a cone of height L and ends-radio and r₁ and r₂ is ___________

A solid frustum is of height 8 cm. If the radii of its lower and upper ends are 3 cm and 9 cm respectively, then its slant height is ___________

Kamalam went to play a lucky draw contest 135 tickets of the lucky draw were sold. If the probability of Kamalam winning is \(\frac { 1 }{ 9 } \), then the number of tickets bought by kamalam is ____________

If a letter is chosen at random from the English alphabets {a, b....,z}, then the probability that the letter chosen precedes x ____________

The standard deviation of a data is 3. If each value is multiplied by 5 then the new variance is

A purse contains 10 notes of Rs. 2000, 15 notes of Rs. 500, and 25 notes of Rs. 200. One note is drawn at random. What is the probability that the note is either a Rs. 500 note or Rs. 200 note?

A number x is chosen at random from -4, -3, -2, -1, 0, 1, 2, 3, 4 find the probability that |x| ≤ 4

If the data is multiplied by 4, then the corresponding variances is get multiplied by ___________

When three coins are tossed, the probability of getting the same face on all the three coins is ___________

A relation ‘f’ \(X \rightarrow Y\) is defined by f(x) = x² - 2 where x \(\in \) {-2, -1, 0, 3} and Y = R
(i) List the elements of f
(ii) Is f a function?

Let A =  {1,2, 3, 4} and B = {-1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12} Let R = {(1, 3), (2, 6), (3, 10), (4, 9)} \(\subseteq \) A x B bea relation. Show that R is a function and find its domain, co-domain and the range of R.

Let A = {0, 1, 2, 3} and B = {1, 3, 5, 7, 9} be two sets. Letf: A \(\rightarrow\)B be a function given by  f(x) = 2x + 1. Represent this function as  a table .

Let A = {0, 1, 2, 3} and B = {1, 3, 5, 7, 9} be two sets. Letf: A \(\rightarrow\)B be a function given by  f(x) = 2x + 1. Represent this function as a graph.

Let A = {1,2,3,7} and B = {3,0,–1,7}, which of the following are relation from A to B ?
R₄ = {(7,–1), (0, 3), (3, 3), (0, 7)

Write an A.P. whose first term is 20 and common difference is 8.

Find the sum of first n terms of the G.P
5, -3, \(\frac { 9 }{ 5 } ,-\frac { 27 }{ 25 } \),...,

Solve x² - 3x - 2 = 0

Determine the quadratic equations, whose sum and product of roots are
\(\frac {-3}{2}\), -1

If figure OPRQ is a square and \(\angle\)MLN = 90^o. Prove that
\(\triangle\)LOP ~\(\triangle\)QMO

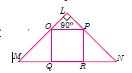
Check whether AD is bisector \(\angle\)A of \(\triangle\)ABC in each of the following AB = 4cm, AC = 6cm, BD = 1.6cm and CD = 2.4cm.

Find the slope of a line joining the given points (- 6, 1) and (-3, 2)

Show that the given points are collinear: (-3, -4) , (7, 2) and (12, 5)

Find the slope of the line which is perpendicular to 2x - 3y + 8 = 0

prove the following identity.
 \(\sqrt { \frac { 1+sin\theta }{ 1-sin\theta } } =sec\theta +tan\theta\)

calculate \(\angle \)BAC in the given triangles (tan 38.7° = 0.8011 )

A garden roller whose length is 3 m long and whose diameter is 2.8 m is rolled to level a garden. How much area will it cover in 8 revolutions?

A metallic sphere of radius 16 cm is melted and recast into small spheres each of radius 2 cm. How many small spheres can be obtained?

Find the range of the following distribution..

Calculate the range of the following data..

Let f = {(2, 7); (3, 4), (7, 9), (-1, 6), (0, 2), (5,3)} be a function from A = {-1,0, 2, 3, 5, 7} to B = {2, 3, 4, 6, 7, 9}. Is this
(i) an one-one function
(ii) an onto function,
(iii) both oneone and onto function?

Let A = {1, 2, 3, 4, 5}, B = N and f: A \(\rightarrow\)B be defined by f(x) = x². Find the range of f. Identify the type of function.

If the sum of the first 14 terms of an AP is 1050 and its first term is 10, find the 20th term.

Find the values of a and b if the following polynomials are perfect squares
4x⁴ - 12x³ + 37x² + bx + a

Simplify
\(\frac { { b }^{ 2 }+3b-28 }{ { b }^{ 2 }+4b+4 } \div \frac { { b }^{ 2 }-49 }{ { b }^{ 2 }-5b-14 } \)

Find the square root of the following
(4x² - 9x + 2)(7x² - 13x - 2)(28x² - 3x - 1)

A two digit number is such that the product of its digits is 12. When 36 is added to the number the digits interchange their places. Find the number.

Rhombus \(\triangle\)QRB is inscribed in \(\triangle\)ABC such that \(\angle\)B is one of its angle. P, Q and R lie on AB, AC and BC respectively. If AB = 12 cm and BC = 6 cm, find the sides PQ, RB of the rhombus.

O is any point inside a triangle ABC. The bisector of \(\angle AOB\), \(\angle BOC\) and \(\angle COA\) meet the sides AB,BC and CA in point D, E and F resopectively.Show that AD x BE x CF = DB x EC x FA

If vertices of quadrilateral are at A(-5, 7), B(-4, k) , C(-1, -6) and D(4, 5) and its area is 72 sq.units. Find the value of k.

Prove that sin² Acos² B + cos² Asin² B + cos² Acos² B + sin² Asin² B=1

prove that \(\frac { sinA }{ secA+tanA-1 } +\frac { cosA }{ cosecA+cotA-1 } =1\)

A man is standing on the deck of a ship, which is 40 m above water level. He observes the angle of elevation of the top of a hill as 60° and the angle of depression of the base of the hill as 30° . Calculate the distance of the hill from the ship and the height of the hill. (\(\sqrt { 3 } \) = 1.732)

The shadow of a tower, when the angle of elevation of the sum is 45^o is found to be 10 metres, longer than when it is 60^o. find the height of the tower

The internal and external diameters of a hollow hemispherical vessel are 20 cm and 28 cm respectively. Find the cost to paint the vessel all over at Rs. 0.14 per cm².

From a solid cylinder whose height is 2.4 cm and the diameter 1.4 cm, a cone of the same height and same diameter is carved out. Find the volume of the remaining solid to the nearest cm³.

A hollow metallic cylinder whose external radius is 4.3 cm and internal radius is 1.1 cm and whole length is 4 cm is melted and recast into a solid cylinder of 12 cm long. Find the diameter of solid cylinder.

For a group of 100 candidates the mean and standard deviation of their marks were found to be 60 and 15 respectively. Later on it was found that the scores 45 and 72 were wrongly entered as 40 and 27. Find the correct mean and standard deviation.

The standard deviation of some temperature data in degree celsius (⁰C) is 5. If the data were converted into degree Farenheit (⁰F) then what is the variance?

Prices of peanut packets in various places of two cities are given below. In which city, prices were more stable?

Find the domain of the function f(x) = \(\sqrt { 1+\sqrt { 1-\sqrt { 1-x^{ 2 } } } } \).

How many terms of the AP: 24, 21, 18, ... must be taken so that their sum is 78?

Find two consecutive natural numbers whose product is 20.

Construct a triangle similar to a given triangle LMN with its sides equal to \(\frac { 4 }{ 5 } \) of the corresponding sides of the triangle LMN (scale factor \(\frac { 4 }{ 5 }<1\)).

Draw the two tangents from a point which is 10 cm away from the centre of a circle of radius 5 cm. Also, measure the lengths of the tangents.

Find the equation of a straight line Passing through (-8, 4) and making equal intercepts on the coordinate axes

Find the value of k if the points A(2, 3), B(4, k) and (6, -3) are collinear.

From a point on a bridge across a river, the angles of depression of the banks on opposite sides at the river are 30° and 45°, respectively. If the bridge is at a height at 3 m from the banks, find the width at the river.

A wooden article was made by scooping out a hemisphere from each end of a cylinder as shown in figure. If the height of the cylinder is 10 cm and its base is of radius 3.5 cm find the total surface area of the article.

S.D. of a data is 2102, mean is 36.6, then find its C.V.