The function t which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined Fahrenheit degree is 95, then the value of  C \(t(C)=\frac { 9c }{ 5 } +32\) is ___________

Sum of first n terms of the series \(\sqrt { 2 } +\sqrt { 8 } +\sqrt { 18 } +...\) is ____________

If \(2A+3B=\left[ \begin{matrix} 2 & -1 & 4 \\ 3 & 2 & 5 \end{matrix} \right] \) and \(A+2B=\left[ \begin{matrix} 5 & 0 & 3 \\ 1 & 6 & 2 \end{matrix} \right] \) then B = [hint: B = (A+2B)-(2+3B)]

The ratio of the areas of two similar triangles is equal to ____________

Two concentric circles if radii a and b where a>b are given. The length of the chord of the circle which touches the smaller circle is ____________

The y-intercept of the line 3x - 4y + 8 = 0 is ___________

The value of the expression \(\left[ \frac { { sin }^{ 2 }{ 22 }^{ o }+{ sin }^{ 2 }{ 68 }^{ o } }{ { cos }^{ 2 }{ 22 }^{ 0 }+{ cos }^{ 2 }{ 68 }^{ 0 } } +{ sin }^{ 2 }{ 63 }^{ o+ }{ cos }63^{ 0 }{ sin27 }^{ 0 } \right] \)is ___________

A cylinder 10 cone and have there are of a equal base and have the same height. what is the ratio of there volumes?

It S₁ denotes the total surface area of a sphere of radius r and S₂ denotes the total surface area of a cylinder of base radius r and height 2r, then ___________

The range of first 10 prime number is ___________

Let A = {0, 1, 2, 3} and B = {1, 3, 5, 7, 9} be two sets. Let f: A \(\rightarrow\)B be a function given by  f(x) = 2x + 1. Represent this function as a graph.

Prove that \(\sqrt { 3 } \) is irrational

Using quadratic formula solve the following equations.9x²-9(a+b)x+(2a²+5ab+2b²)=0

In figure if PQ || RS Prove that \(\Delta POQ\sim \Delta SOQ\)

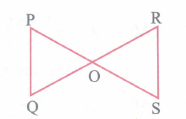
Find a relation between x and y such that the point (x, y) is equidistant from the points (7, 1) and (3, 5).

If the radii of the circular ends of a conical bucket which is 45 cm high are 28 cm and 7 cm, find the capacity of the bucket. (Use π = \(\frac{22}{7}\))

Find the standard deviation of 30, 80, 60, 70, 20, 40, 50 using the direct method.

Let A = {1, 2, 3, 4, 5}, B = N and f: A \(\rightarrow\)B be defined by f(x) = x². Find the range of f. Identify the type of function.

Which of the following list of numbers form an AP? If they form an AP, write the next two terms:
1,-1,-3, -5, ...

A chess board contains 64 equal squares and the area of each square is 6.25 cm², A border round the board is 2 cm wide.

In \(AD\bot BC\) prove that AB² + CD² = BD² + AC². 

Find the area of a triangle vertices are(1, -1), (-4, 6) and (-3, -5).

If sin (A - B) = \(\frac12\),  cos (A + B) = \(\frac12\), 0^o < A + ≤  90°, A > B, find A and B.

Find the number of coins, 1.5 cm is diameter and 0.2 cm thick, to be melted to form a right circular cylinder of height 10 cm and diameter 4.5 cm.

Find the co-efficient of variation for the following data: 16, 13, 17,21, 18.

A function f: (1,6) \(\rightarrow\)R is defined as follows:

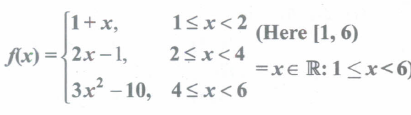
Find the value of f(3),

How many terms of the AP: 24, 21, 18, ... must be taken so that their sum is 78?

Find two consecutive natural numbers whose product is 20.

A ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall and its top reaches a window 6 m above the ground. Find the length of the ladder.

Find the value of k if the points A(2, 3), B(4, k) and (6, -3) are collinear.

From a point on a bridge across a river, the angles of depression of the banks on opposite sides at the river are 30° and 45°, respectively. If the bridge is at a height at 3 m from the banks, find the width at the river.

A wooden article was made by scooping out a hemisphere from each end of a cylinder as shown in figure. If the height of the cylinder is 10 cm and its base is of radius 3.5 cm find the total surface area of the article.

S.D. of a data is 2102, mean is 36.6, then find its C.V.