Let A = {1, 2, 3, 4} and B = {4, 8, 9, 10}. A function f: A ⟶ B given by f = {(1, 4), (2, 8), (3, 9), (4,10)} is a

(a)

Many-one function

(b)

Identity function

(c)

One-to-one function

(d)

Into function

If f(x) = 2x² and g(x) = \(\frac{1}{3x}\), then f o g is

(a)

\(\\ \frac { 3 }{ 2x^{ 2 } } \)

(b)

\(\\ \frac { 2 }{ 3x^{ 2 } } \)

(c)

\(\\ \frac { 2 }{ 9x^{ 2 } } \)

(d)

\(\\ \frac { 1 }{ 6x^{ 2 } } \)

If function f : N⟶N, f(x) = 2x then the function is, then the function is ___________

(a)

Not one - one and not onto

(b)

one-one and onto

(c)

Not one -one but not onto

(d)

one - one but not onto

If 6 times of 6^th term of an A.P. is equal to 7 times the 7^th term, then the 13^th term of the A.P. is

(a)

0

(b)

6

(c)

7

(d)

13

An A.P. consists of 31 terms. If its 16^th term is m, then the sum of all the terms of this A.P. is

(a)

16 m

(b)

62 m

(c)

31 m

(d)

\(\frac { 31 }{ 2 } \) m

The difference between the remainders when 6002 and 601 are divided by 6 is ____________

(a)

2

(b)

1

(c)

0

(d)

3

The solution of (2x - 1)² = 9 is equal to

(a)

-1

(b)

2

(c)

-1, 2

(d)

None of these

The values of a and b if 4x⁴ - 24x³ + 76x² + ax + b is a perfect square are

(a)

100, 120

(b)

10, 12

(c)

-120, 100

(d)

12, 10

Consider the following statements:
(i) The HCF of x+y and x⁸-y⁸ is x+y
(ii) The HCF of x+y and x⁸+y⁸ is x+y
(iii) The HCF of x-y nd x⁸+y⁸ is x-y
(iv) The HCF of x-y and x⁸-y⁸ is x-y

(a)

(i) and (ii)

(b)

(ii) and (iii)

(c)

(i) and (iv)

(d)

(ii) and (iv)

Two poles of heights 6 m and 11 m stand vertically on a plane ground. If the distance between their feet is 12 m, what is the distance between their tops?

(a)

13 m

(b)

14 m

(c)

15 m

(d)

12.8 m

In the given figure, PR = 26 cm, QR = 24 cm, \(\angle PAQ\) = 90^o, PA = 6 cm and QA = 8 cm. Find \(\angle\)PQR

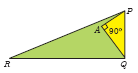(a)

80^o

(b)

85^o

(c)

75^o

(d)

90^o

The ratio of the areas of two similar triangles is equal to ____________

(a)

The ratio of their corresponding sides

(b)

The cube of the ratio of their corresponding sides

(c)

The ratio of their corresponding attitudes

(d)

The square of the ratio of their corresponding sides

If A is a point on the Y axis whose ordinate is 8 and B is a point on the X axis whose abscissae is 5 then the equation of the line AB is

(a)

8x + 5y = 40

(b)

8x - 5y = 40

(c)

x = 8

(d)

y = 5

The equation of a line passing through the origin and perpendicular to the line 7x - 3y + 4 = 0 is

(a)

7x - 3y + 4 = 0

(b)

3x - 7y + 4 = 0

(c)

3x + 7y = 0

(d)

7x - 3y = 0

The four vertices of a quadrilateral are (1, 2), (5, -6), (7, -4) and (k, -2) taken in order. If the area of quadrilateral is zero then find the value of k.

(a)

4

(b)

-2

(c)

6

(d)

3

If the ratio of the height of a tower and the length of its shadow is \(\sqrt{3}: 1\), then the angle of elevation of the sun has measure

(a)

45°

(b)

30°

(c)

90°

(d)

60°

The electric pole subtends an angle of 30° at a point on the same level as its foot. At a second point ‘b’ metres above the first, the depression of the foot of the pole is 60°. The height of the pole (in metres) is equal to

(a)

\(\sqrt { 3 } \) b

(b)

\(\frac { b }{ 3 } \)

(c)

\(\frac { b }{ 2 } \)

(d)

\(\frac { b }{ \sqrt { 3 } } \)

If cos (∝ - β) =, then sin (∝ - β)  can be reduced to ___________

(a)

cos β

(b)

cos 2β

(c)

sin ∝

(d)

sin 2∝

The value of \(\cfrac { 3 }{ cot^{ 2 }\theta } -\cfrac { 3 }{ { cos }^{ 2 }\theta } \) is equal to ___________

(a)

\(\frac { 1 }{ 3 } \)

(b)

3

(c)

0

(d)

-3

A solid sphere of radius x cm is melted and cast into a shape of a solid cone of same radius. The height of the cone is

(a)

3x cm

(b)

x cm

(c)

4x cm

(d)

2x cm

A frustum of a right circular cone is of height 16 cm with radii of its ends as 8 cm and 20 cm. Then, the volume of the frustum is

(a)

3328\(\pi\) cm³

(b)

3228\(\pi\) cm³

(c)

3240\(\pi\) cm³

(d)

3340\(\pi\) cm³

The volume of a frustum if a cone of height L and ends-radio and r₁ and r₂ is ___________

(a)

\(\frac{1}{3}\)πh1(r₁²+r₂²+r₁r₂)

(b)

\(\frac{1}{3}\)πh(r₁²+r₂²-r₁r₂)

(c)

πh(r₁²+r₂²+r₁r₂)

(d)

πh(r₁²+r₂²-r₁r₂)

Which of the following is incorrect?

(a)

P(A) > 1

(b)

0 ≤ P(A) ≤ 1

(c)

P(ф) = 0

(d)

P(A) + P(\(\bar { A } \)) = 1

The probability a red marble selected at random from a jar containing p red, q blue and r green marbles is

(a)

\(\frac { q }{ p+q+r } \)

(b)

\(\frac { p }{ p+q+r } \)

(c)

\(\frac { p+q }{ p+q+r } \)

(d)

\(\frac { p+r }{ p+q+r } \)

which of the following is true?

(a)

0 ≤ p(∈) ≤ 1

(b)

p(∈) > 1

(c)

p(∈) < 0

(d)

\(-\frac { 1 }{ 2 } \ge P(\in )\le \frac { 1 }{ 2 } \)