Let n(A) = m and n(B) = n then the total number of non-empty relations that can be defined from A to B is

(a)

mⁿ

(b)

n^m

(c)

2^mn-1

(d)

2^mn

If {(a, 8 ),(6, b)}represents an identity function, then the value of a and b are respectively

(a)

(8,6)

(b)

(8,8)

(c)

(6,8)

(d)

(6,6)

If the order pairs (a, -1) and (5, b) belongs to {(x, y) | y = 2x + 3}, then a and b are __________

(a)

-13, 2

(b)

2, 13

(c)

2, -13

(d)

-2,13

Given F₁ = 1, F₂ = 3 and F_n = F_n-1 + F_n-2 then F₅ is

(a)

3

(b)

5

(c)

8

(d)

11

The first term of an arithmetic progression is unity and the common difference is 4. Which of the following will be a term of this A.P.

(a)

4551

(b)

10091

(c)

7881

(d)

13531

If 3 is the least prime factor of number 'a' and 7 is least prime factor of number 'b', then the least prime factor of a + b is ____________

(a)

a + b

(b)

2

(c)

5

(d)

10

The square root of \(\frac { 256{ x }^{ 8 }{ y }^{ 4 }{ z }^{ 10 } }{ 25{ x }^{ 6 }{ y }^{ 6 }{ z }^{ 6 } } \) is equal to

(a)

\(\frac { 16 }{ 5 } \left| \frac { { x }^{ 2 }{ z }^{ 4 } }{ { y }^{ 2 } } \right| \)

(b)

\(16\left| \frac { { y }^{ 2 } }{ { x }^{ 2 }{ z }^{ 4 } } \right| \)

(c)

\(\frac { 16 }{ 5 } \left| \frac { y }{ x{ z }^{ 2 } } \right| \)

(d)

\(\frac { 16 }{ 5 } \left| \frac { x{ z }^{ 2 } }{ y } \right| \)

Which of the following should be added to make x⁴ + 64 a perfect square

(a)

4x²

(b)

16x²

(c)

8x²

(d)

-8x²

The HCF of two polynomials p(x) and q(x) is 2x(x + 2) and LCM is 24x(x + 2)² (x - 2) if p(x) = 8x³ + 32x² + 32x, then q(x) ___________

(a)

4x³-16x

(b)

6x³-24x

(c)

12x³+24x

(d)

12x³-24x

In a \(\triangle\)ABC, AD is the bisector \(\angle\)BAC. If AB = 8 cm, BD = 6 cm and DC = 3 cm. The length of the side AC is

(a)

6 cm

(b)

4 cm

(c)

3 cm

(d)

8 cm

In the adjacent figure \(\angle BAC\) = 90^o and AD\(\bot \)BC then 

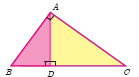(a)

BD.CD = BC²

(b)

AB.AC = BC²

(c)

BD.CD = AD²

(d)

AB.AC = AD²

In the given figure DE||BC:BD = x - 3, BA = 2x,CE = x- 2, and AC = 2x + 3, Find the value of x.

(a)

3

(b)

6

(c)

9

(d)

12

The slope of the line which is perpendicular to a line joining the points (0, 0) and (– 8, 8) is

(a)

–1

(b)

1

(c)

\(\frac13\)

(d)

-8

If slope of the line PQ is \(\frac { 1 }{ \sqrt { 3 } } \) then slope of the perpendicular bisector of PQ is

(a)

\(\sqrt { 3 } \)

(b)

\(-\sqrt { 3 } \)

(c)

\(\frac { 1 }{ \sqrt { 3 } } \)

(d)

0

The area of triangle formed by the points (a, b+c), (b, c+a) and (c, a+b) is ____________

(a)

a+b+c

(b)

abc

(c)

(a+b+c)²

(d)

0

(1 + tan \(\theta \) + sec\(\theta \)) (1 + cot\(\theta \) - cosec\(\theta \)) is equal to 

(a)

0

(b)

1

(c)

2

(d)

-1

a cot \(\theta \) + b cosec\(\theta \) = p and b cot \(\theta \) + a cosec\(\theta \) = q then p²- q² is equal to 

(a)

a² - b²

(b)

b² - a²

(c)

a² + b²

(d)

b - a

Given that sinθ = \(\frac{a}{b}\), then cosθ is equal to ___________

(a)

\(\frac { b }{ \sqrt { { b }^{ 2 }-{ a }^{ 2 } } } \)

(b)

\(\frac { b }{ a } \)

(c)

\(\frac { \sqrt { { b }^{ 2 }-{ a }^{ 2 } } }{ b } \)

(d)

\(\frac { b }{ \sqrt { { b }^{ 2 }-{ a }^{ 2 } } } \)

If the radius of the base of a cone is tripled and the height is doubled then the volume is

(a)

made 6 times

(b)

made 18 times

(c)

made 12 times

(d)

unchanged

The total surface area of a hemi-sphere is how much times the square of its radius.

(a)

\(\pi\)

(b)

4\(\pi\)

(c)

3\(\pi\)

(d)

2\(\pi\)

How many balls, each of radius 1 cm, can be made from a solid sphere of lead of radius cm?

(a)

64

(b)

216

(c)

512

(d)

16

A spherical steel ball is melted to make 8 new identical balls. Then the radius each new ball is how much times the radius of the original ball?

(a)

\(\frac { 1 }{ 3 } \)

(b)

\(\frac { 1 }{ 4 } \)

(c)

\(\frac { 1 }{ 2 } \)

(d)

\(\frac { 1 }{ 8 } \)

If the standard deviation of x, y, z is p then the standard deviation of 3x + 5, 3y + 5, 3z + 5 is

(a)

3p + 5

(b)

3p

(c)

p + 5

(d)

9p + 15

If the mean and coefficient of variation of a data are 4 and 87.5% then the standard deviation is

(a)

3.5

(b)

3

(c)

4.5

(d)

2.5

A number x is chosen at random from -4, -3, -2, -1, 0, 1, 2, 3, 4 find the probability that |x| ≤ 4

(a)

0

(b)

1

(c)

\(\frac{1}{2}\)

(d)

\(\frac{1}{9}\)