If n(A x B) = 6 and A = {1,3} then n(B) is

f(x) = (x + 1)³ - (x - 1)³ represents a function which is

If the HCF of 65 and 117 is expressible in the form of 65m - 117 , then the value of m is

A system of three linear equations in three variables is inconsistent if their planes

If \(\triangle\)ABC is an isosceles triangle with \(\angle\)C = 90^o and AC = 5 cm, then AB is

A tangent is perpendicular to the radius at the

If (5, 7), (3, p) and (6, 6) are collinear, then the value of p is

tan \(\theta \) cosec²\(\theta \) - tan\(\theta \) is equal to 

The electric pole subtends an angle of 30° at a point on the same level as its foot. At a second point ‘b’ metres above the first, the depression of the foot of the pole is 60°. The height of the pole (in metres) is equal to

The value of the expression [cosec (75^o + θ) - sec (15^o - θ) - tan (55^o + θ) + cot(35^o - θ] is ___________

A pole 6 m high a shadow 2\(\sqrt{3}\) m long on the ground, then the sun's elevation is ___________

In a hollow cylinder, the sum of the external and internal radii is 14 cm and the width is 4 cm. If its height is 20 cm, the volume of the material in it is

The range of the data 8, 8, 8, 8, 8. . . 8 is

A purse contains 10 notes of Rs. 2000, 15 notes of Rs. 500, and 25 notes of Rs. 200. One note is drawn at random. What is the probability that the note is either a Rs. 500 note or Rs. 200 note?

If A x B = {(3,2), (3, 4), (5,2), (5, 4)} then find A and B.

Find the HCF of 396, 504, 636.

Find the sum of
1² + 2² +...+ 19²

Solve x + 2y - z = 5; x - y + z = -2; -5x - 4y + z = -11

If A = \(\left[ \begin{matrix} 1 & 2 & 0 \\ 3 & 1 & 5 \end{matrix} \right] \), B = \(\left[ \begin{matrix} 8 & 3 & 1 \\ 2 & 4 & 1 \\ 5 & 3 & 1 \end{matrix} \right] \), find AB.

Show that the points P(-1, 5, 3), Q(6, -2) , R(-3, 4) are collinear.

A line makes positive intercepts on coordinate axes whose sum is 7 and it passes through (-3,8). Find its equation

if cosec\(\theta \) + cot\(\theta \) = p, then prove that cos\(\theta \) = \(\frac { { p }^{ 2 }-1 }{ { p }^{ 2 }+1 } \)

The radius of a conical tent is 7 m and the height is 24 m. Calculate the length of the canvas used to make the tent if the width of the rectangular canvas is 4 m?

The number of televisions sold in each day of a week are 13, 8, 4, 9, 7, 12, 10. Find its standard deviation.

Let A = {3,4,7,8} and B = {1,7,10}. Which of the following sets are relations from A to B?
R₁ = {(3,7), (4,7), (7,10), (8,1)}

Can the number 6ⁿ, n being a natural number end with the digit 5 ? Give reason for your answer.

Find the sum of all natural numbers between 300 and 600 which are divisible by 7.

Determine the nature of roots for the following quadratic equation. 2x² - x - 1 = 0

Find the LCM of the following
x³ - 27, (x - 3)², x² - 9.

In the figure, AD is the bisector of \(\angle\)A. If BD = 4 cm, DC = 3 cm and AB = 6 cm, find AC.

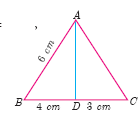
Draw a circle of radius 4 cm. At a point L on it draw a tangent to the circle using the alternate segment.

Show that the straight lines 2x + 3y - 8 = 0 and 4x + 6y + 18 = 0 are parallel.

A tower stands vertically on the ground. from a point on the ground,which is 48m away from the foot of the tower, the angel of elevation of the top of  the tower is 30°.find the hieght of the tower.

Find the volume of a cylinder whose height is 2 m and whose base area is 250 m².

Let A = {1, 2, 3, 7} and B = {3, 0, –1, 7}, which of the following are relation from A to B ?
R₁ = {(2, 1), (7,1)}

Using the functions f and g given below, find f o g and g o f. Check wheather f o g = g o f
 f(x) = 3 + x, g(x) = x - 4