If A \(=\begin{pmatrix} -1 & 2 \\ 1 & -4 \end{pmatrix}\) then A (adj A) is ________.

(a)

\(\begin{pmatrix} -4 & -2 \\ -1 & -1 \end{pmatrix}\)

(b)

\(\begin{pmatrix} 4 & -2 \\ -1 & 1 \end{pmatrix}\)

(c)

\(\begin{pmatrix} 2 & 0 \\ 0 & 2 \end{pmatrix}\)

(d)

\(\begin{pmatrix} 0 & 2 \\ 2 & 0 \end{pmatrix}\)

The value of \(\begin{vmatrix} x & x^2 & -yz & 1 \\ y & y^2 & -zx & 1 \\ z & z^2 & -xy &1 \end{vmatrix}\) is ________.

(a)

1

(b)

0

(c)

-1

(d)

-xyz

The number of ways selecting 4 players out of 5 is _______.

(a)

4!

(b)

20

(c)

25

(d)

5

The value of (5C₀ + 5C₁) + (5C₁ + 5C₂) + (5C₂ + 5C₃) + (5C₃ + 5C₄) + (5C₄ + 5C₅ ) is ________.

(a)

2⁶-2

(b)

2⁵-1

(c)

2⁸

(d)

2⁷

(1, - 2) is the centre of the circle x² + y² + ax + by - 4 = 0 , then its radius _______.

(a)

3

(b)

2

(c)

4

(d)

1

The equation of directrix of the parabola y² = - x is _______.

(a)

4x+ 1 =0

(b)

4x - 1 = 0

(c)

x - 4=0

(d)

x + 4 = 0

The radian measure of 37^o30^' is ______.

(a)

\(\frac{5\pi}{24}\)

(b)

\(\frac{3\pi}{24}\)

(c)

\(\frac{7\pi}{24}\)

(d)

\(\frac{9\pi}{24}\)

\(\left(\frac{\cos x}{cosec x}\right)-\sqrt{1-\sin^2x}\sqrt{1-\cos^2x}\) is _______.

(a)

cos²x-sin²x

(b)

sin²x-cos²x

(c)

1

(d)

0

\(\frac{d}{dx}(\frac{1}{x})\) is equal to ________.

(a)

\(-\frac{1}{x^2}\)

(b)

\(-\frac{1}{x}\)

(c)

log x

(d)

\(\frac{1}{x^2}\)

\(\frac{d}{dx}(5e^x-2logx)\) is equal to ________.

(a)

5e^x - \(\frac{2}{x}\)

(b)

5e^x - 2x

(c)

5e^x - \(\frac{1}{x}\)

(d)

2 logx

Marginal revenue of the demand function p = 20–3x is _______.

(a)

20–6x

(b)

20–3x

(c)

20+6x

(d)

20+3x

If demand and the cost function of a firm are p = 2–x and c = 2x² + 2x + 7 then its profit function is ________.

(a)

x² + 7

(b)

x² - 7

(c)

- x² + 7

(d)

- x² - 7

The brokerage paid by a person on the sale of 400 shares of face value Rs.100 at 1% brokerage ________.

(a)

Rs. 600

(b)

Rs. 500

(c)

Rs. 200

(d)

Rs. 400

If ‘a’ is the annual payment, ‘n’ is the number of periods and ‘i’ is compound interest for Rs. 1 then future amount of the annuity is  _______.

(a)

A = \(\frac{a}{i}(1+i)(1+i)^n-1]\)

(b)

A = \(\frac{a}{i}[(1+i)^n-1]\)

(c)

P = \(\frac{a}{i}\)

(d)

P = \(\frac{a}{i}(1+i)[1-(1+i)^{-n}]\)

Harmonic mean is better than other means if the data are for _________.

(a)

Speed or rates.

(b)

Heights or lengths.

(c)

Binary values like 0 and 1

(d)

Ratios or proportions.

The probability of drawing a spade from a pack of card is _________.

(a)

1/52

(b)

1/13

(c)

4/13

(d)

1/4

Correlation co-efficient lies between ______.

(a)

0 to ∞

(b)

-1 to +1

(c)

-1 to 0

(d)

-1 to ∞

If r(X,Y) = 0 the variables X and Y are said to be ______.

(a)

Positive correlation

(b)

Negative correlation

(c)

No correlation

(d)

Perfect positive correlation

The critical path of the following network is________.

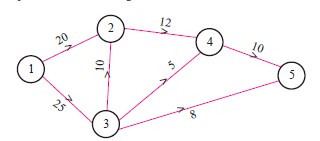(a)

1 – 2 – 4 – 5

(b)

1 – 3 – 5

(c)

1 – 2 – 3 – 5

(d)

1 – 2 – 3 – 4 – 5

Maximize: z = 3x₁ + 4x₂ subject to 2x₁ + x₂ ≤ 40, 2x₁+ 5x₂ ≤ 180, x₁, x₂ ≥ 0. In the LPP, which one of the following is feasible corner point?

(a)

x₁ = 18, x₂ = 24

(b)

x₁ = 15, x₂ = 30

(c)

x₁ = 2.5, x₂ = 35

(d)

x₁ = 20.5, x₂ = 19

The technology matrix of an economic system of two industries is\(\begin{bmatrix} 0.6 & 0.9 \\ 0.20 & 0.80 \end{bmatrix}\) .Test whether the system is viable as per Hawkins-Simon conditions.

Resolve into partial fractions for the following : \(\frac{3 x+7}{x^2-3 x+2}\)

Show that perpendicular distances of the line x - y + 5 = 0 from origin and from the point P(2, 2) are equal.

Find the principal value of \(\cos^{-1}\left(\frac{-1}{\sqrt2}\right)\)

Evaluate: \(\underset { x\rightarrow 1 }{ lim } \frac { { x }^{ 3 }-1 }{ x-1 } \)

If f(x,y) = 3x² + 4y³ + 6xy - x²y³ + 6. Find f_x(1, -1)

How much will be required to buy 125 of Rs. 25 shares at a discount of Rs. 7

A die is thrown. Find the probability of getting
(i) a prime number
(ii) a number greater than or equal to 3

Calculate the correlation coefficient from the following data
N = 9, ΣX = 45, ΣY = 108, ΣX² = 285, ΣY² = 1356, ΣXY = 597

A producer has 30 and 17 units of labour and capital respectively which he can use to produce two types of goods X and Y. To produce one unit of X, 2 unit of labour and 3 units of capital are required. Similarly, 3 units of labour and 1 unit of capital is required to produce one unit of Y. If X and Yare priced at HOO and H20 per unit respectively, how should the producer use his resources to maximize the total revenue? Formulate the LPP for the above.

If \({ A }^{ -1 }=\left[ \begin{matrix} 1 & 0 & 3 \\ 2 & 1 & -1 \\ 1 & -1 & 1 \end{matrix} \right] \) then, find A.

Find the number of arrangements that can be made out of the letters of the word "ASSASSINATION".

Find the slope of the lines which make an angle of 45° with the line 3x - y + 5 = 0.

Prove that \(\frac { sin(-\theta )tan({ 90 }^{ o }-\theta )sec\left( { 180 }^{ o }-\theta \right) }{ sin(180+\theta )cot(360-\theta )cosec({ 90 }^{ o }-\theta ) } =1\)

Differentiate the following with respect to x \(\frac { { e }^{ x } }{ 1+{ e }^{ x } } \)

Verify Euler’s theorem for the function \(u=\frac{1}{\sqrt{x^2+y^2}}\)

A man bought 6% stock of Rs.12,000 at 92 and sold it when the rise to 96.Find his gain.

Compared to the previous year the overhead expenses went up by 32% in 1995, they increased by 40% in the next year and by 50% in the following year. Calculate the average rate of increase in overhead expenses over the three years.

If two lines of regression are 3X-2Y+1=0, 2X-Y-2=0, find \(\bar {X}\)and \(\bar{Y}\).

Solve the following LPP graphically. Maximize Z = 6x₁ + 5x₂ Subject to the constraints 3x₁ + 5x₂ ≤ 15, 5x₁ + 2x₂ ≤ 10 and x₁,x₂ ≥ 0

1. Solve by matrix inversion method: 3x - y + 2z = 13 ; 2x + Y - z = 3 ; x + 3y - 5z = - 8.

2. By the principle of mathematical induction, prove the following.
   1³ + 2³ + 3³ + ....... + n³ = \(\frac { { n }^{ 2 }(n+1)^{ 2 } }{ 4 } \) for all \(n\in N\).

1. How many numbers greater than a million can be formed with the digits 2, 3, 0, 3, 4, 2, 3?

2. Show that the given lines 3x - 4y - 13 = 0, 8x - 11y = 33 and 2x - 3y - 7 = 0 are concurrent and find the concurrent point.

1. If tan x = \(\frac { -4 }{ 3 } \) and x is in II quadrant,find \(sin\frac { x }{ 2 } ,cos\frac { x }{ 2 } \) and \(tan\frac { x }{ 2 } \)

2. Find the value of tan \(\left( {{\pi}\over{8}}\right).\)

1. if y = 2 sin x + 3 cos x, then show that y₂ + y = 0

2.  The demand for a commodity x is q = 5-2p₁+ P₂ -\({ p }_{ 1 }^{ 2 }{ p }_{ 2 }\). Find the partial elasticities \(\frac { Eq }{ { EP }_{ 1 } } \) and \(\frac { Eq }{ { EP }_{ 2 } } \) when p₁= 3 and p₂ = 7

1. For the total revenue function R = - 90 + 6x² - x³ find when R is increasing and when it is decreasing. Also, discuss the behaviour of marginal revenue.

2. Kamal sold Rs.9000 worth 7% stock at 80 and invested the proceeds in 15% stock at 120. Find the change in his income?

1. Calculate the Mean deviation about median and its relative measure for the following data.

   X	15	25	35	45	55	65	75	85
   frequency	12	11	10	15	22	13	18	19

2. Find the equation of the regression line of Y on X, if the observations ( X_i, Y_i) are the following (1, 4) (2, 8) (3, 2) ( 4, 12) (5, 10) (6, 14) (7, 16) ( 8, 6) (9, 18).

1. A company is producing three products P₁, P₂ and P₃, with profit contribution of Rs.20, Rs.25 and Rs.15 per unit respectively. The resource requirements per unit of each of the products and total availability are given below.

   Product	P1	P2	P3	Total availability
   Man hours/unit	6	3	12	200
   Machine hours/unit	2	5	4	350
   Material/unit	1kg	2kg	1kg	100kg

   Formulate the above as a linear programming model.

2. Every gram of wheat provides 0.1 g of proteins and 0.25 g of carbohydrates. The corresponding values of rice are 0.05 g and 0.5 g respectively. Wheat cost Rs.4 per kg and rice cost Rs.6 per kg. The minimum daily requirements of proteins and carbohydrate for an average child are 50 g and 200 g respectively. In what quantities should wheat and rice be mixed in the daily diet to provide minimum daily requirements of proteins and carbohydrate at minimum cost. Frame an LPP and solve it graphically.