If n((A \(\times\) B) ∩(A \(\times\) C)) = 8 and n(B ∩ C) = 2, then n(A) is

\(n(A\cap B)=4\) and \((A\cup B)=11\) then \(n(p(A\triangle B))\) is __________

The value of \({ log }_{ 3 }\frac { 1 }{ 81 } \) is

The quadratic equation whose roots are tan 75° and cot 75° is _______________

The numerical value of tan^-11 + tan^-12 + tan^-13 = _______________

If 10 lines are drawn in a plane such that no two of them are parallel and no three are concurrent, then the total number of points of intersection are

There is a letter lock with 3 rings each marked with 5 letters and do not know the keyword. The total number of attempts can be made to know the keyword is  _________

The sum up to n terms of the series \(\frac { 1 }{ \sqrt { 1 } +\sqrt { 3 } } +\frac { 1 }{ \sqrt { 3 } +\sqrt { 5 } } +\frac { 1 }{ \sqrt { 5 } +\sqrt { 7 } } +\)....is 

Equation of the straight line that forms an isosceles triangle with coordinate axes in the I-quadrant with perimeter 4 + 2\(\sqrt{2}\) is

The equation of the bisectors of the angle between the co-ordinate axes are ______________

If \(\left\lfloor . \right\rfloor \) denotes the greatest integer less than or equal to the real number under consideration and −1\(\le\) x < 0, 0 \(\le\) y < 1, 1 \(\le\) z < 2, then the value of the determinant \(\begin{vmatrix} \left\lfloor x \right\rfloor +1& \left\lfloor y \right\rfloor & \left\lfloor z \right\rfloor \\ \left\lfloor x \right\rfloor & \left\lfloor y \right\rfloor +1& \left\lfloor z \right\rfloor \\ \left\lfloor x \right\rfloor & \left\lfloor y \right\rfloor & \left\lfloor z \right\rfloor +1\end{vmatrix}\) is

The value of \(\left| \begin{matrix} x+1 & x+2 & x+a \\ x+2 & x+3 & x+b \\ x+3 & x+4 & x+c \end{matrix} \right| \) =_____________ 0, where a, b, c are in AP is 

If \(\overrightarrow{a}, \overrightarrow{b}, \overrightarrow{c}\) are the position vectors of three collinear points, then which of the following is true?

\(lim_{x\rightarrow {\pi/2}}{2x-\pi\over cosx} \)

The points of discontinuity of the function \(\frac { { x }^{ 2 }+6x+8\quad }{ { x }^{ 2 }-5x+6\quad } is\)

\(\text { If } f(x)=\left\{\begin{array}{ll} a x^2-b, & -1<x<1 \\ \frac{1}{|x|}, & \text { elsewhere } \end{array} \ \text { is differentiable at } x=1\right. \text {, then }\) 

If \(\int {3^{1\over x}\over x^2}dx=k(3^{1\over x})+c\), then the value of k is

\(\int { \frac { \left( log{ x } \right) ^{ 3 } }{ x } } \) dx = _________+c.

If A and B are any two events, then the probability that exactly one of them occur is

Three integers are chosen at random from the first 20 integers. The probability that their product is even is

Write the following in roster form.
The set of all positive roots of the equation (x-1)(x+1)(x²-1) = 0.

Determine whether the following functions are even, odd or neither.
sin²x - 2 cos²x - cos x.

If nP₄ = 20 \(\times\) 3 nP₂, then find n.

If a, b, c are in A.P., show that (a-c)² = 4(b² - ac).

Find the combined equation of the straight lines through the origin one of which is parallel to and the other is perpendicular to the straight line 3x + y + 5 = 0.

Find \(\overrightarrow{a}.\overrightarrow{b}\) when \(\overrightarrow{a}\)= \(\hat{i}-\hat{j}+5\hat{k}\)  and \(\overrightarrow{b}=3\hat{i}-2\hat{k}\)

Evaluate \(\lim _{ x\rightarrow 1 }{ \frac { 1+(x-1{ ) }^{ 2 } }{ 1+{ x }^{ 2 } } } \)

Differentiate the following with respect to x : \(y={log x \ x \over e^x}\)

Integrate the following functions with respect to x : \({1\over (2-3x)^4}\)

Two cards are drawn at random and without replacement from a pack of 52 playing cards. Find the probability that both the cards are black.

Prove that the relation "friendship" is not an equivalence relation on the set of all people in Chennai.

Solve the quadratic equation 5^2x- 5^{x + 3}+ 125 = 5^x.

Express each of the following angles in radian measure
30⁰

The Sum of infinite number of terms of G.P is 23 and the sum of their sequence is 69. Find the G.P

If the slope of one of the lines given by ax²+2hxy+by² = 0 is k times the other, prove that 4Kh² = ab (HK)²

Identify the singular and non-singular matrices:\(\begin{bmatrix} 0&a-b &k \\ b-a & 0 &5 \\ -k & -5 & 0 \end{bmatrix}\)

Use the graph to find the limits (if it exists). If the limit does not exist, explain why?
\(lim_{x\rightarrow3}(4-x)\).

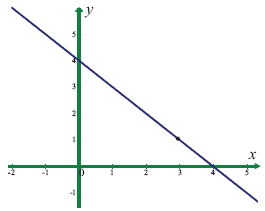
If \({ x }^{ 2 }+2xy+{ y }^{ 3 }=42,\) find \(\frac { dy }{ dx } \)

Integrate the following functions with respect to x : \({sin^2x\over 1+cos \ x}\)

The chances of A, B, and C becoming manager of a certain company are 5 : 3: 2. The probabilities that the office canteen will be improved if A, B, and C become managers are 0.4, 0.5 and 0.3 respectively. If the office canteen has been improved, what is the probability that B was appointed as the manager?

If f, g, h are real valued functions defined on R, then prove that (f + g) o h = f o h + g o h. What can you say about f o(g + h)? Justify your answer.

Solve the equation x³+ 5x²-16x-14 = 0. Given x + 7 is a root

In a \(\triangle \)ABC, if \(\frac { sin \ A }{ sin \ C } =\frac { sin(A-B) }{ sin(B-c) },\), prove that a², b², c²are in arithmetic progression 

Find the sum of all 4-digit numbers that can be formed using the digits 1, 2, 4, 6, 8.

Prove that \(\sqrt [ 3 ]{ x^3+7 } -\sqrt [ 3 ]{ x^3+4 } \) is approximately equal to \({1\over x^2}\) when x is large.

Find the equation of the lines passing through the point of intersection lines 4x - y + 3 = 0 and 5x + 2y + 7 = 0
(i) through the point (-1, 2)
(ii) Parallel to x - y + 5 = 0
(iii) Perpendicular to x - 2y + 1 = 0.

Locus of the mid points of the portion of the line \(x\sin\theta+y\cos\theta=p\) intercepted between the axis is ............

A shopkeeper in a Nuts and Spices shop makes gift packs of cashew nuts, raisins, and almonds.
Pack I contains 100 gm of cashew nuts, 100 gm of raisins and 50 gm of almonds.
Pack-II contains 200 gm of cashew nuts, 100 gm of raisins and 100 gm of almonds.
Pack-III contains 250 gm of cashew nuts, 250 gm of raisins and 150 gm of almonds.
The cost of 50 gm of cashew nuts is Rs.50, 50 gm of raisins is Rs.10, and 50 gm of almonds is Rs.60. What is the cost of each gift pack?

Let \(\overrightarrow{a},\overrightarrow{b},\overrightarrow{c}\)be unit vectors such that\(\overrightarrow{a}.\overrightarrow{b}=\overrightarrow{a}.\overrightarrow{c}=0\) and the angle between \(\overrightarrow{b} \ and \ \overrightarrow{c}\) is  \({\pi\over 3}.\) Prove that \(\overrightarrow{a}=\pm{2\over \sqrt{3}}(\overrightarrow{b}\times \overrightarrow{c}).\)

Evaluate the following limits :\(lim_{x\rightarrow 0}{e^{ax}-e^{bx}\over x}\)

Discuss the differentiability of \(f\left( x \right) =\begin{cases} x{ e }^{ -\left( \frac { 1 }{ \left| x \right| } +\frac { 1 }{ x } \right) } \\ 0,\quad x=0 \end{cases}, x\neq 0\) at x = 0

Integrate the following with respect to x : e^x

Evaluate \(\int { \frac { { x }^{ 3 }dx }{ { x }^{ 4 }+{ 3x }^{ 2 }+2 } } \)

A purse contains 3 silver and 4 copper coins. A second purse contains 4 silver and 3 copper coins. If a coin is pulled out at random from one of the two purses, what is the probability that it is a silver coin?