If ^a2-a \(C_2 = ^{a^2-a}\) C₄ then the value of 'a' is

There are 10 points in a plane and 4 of them are collinear. The number of straight lines joining any two points is

The number of ways in which a host lady invite 8 people for a party of 8 out of 12 people of whom two do not want to attend the party together is

The number of rectangles that a chessboard has

The number of 10 digit number that can be written by using the digits 2 and 3 is

The number of ways to average the letters of the word CHEESE are _________

5c₁ + 5c₂ + 5c₃ + 5c₄ + 5c₅  is equal to _________

The number of diagonals of a decagon _________

If a and b are chosen randomly from the set {1,2,3,4} with replacement, then the probability of the real roots of the equation \(x^2+ax+b=0\) is

In a certain college 4% of the boys and 1% of the girls are taller than 1.8 meter. Further 60% of the students are girls. If a student is selected at random and is taller than 1.8 meters, then the probability that the student is a girl is

How many two-digit numbers can be formed using 1, 2, 3, 4, 5 without repetition of digits?

Count the number of three-digit numbers which can be formed from the digits 2, 4, 6, 8 if
(i) repetitions of digits is allowed.
(ii) repetitions of digits is not allowed

How many three-digit odd numbers can be formed using the digits 0, 1, 2, 3, 4, 5? if 
The repetition of digits is allowed

By the principle of mathematical induction, prove that for n > 1
\(1^3 +2^3 +3^3 + .. +n^3=\left[n(n+1)\over 2\right]^2\)

Using the mathematical induction, show that for any natural number n > 2,
\(\left(1-{1\over 2^2} \right)\left(1-{1\over 3^2} \right)\left(1-{1\over 4^2} \right)...\left(1-{1\over n^2} \right)={n+1\over 2n}\)

Use induction to prove that 10ⁿ + 3 \(\times\) 4ⁿ⁺² + 5 is divisible by 9 for all natural numbers n

How many paths are there from start to end on a 6\(\times\) 4 grid as shown in the picture?

If ⁵P_r = ⁷P_r-1 find r.

If an experiment has exactly the three possible mutually exclusive outcomes A, B, and C, check in each case whether the assignment of probability is permissible.
\(P(A)=\frac { 1 }{ \sqrt { 3 } } ,\quad P(B)-1-\frac { 1 }{ \sqrt { 3 } } ,\quad P(C)-0\)

A factory has two Machines-I and II. Machine-I produces 60% of items and Machine-II produces 40% of the items of the total output. Further 2% of the items produced by Machine-I are defective whereas 4% produced by Machine-II are defective. If an item is drawn at random what is the probability that it is defective?

A student appears in an objective test which contain 5 multiple choice questions. Each question has four choices out of which one correct answer.
(i) What is the maximum number of different answers can the students give?
(ii) How will the answer change if each question may have more than one correct answers?

How many strings can be formed from the letters of the word ARTICLE, so that vowels occupy the even Places?

Find the number of strings that can be made using all letters of the word THING. If these words are written as in a dictionary, what will be the 85^th string?

(i) Find the number of strings of length 4, which can be formed using the letters of the word BIRD, without repetition of the letters.
(ii) How many strings of length 5 can be formed out of the letters of the word PRIME taking all the letters at a time without repetition.

Prove that the sum of first n positive odd numbers is n².

The chances of A, B, and C becoming manager of a certain company are 5 : 3: 2. The probabilities that the office canteen will be improved if A, B, and C become managers are 0.4, 0.5 and 0.3 respectively. If the office canteen has been improved, what is the probability that B was appointed as the manager?

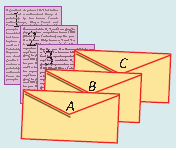
Three letters are written to three different persons and addresses on three envelopes are also written. Without looking at the addresses, what is the probability that (i) exactly one letter goes to the right envelopes (ii) none of the letters go into the right envelopes?