The critical path of the following network is________.

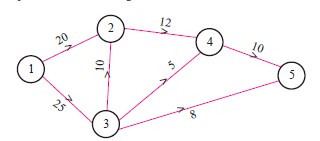A solution which maximizes or minimizes the given LPP is called ______.

The maximum value of the objective function Z = 3x + 5y subject to the constraints x > 0 , y > 0 and 2x + 5y ≤ 10 is ______.

Which of the following is not correct?

Network problems have advantage in terms of project _______.

Given an L.P.P maximize Z = 2x₁ + 3x₂ subject to the constrains x₁ + x₂ ≤ 1, 5x₁ + 5x₂ ≥ 0 and x₁ ≥ 0, x₂ ≥ 0 using graphical method, we observe ______.

Draw the logic network for the following:
Activities C and D both follow A, activity E follows C, activity F follows D, activity E and F precedes B.

Draw a network diagram for the project whose activities and their predecessor relationships are given below:

Construct a network diagram for the following situation:
A < D, E; B, D < F; C < G and B < H.

Draw the network for the project whose activities with their relationships are given below:
Activities A, D, E can start simultaneously; B, C > A; G, F > D, C; H > E, F.

Construct the network for the projects consisting of various activities and their precedence relationships are as given below:
A, B, C can start simultaneously A < F, E; B < D, C; E, D < G

An aeroplane can carry a maximum of 200 passengers. A profit of Rs.1000 is made on each executive class ticket and a profit of Rs. 600 is made on each economy class ticket. The airline reserves at least 20 seats for executive class. However, at least 4 times as many passengers prefer to travel by economy class than by the executive class. Determine how many tickets of each type must be sold in order to maximize the profit for the airline. Formulate the mathematical LPP for the above.

A producer has 30 and 17 units of labour and capital respectively which he can use to produce two types of goods X and Y. To produce one unit of X, 2 unit of labour and 3 units of capital are required. Similarly, 3 units of labour and 1 unit of capital is required to produce one unit of Y. If X and Yare priced at HOO and H20 per unit respectively, how should the producer use his resources to maximize the total revenue? Formulate the LPP for the above.

A dealer whises to purchase a number of fans and sewing machines. He has only Rs.5760 to invest and has a space for atmost 20 items. A fan costs him Rs.360 and a sewing machine Rs. 240. His expectation is he can sell a fan at a profit of Rs.22  and a sewing machine at a profit of ns. Formulate this as an LPP to maximize his profit?

Draw a network diagram for the project whose activities and their predecessor relationships are given below

Construct the network for the projects consisting of various activities and their precedence relationships are as given below: A, B can start simultaneously
 A < D, E; B < F; E < G, D < C, F < H.

Maximize Z = 3x₁ + 4x₂ subject to x₁ – x₂ ≤ –1; –x₁ + x₂ ≤ 0 and x₁, x₂ ≥ 0

Solve the following LPP graphically. ∴ Maximize Z = 3x₁ + 4x₂  subject to the constraints x₁ + x₂ ≤ 4 and x₁,x₂ ≥ 0.

Solve the following LPP graphically. Maximize \(Z={ x }_{ 1 }+{ x }_{ 2 }\)
Subject to the constraints \({ x }_{ 1 }-{ x }_{ 2 }\le -1,{ -x }_{ 1 }+{ x }_{ 2 }\le 0\quad and\quad { x }_{ 1 }+{ x }_{ 2 }\ge 0\)

Solve the following LPP. Maximize Z = 2 x₁ + 5x₂ subject to the conditions x₁+ 4x₂ ≤ 24. 3x₁+x₂ ≤ 21, x₁+x₂ ≤ 9 and x₁, x₂ ≥ 0.

Solve the following linear programming problems by graphical method.
(i) Maximize Z = 6x₁ + 8x₂ subject to constraints 30x₁+20x₂≤300; 5x₁+10x₂≤110; and x₁, x₂ ≥ 0 .
(ii) Maximize Z = 22x₁+ 18x₂  subject to constraints 960x₁+ 640x₂≤15360; x₁≥ x≤20 and x₁, x₂ ≥ 0.
(iii) Minimize Z = 3x₁+ 2x₁ subject to the constraints 5x₁+ x₂≥10; x₁+x₂≥6; x₁+ 4, x₂ ≥12 and x₁, x₂ ≥ 0.
(iv) Maximize Z = 40x₁+ 50x₂ = subject to constraints 30x₁+x₂≤9; x₁+2x₂≤8 and x₁, x₂ ≥ 0
(v) Maximize Z = 20x₁ + 30x₂ subject to constraints 3x₁+3x₂≤36; 5x₁+2x₂≤50; 2x₁+6x₂≤60 and x₁,x₂ ≥ 0
(vi) Minimize Z = 20x ₁+ 40x₂ subject to the constraints 36x₁+ 6x₂≥108, 3x₁+12x₂≥36, 20x₁+10x₂≥100 and x₁,x₂ ≥ 0.

Draw the network and calculate the earliest start time, earliest finish time, latest start time and latest finish time of each activity and determine the Critical path of the project and duration to complete the project.