The value of \(\begin{vmatrix} 2x+y & x & y \\ 2y+z & y & z \\ 2z+x & z & x \end{vmatrix}\) is ________.

(a)

xyz

(b)

x+y+z

(c)

2x+2y+2z

(d)

0

If \(\triangle=\begin{vmatrix} 1 & 2 & 3 \\ 3 & 1 & 2 \\ 2 & 3 & 1 \end{vmatrix}\) then \(\begin{vmatrix} 3 & 1 & 2 \\ 1 & 2 & 3 \\ 2 & 3 & 1 \end{vmatrix}\) is ________.

(a)

\(\triangle\)

(b)

-\(\triangle\)

(c)

3\(\triangle\)

(d)

-3\(\triangle\)

The value of the determinant \({\begin{vmatrix} a & 0 & 0 \\ 0 & a & 0 \\ 0 & 0 & c \end{vmatrix}}^{2}\)is ________.

(a)

abc

(b)

0

(c)

a²b²c²

(d)

-abc

adj (AB) is equal to ________.

(a)

adj A adj B

(b)

adj A^T adj B^T

(c)

adj B adj A

(d)

adj B^T adj A^T

The inverse matrix of \(\begin{pmatrix} \frac { 1 }{ 5 } & \frac { 5 }{ 25 } \\ \frac { 2 }{ 5 } & \frac { 1 }{ 2 } \end{pmatrix}\) is ________.

(a)

\({{7}\over{30}}\begin{pmatrix} \frac { 1 }{ 2 } & \frac { 5 }{ 12 } \\ \frac { 2 }{ 5 } & \frac { 4 }{ 5 } \end{pmatrix}\)

(b)

\({{7}\over{30}}\begin{pmatrix} \frac { 1 }{ 2 } & \frac { -5 }{ 12 } \\ \frac { -2 }{ 5 } & \frac { 1 }{ 5 } \end{pmatrix}\)

(c)

\({{30}\over{7}}\begin{pmatrix} \frac { 1 }{ 2 } & \frac { 5 }{ 12 } \\ \frac { 2 }{ 5 } & \frac { 4 }{ 5 } \end{pmatrix}\)

(d)

\({{30}\over{7}}\begin{pmatrix} \frac { 1 }{ 2 } & \frac { -5 }{ 12 } \\ \frac { -2 }{ 5 } & \frac { 4 }{ 5 } \end{pmatrix}\)

The number of Hawkins-Simon conditions for the viability of an input - output analysis is ________.

(a)

1

(b)

3

(c)

4

(d)

2

The inventor of input-output analysis is ________.

(a)

Sir Francis Galton

(b)

Fisher

(c)

Prof. Wassily W. Leontief

(d)

Arthur Caylay

Which of the following matrix has no inverse.

(a)

\(\begin{pmatrix} -1 & 1 \\ 1 &-4 \end{pmatrix}\)

(b)

\(\begin{pmatrix} 2 & -1 \\ -4 &2 \end{pmatrix}\)

(c)

\(\begin{pmatrix} cos\ a & sin\ a \\ -sin\ a & cos\ a \end{pmatrix}\)

(d)

\(\begin{pmatrix} sin\ a & cos\ a \\ -cos\ a & sin\ a \end{pmatrix}\)

If A \(=\begin{pmatrix} -1 & 2 \\ 1 & -4 \end{pmatrix}\) then A (adj A) is ________.

(a)

\(\begin{pmatrix} -4 & -2 \\ -1 & -1 \end{pmatrix}\)

(b)

\(\begin{pmatrix} 4 & -2 \\ -1 & 1 \end{pmatrix}\)

(c)

\(\begin{pmatrix} 2 & 0 \\ 0 & 2 \end{pmatrix}\)

(d)

\(\begin{pmatrix} 0 & 2 \\ 2 & 0 \end{pmatrix}\)

If A is an invertible matrix of order 2, then det (A^-1) be equal to ________.

(a)

det (A)

(b)

\({{1}\over{det(A)}}\)

(c)

1

(d)

0

If A is 3 \(\times\) 3 matrix and |A| = 4, then |A^-1| is equal to ________.

(a)

\({{1}\over{4}}\)

(b)

\({{1}\over{16}}\)

(c)

2

(d)

4

If A is a square matrix of order 3 and IAI = 3 then | adj A| is equal to ________.

(a)

81

(b)

27

(c)

3

(d)

9

If A = \(\begin{vmatrix}cos \theta & sin \theta \\ -sin \theta&cons\theta \end{vmatrix},\) then |2A| is equal to ________.

(a)

4 cos 2 \(\theta\)

(b)

4

(c)

2

(d)

1

If \(\triangle=\begin{vmatrix} {a}_{11} & {a}_{12} & {a}_{13} \\ {a}_{21} & {a}_{22} & {a}_{23} \\ {a}_{31} & {a}_{32} & {a}_{33} \end{vmatrix}\) and A_ij is cofactor of a_ij, then value of \(\triangle\) is given by ________.

(a)

a₁₁ A₃₁ + a₁₂ A₃₂ + a₁₃ A₃₃

(b)

a₁₁ A₁₁ + a₁₂ A₂₁ + a₁₃ A₃₁

(c)

a₂₁ A₁₁ + a₂₂ A₁₂ + a₂₃ A₁₃

(d)

a₁₁ A₁₁ + a₂₁ A₂₁ + a₃₁ A₃₁

If \(\begin{vmatrix} 4 & 3 \\ 3 & 1 \end{vmatrix}=-5\) then value of \(\begin{vmatrix} 20 & 15 \\ 15 & 5 \end{vmatrix}\) is ________.

(a)

-5

(b)

-125

(c)

-25

(d)

0

If nC₃ = nC₂, then the value of nC₄ is _______.

(a)

2

(b)

3

(c)

4

(d)

5

The number of ways selecting 4 players out of 5 is _______.

(a)

4!

(b)

20

(c)

25

(d)

5

The greatest positive integer which divide n(n + 1) (n + 2) (n + 3) for n \(\in\) N is ________.

(a)

2

(b)

6

(c)

20

(d)

24

For all n > 0, nC₁ + nC₂ + nC₃ + ... +nC_n is equal to _______

(a)

2n

(b)

2ⁿ- 1

(c)

n²

(d)

n² - 1

The constant term in the expansion of \({ \left( x+\frac { 2 }{ x } \right) }^{ 6 }\) is _______

(a)

156

(b)

165

(c)

162

(d)

160

If \(\frac { kx }{ (x+4)(2x-1) } =\frac { 4 }{ x+4 } +\frac { 1 }{ 2x-1 } \) then k is equal to _______.

(a)

9

(b)

11

(c)

5

(d)

7

The number of parallelograms that can be formed from a set of four parallel lines intersecting another set of three parallel lines is _________.

(a)

18

(b)

12

(c)

9

(d)

6

The total number of 9 digit number which have all different digit is ________.

(a)

10!

(b)

9!

(c)

9\(\times\)9!

(d)

10\(\times\)10!

Number of words with or without meaning that can be formed using letters of the word "EQUATION" , with no repetition of letters is _____.

(a)

7!

(b)

3!

(c)

8!

(d)

5!

The number of permutation of n different things taken r at a time, when the repetition is allowed is ________.

(a)

rⁿ

(b)

n^r

(c)

\(\frac { n! }{ (n-r)! } \)

(d)

\(\frac { n! }{ (n+r)! } \)

The angle between the pair of straight lines x² - 7xy + 4y² = 0 _______.

(a)

\(tan^{-1}\left(1\over 3\right)\)

(b)

\(tan^{-1}\left(1\over 2\right)\)

(c)

\(tan^{-1}\left(\sqrt{33}\over 5\right)\)

(d)

\(tan^{-1}\left(5\over\sqrt{33}\right)\)

If the lines 2x - 3y - 5 = 0 and 3x - 4y - 7 = 0 are the diameters of a circle, then its centre is _______.

(a)

(-1, 1)

(b)

(1,1)

(c)

(1, -1 )

(d)

(-1, -1)

The locus of the point P which moves such that P is at equidistance from their coordinate axes is _______.

(a)

\(y={1\over x}\)

(b)

y = -x

(c)

y = x

(d)

\(y=-{1\over x}\)

If kx² + 3xy - 2y² = 0 represent a pair of lines which are perpendicular then k is equal to _______.

(a)

1/2

(b)

-1/2

(c)

2

(d)

-2

The focus of the parabola x² = 16y is _______.

(a)

(4,0)

(b)

(-4,0)

(c)

(0,4)

(d)

(0,-4)

The centre of the circle x² + y - 2x + 2y - 9 = 0 is _______.

(a)

(1,1)

(b)

(-1,-1)

(c)

(-1,1)

(d)

(1, -1)

If the centre of the circle is (-a, -b) and radius is \(\sqrt{a^2-b^2}\), then the equation of circle is _______.

(a)

x²+y²+2ax+2by+2b² = 0

(b)

x²+y²+2ax +2by-2b² = 0

(c)

x² +y² - 2ax - 2by - 2b² = 0

(d)

x² + y - 2ax - 2by + 2b² = 0

ax² + 4xy + 2y² = 0 represents a pair of parallel lines then 'a' is _______.

(a)

2

(b)

-2

(c)

4

(d)

-4

The equation of the circle with centre (3,-4) and touches the x - axis is _______.

(a)

(x - 3)² +(y - 4)² = 4

(b)

(x - 3)² +(y + 4)² = 16

(c)

(x-3)² + (y- 4)² = 16

(d)

x²+y² = 16

The eccentricity of the parabola is _______.

(a)

3

(b)

2

(c)

0

(d)

1

The distance between directrix and focus of a parabola y² = 4ax is _______.

(a)

a

(b)

2a

(c)

4a

(d)

3a

The degree measure of \(\frac{\pi}{8}\) is ______.

(a)

20^o60^'

(b)

22^o30^'

(c)

20^o60^'

(d)

20^o30^'

If \(\tan\theta=\frac{1}{\sqrt5}\) and \(\theta\) lies in the first quadrant, then \(\cos\theta\) is _______.

(a)

\(\frac{1}{\sqrt6}\)

(b)

\(\frac{-1}{\sqrt6}\)

(c)

\(\frac{\sqrt5}{\sqrt6}\)

(d)

\(\frac{-\sqrt5}{\sqrt6}\)

The value of \(\sin(-420^o)\) is _______.

(a)

\(\frac{\sqrt3}{2}\)

(b)

\(-\frac{\sqrt3}{2}\)

(c)

\(\frac{1}{2}\)

(d)

\(\frac{-1}{2}\)

The value of sin 15^o cos 15^o is ______.

(a)

1

(b)

\(\frac{1}{2}\)

(c)

\(\frac{\sqrt3}{2}\)

(d)

\(\frac{1}{4}\)

The value of cos²45^o - sin²45^o is_______.

(a)

\(\frac{\sqrt3}{2}\)

(b)

\(\frac{1}{2}\)

(c)

0

(d)

\(\frac{1}{\sqrt{2}}\)

The value of 1 - 2sin²45^o is _______.

(a)

1

(b)

\(\frac{1}{2}\)

(c)

\(\frac14\)

(d)

0

The value of \(\frac{2\tan30^o}{1+tan^230}\) is _____.

(a)

\(\frac12\)

(b)

\(\frac{1}{\sqrt3}\)

(c)

\(\frac{\sqrt{3}}{2}\)

(d)

\(\sqrt3\)

The value of \(\frac{3 \tan 10^{\circ}-\tan ^3 10^{\circ}}{1-3 \tan ^2 10^{\circ}}\) is _______,

(a)

\(\frac{1}{\sqrt3}\)

(b)

\(\frac{1}{2}\)

(c)

\(\frac{\sqrt3}2\)

(d)

\(\frac{1}{\sqrt2}\)

If \(\alpha\) and \(\beta\) be between 0 and \(\frac{\pi}{2}\) and if \(\cos(\alpha+\beta)=\frac{12}{13}\) and \(\sin(\alpha-\beta)=\frac{3}{5}\) then \(\sin2\alpha\) is  _____.

(a)

\(\frac{16}{15}\)

(b)

0

(c)

\(\frac{56}{65}\)

(d)

\(\frac{64}{65}\)

\(\tan\left(\frac{\pi}{4}-x\right)\) is _______.

(a)

\(\left(\frac{1+\tan x}{1-\tan x}\right)\)

(b)

\(\left(\frac{1-\tan x}{1+\tan x}\right)\)

(c)

1-tan x

(d)

1+tan x

\(\sin\left(\cos^{-1}\frac{3}{5}\right)\) is _____.

(a)

\(\frac{3}{5}\)

(b)

\(\frac{5}{3}\)

(c)

\(\frac{4}{5}\)

(d)

\(\frac{5}{4}\)

\(\left(\frac{\cos x}{cosec x}\right)-\sqrt{1-\sin^2x}\sqrt{1-\cos^2x}\) is _______.

(a)

cos²x-sin²x

(b)

sin²x-cos²x

(c)

1

(d)

0

If f(x) = x² - x + 1, then f (x + 1) is _______.

(a)

x²

(b)

x

(c)

1

(d)

x² + x + 1

If f(x) = \(\frac{1-x}{1+x}\) then f(-x) is equal to _______.

(a)

-f(x)

(b)

\(\frac{1}{f(x)}\)

(c)

\(-\frac{1}{f(x)}\)

(d)

f(x)

The graph of y = 2x² is passing through _______.

(a)

(0,0)

(b)

(2,1)

(c)

(2,0)

(d)

(0,2)

If f(x) = 2^x and get g(x) = \(\frac{1}{2^x}\) then (fg)(x) is ________.

(a)

1

(b)

0

(c)

4^x

(d)

\(\frac{1}{4^x}\)

The range of f(x) = |x|, for all \(x\in R\), is ________.

(a)

(0, \(\infty \))

(b)

(0, \(\infty \))

(c)

(-\(\infty \), \(\infty \))

(d)

(1, \(\infty \))

\(\lim _{ x\rightarrow \infty }{ \frac { \tan { \theta } }{ \theta } } =\)________.

(a)

1

(b)

\(\infty\)

(c)

\(-\infty\)

(d)

\(\theta\)

For what value of x, f(x) = \(\frac{x+2}{x-1}\) is not continuous?

(a)

-2

(b)

1

(c)

2

(d)

-1

\(\frac{d}{dx}(\frac{1}{x})\) is equal to ________.

(a)

\(-\frac{1}{x^2}\)

(b)

\(-\frac{1}{x}\)

(c)

log x

(d)

\(\frac{1}{x^2}\)

If y = x and z = \(\frac{1}{x}\) then \(\frac{dy}{dz}=\)________.

(a)

x²

(b)

1

(c)

-x²

(d)

\(-\frac{1}{x^2}\)

\(\frac{d}{dx}(a^x)=\) ________.

(a)

\(\frac { 1 }{ x\log e^a}\)

(b)

a^a

(c)

x log_e^a

(d)

a^x log_e^a

Average fixed cost of the cost function C(x) = 2x³ +5x² - 14x +21 is _______.

(a)

\(\frac { 2 }{ 3 } \)

(b)

\(\frac { 5}{ x } \)

(c)

\(\frac { 14 }{ x } \)

(d)

\(\frac { 21 }{ x } \)

Marginal revenue of the demand function p = 20–3x is _______.

(a)

20–6x

(b)

20–3x

(c)

20+6x

(d)

20+3x

If the demand function is said to be inelastic, then _______.

(a)

|η_d| > 1

(b)

|η_d| = 1

(c)

|η_d| < 1

(d)

|η_d| = 0

The elasticity of demand for the demand function x = \(\frac { 1 }{ p } \) is______.

(a)

0

(b)

1

(c)

\(-\frac { 1 }{ p } \)

(d)

\(\infty \)

Relationship among MR, AR and η_d is ______.

(a)

\({ n }_{ d }=\frac { AR }{ AR-MR } \)

(b)

η_d =  AR - MR

(c)

MR = AR = η_d

(d)

\(AR=\frac { MR }{ {ηd } } \)

Instantaneous rate of change of y = 2x² + 5x with respect to x at x = 2 is _________.

(a)

4

(b)

5

(c)

13

(d)

9

Profit P(x) is maximum when ________.

(a)

MR = MC

(b)

MR = 0

(c)

MC = AC

(d)

TR = AC

The maximum value of f(x) = sinx is ________.

(a)

1

(b)

\(\frac { \sqrt { 3 } }{ 2 } \)

(c)

\(\frac { 1 }{ \sqrt { 2 } } \)

(d)

\(-\frac { 1 }{ \sqrt { 2 } } \)

If f(x,y) is a homogeneous function of degree n, then \(x\frac { \partial f }{ \partial x } +y\frac { \partial f }{ \partial y } \) is equal to ________.

(a)

(n–1)f

(b)

n(n–1)f

(c)

nf

(d)

f

If u = \({ e }^{ { x }^{ 2 } }\) then \(\frac { \partial u }{ \partial x } \) is equal to _______.

(a)

\({ 2x }e^{ { x }^{ 2 } }\)

(b)

\({ e }^{ { x }^{ 2 } }\)

(c)

\({2 e }^{ { x }^{ 2 } }\)

(d)

0

A company begins to earn profit at _______.

(a)

Maximum point

(b)

Breakeven point

(c)

Stationary point

(d)

Even point

The demand function is always _______.

(a)

Increasing function

(b)

Decreasing function

(c)

Non-decreasing function

(d)

Undefined function

if q = 1000 + 8p₁ - p₂ then, \(\frac { \partial q }{ \partial { p }_{ 1 } } \) is _______.

(a)

-1

(b)

8

(c)

1000

(d)

1000 - p₂

If R = 5000 units / year, C₁ = 20 paise , C₃ = Rs. 20 then EOQ is _______.

(a)

5000

(b)

100

(c)

1000

(d)

200

The dividend received on 200 shares of face value Rs.100 at 8% is ________.

(a)

Rs. 1600

(b)

Rs. 1000

(c)

Rs. 1500

(d)

Rs. 800

If a man received a total dividend of Rs. 25,000 at 10% dividend rate on a stock of face value Rs.100, then the number of shares purchased.

(a)

3500

(b)

4500

(c)

2500

(d)

300

The brokerage paid by a person on the sale of 400 shares of face value Rs.100 at 1% brokerage ________.

(a)

Rs. 600

(b)

Rs. 500

(c)

Rs. 200

(d)

Rs. 400

A person brought 100 shares of 9% stock of face value Rs. 100, at a discount of 10%, then the stock purchased is _______.

(a)

Rs. 9000

(b)

Rs. 6000

(c)

Rs. 5000

(d)

Rs. 4000

The annual income on 500 shares of face value Rs.100 at 15% is _______.

(a)

Rs. 7,500

(b)

Rs. 5,000

(c)

Rs. 8,000

(d)

Rs. 8,500

If ‘a’ is the annual payment, ‘n’ is the number of periods and ‘i’ is compound interest for Rs. 1 then future amount of the annuity is  _______.

(a)

A = \(\frac{a}{i}(1+i)(1+i)^n-1]\)

(b)

A = \(\frac{a}{i}[(1+i)^n-1]\)

(c)

P = \(\frac{a}{i}\)

(d)

P = \(\frac{a}{i}(1+i)[1-(1+i)^{-n}]\)

An annuity in which payments are made at the beginning of each payment period is called _______.

(a)

Annuity due

(b)

An immediate annuity

(c)

perpetual annuity

(d)

none of these

The present value of the perpetual annuity of Rs. 2000 paid monthly at 10 % compound interest is _______.

(a)

Rs. 2,40,000

(b)

Rs. 6,00,000

(c)

Rs. 20,40,000

(d)

Rs. 2,00,400

Which of the following is positional measure?

(a)

Range

(b)

Mode

(c)

Mean deviation

(d)

Percentiles

When calculating the average growth of economy, the correct mean to use is?

(a)

Weighted mean

(b)

Arithmetic mean

(c)

Geometric mean

(d)

Harmonic mean

The best measure of central tendency is _________.

(a)

Arithmetic mean

(b)

Harmonic mean

(c)

Geometric mean

(d)

Median

The harmonic mean of the numbers 2,3,4 is _________.

(a)

\(\frac { 12 }{ 13 } \)

(b)

2

(c)

\(\frac { 36 }{ 13 } \)

(d)

\(\frac { 13 }{ 36 } \)

The geometric mean of two numbers 8 and 18 shall be _________.

(a)

12

(b)

13

(c)

15

(d)

11.08

The correct relationship among A.M.,G.M.and H.M.is: ________.

(a)

A.M.

(b)

G.M.≥A.M.≥H.M.

(c)

H.M.≥G.M.≥A.M.

(d)

A.M.≥G.M.≥H.M.

The median of 10,14,11,9,8,12,6 is _________.

(a)

10

(b)

12

(c)

14

(d)

9

If the mean of 1,2,3,.......n is\(\frac { 6n }{ 11 } \), then the value of n is _________.

(a)

10

(b)

12

(c)

11

(d)

13

The first quartile is also known as _________.

(a)

median

(b)

lower quartile

(c)

mode

(d)

third decile

If Q₁ = 30 and Q₃ = 50, the coefficient of quartile deviation is _________.

(a)

20

(b)

40

(c)

10

(d)

0.25

The two events A and B are mutually exclusive if _________.

(a)

\(P\left( A\cap B \right) =0\)

(b)

\(P\left( A\cap B \right) =1\)

(c)

\(P\left( A\cup B \right) =0\)

(d)

\(P\left( AUB \right) =1\)

If two events A and B are dependent then the conditional probability of P(B/A) is _________.

(a)

\(P(A)P(B/A)\)

(b)

\(\frac { P(A\cap B) }{ P(B) } \)

(c)

\(\frac { P(A\cap B) }{ P(A) } \)

(d)

\(P(A)P(A/B)\)

If the outcome of one event does not influence another event then the two events are _________.

(a)

Mutually exclusive

(b)

Dependent

(c)

Not disjoint

(d)

Independent

The probability of obtaining an even prime number on each die, when a pair of dice is rolled is _________.

(a)

1/36

(b)

0

(c)

1/3

(d)

1/6

Probability that at least one of the events A, B occur is _________.

(a)

\(P(A\cup B)\)

(b)

\(P(A\cap B)\)

(c)

P(A/B)

(d)

\((A\cup B)\)

Example for positive correlation is______.

(a)

Income and expenditure

(b)

Price and demand

(c)

Repayment period and EMI

(d)

Weight and Income

If the values of two variables move in opposite direction then the correlation is said to be ______.

(a)

Negative

(b)

Positive

(c)

Perfect positive

(d)

No correlation

If r(X,Y) = 0 the variables X and Y are said to be ______.

(a)

Positive correlation

(b)

Negative correlation

(c)

No correlation

(d)

Perfect positive correlation

The correlation coefficient from the following data N = 25, ΣX = 125, ΣY = 100, ΣX² = 650, ΣY² = 436, ΣXY = 520 ________.

(a)

0.667

(b)

-0.006

(c)

-0.667

(d)

0.70

The correlation coefficient is ________.

(a)

r(X, Y) = \(\frac { { \sigma }_{ x }{ \sigma }_{ y } }{ cov(x,y) } \)

(b)

r(X, Y) = \(\frac { cov(x,y) }{ { \sigma }_{ x }{ \sigma }_{ y } } \)

(c)

r(X, Y) = \(\frac { cov(x,y) }{ { \sigma }_{ y } } \)

(d)

r(X, Y) = \(\frac { cov(x,y) }{ { \sigma }_{ x } } \)

The variable whose value is influenced (or) is to be predicted is called ________.

(a)

dependent variable

(b)

independent variable

(c)

regressor

(d)

explanatory variable

The correlation coefficient ________.

(a)

r = ±\(\sqrt { { b }_{ xy }\times { b }_{ yx } } \)

(b)

r = \(\frac { 1 }{ { b }_{ xy }\times { b }_{ yx } } \)

(c)

r = b_xy x b_yx

(d)

r = ±\(\sqrt { \frac { 1 }{ { b }_{ xy }\times { b }_{ yx } } } \)

The regression coefficient of X on Y ________.

(a)

b_xy = \(\frac { N\Sigma dxdy-(\Sigma dx)(\Sigma dy) }{ N\Sigma dy^{ 2 }-(\Sigma dy)^{ 2 } } \)

(b)

b_yx = \(\frac { N\Sigma dxdy-(\Sigma dx)(\Sigma dy) }{ N\Sigma dy^{ 2 }-(\Sigma dy)^{ 2 } } \)

(c)

b_xy = \(\frac { N\Sigma dxdy-(\Sigma dx)(\Sigma dy) }{ N\Sigma dx^{ 2 }-(\Sigma dx)^{ 2 } } \)

(d)

b_y =\(\frac { N\Sigma xy-(\Sigma x)(\Sigma y) }{ \sqrt { N\Sigma { x }^{ 2 }-(\Sigma x)^{ 2 }\times \sqrt { N\Sigma y^{ 2 }-(\Sigma y)^{ 2 } } } } \)

When one regression coefficient is negative, the other would be ________.

(a)

Negative

(b)

Positive

(c)

Zero

(d)

None of them

If X and Y are two variates, there can be atmost ________.

(a)

One regression line

(b)

two regression lines

(c)

three regression lines

(d)

more regression lines

Scatter diagram of the variate values (X,Y) give the idea about ________.

(a)

functional relationship

(b)

regression model

(c)

distribution of errors

(d)

no relation

If two variables moves in decreasing direction then the correlation is ________.

(a)

positive

(b)

negative

(c)

perfect negative

(d)

no correlation

The person suggested a mathematical method for measuring the magnitude of linear relationship between two variables say X and Y is ________.

(a)

Karl Pearson

(b)

Spearman

(c)

Croxton and Cowden

(d)

Ya Lun Chou

The term regression was introduced by ________.

(a)

R.A Fisher

(b)

Sir Francis Galton

(c)

Karl Pearson

(d)

Croxton and Cowden

The coefficient of correlation describes ________.

(a)

the magnitude and direction

(b)

only magnitude

(c)

only direction

(d)

no magnitude and no direction

If Cov(x, y) = –16.5, \({ \sigma }_{ x }^{ 2 }=2.89,{ \sigma }_{ y }^{ 2 }\) = 100. Find correlation coefficient ________.

(a)

-0.12

(b)

0.001

(c)

-1

(d)

-0.97

The critical path of the following network is________.

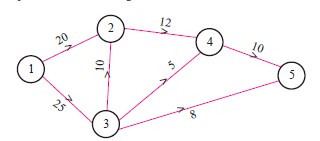(a)

1 – 2 – 4 – 5

(b)

1 – 3 – 5

(c)

1 – 2 – 3 – 5

(d)

1 – 2 – 3 – 4 – 5

Maximize: z = 3x₁ + 4x₂ subject to 2x₁ + x₂ ≤ 40, 2x₁+ 5x₂ ≤ 180, x₁, x₂ ≥ 0. In the LPP, which one of the following is feasible corner point?

(a)

x₁ = 18, x₂ = 24

(b)

x₁ = 15, x₂ = 30

(c)

x₁ = 2.5, x₂ = 35

(d)

x₁ = 20.5, x₂ = 19

A solution which maximizes or minimizes the given LPP is called ______.

(a)

a solution

(b)

a feasible solution

(c)

an optimal solution

(d)

none of these

The maximum value of the objective function Z = 3x + 5y subject to the constraints x > 0 , y > 0 and 2x + 5y ≤ 10 is ______.

(a)

6

(b)

15

(c)

25

(d)

31

In the context of network, which of the following is not correct?

(a)

A network is a graphical representation

(b)

A project network cannot have multiple initial and final nodes

(c)

An arrow diagram is essentially a closed network

(d)

An arrow representing an activity may not have a length and shape

The objective of network analysis is to ________.

(a)

Minimize total project cost

(b)

Minimize total project duration

(c)

Minimize production delays, interruption and conflicts

(d)

All the above

Network problems have advantage in terms of project _______.

(a)

Scheduling

(b)

Planning

(c)

Controlling

(d)

All the above

In critical path analysis, the word CPM mean ______.

(a)

Critical path method

(b)

Crash project management

(c)

Critical project management

(d)

Critical path management

Given an L.P.P maximize Z = 2x₁ + 3x₂ subject to the constrains x₁ + x₂ ≤ 1, 5x₁ + 5x₂ ≥ 0 and x₁ ≥ 0, x₂ ≥ 0 using graphical method, we observe ______.

(a)

No feasible solution

(b)

unique optimum solution

(c)

multiple optimum solution

(d)

none of these