If n((A \(\times\) B) ∩(A \(\times\) C)) = 8 and n(B ∩ C) = 2, then n(A) is

(a)

6

(b)

4

(c)

8

(d)

16

If A = {x / x is an integer, x² \(\le\) 4} then elements of A are ___________

(a)

A = {-1, 0, 1}

(b)

A = {-1, 0, 1, 2}

(c)

A = {0, 2, 4}

(d)

A = {- 2, - 1, 0, 1, 2}

If 8 and 2 are the roots of x²+ ax + c = 0 and 3, 3 are the roots of x² + dx + b = 0; then the roots of the equation x²+ ax + b = 0 are

(a)

1, 2

(b)

-1, 1

(c)

9, 1

(d)

-1, 2

If \(\pi <2\theta <\frac { 3\pi }{ 2 } \), then \(\sqrt { 2+\sqrt { 2+2cos4\theta } } \) equals to

(a)

-2 cosፀ

(b)

-2 sinፀ

(c)

2 cosፀ

(d)

2 sinፀ

2 sin 5x cos x _______________

(a)

sin 6x + cos 4x

(b)

sin 6x + sin 4x

(c)

cos 6x + sin 4x

(d)

cos 6x + cos 4x

The number of permutations of n different things taking r at a time when 3 particular things are to be included is _________

(a)

n-3 P_r-3

(b)

n-3 P_r

(c)

nP_r-3

(d)

r! n-3C_r-3

If ⁿP_r = 720, ⁿC_r = 120 then r is _________

(a)

2

(b)

4

(c)

3

(d)

5

AM, GM, HM denote the Arithmetic mean, Geometric mean and Harmonic mean respectively the relationship between this is ______________

(a)

AM < GM < HM

(b)

AM ≤ GM ≤ HM

(c)

AM>GM>HM

(d)

AM≥GM≥HM

Slope of x-axis or a line parallel to x-axis is ______________

(a)

0

(b)

positive

(c)

negative

(d)

infinity

The co-ordinates of the foot of the perpendicular drawn from the point (2, 3) to the line 3x - y + 4 = 0 is ______________

(a)

\((\frac{1}{10},\frac{37}{10})\)

(b)

\((\frac{-1}{10},-\frac{37}{10})\)

(c)

\((\frac{-1}{10},\frac{37}{10})\)

(d)

\((\frac{37}{10},\frac{-1}{10})\)

If a_ij = \({1\over2}(3i-2j)\) and A = [a_ij]_2x2 is

(a)

\(\begin{bmatrix} {1\over 2}& 2 \\ -{1\over2} & 1 \end{bmatrix}\)

(b)

\(\begin{bmatrix} {1\over 2}& -{1\over2} \\ 2& 1 \end{bmatrix}\)

(c)

\(\begin{bmatrix} 2& 2\\ {1\over 2}& -{1\over2} \end{bmatrix}\)

(d)

\(\begin{bmatrix} -{1\over 2}& {1\over2} \\ 1& 2 \end{bmatrix}\)

The value of\(\left| \begin{matrix} 1 & 1 & 1 \\ 1 & 1+sin\theta & 1 \\ 1 & 1 & 1+cos\theta \end{matrix} \right| \) is _____________

(a)

3

(b)

1

(c)

2

(d)

\(\frac{1}{2}\)

If \(\overrightarrow{a}+2\overrightarrow{b}\) and \(3\overrightarrow{a}+m\overrightarrow{b}\) are parallel, then the value of m is

(a)

3

(b)

\(1\over3\)

(c)

6

(d)

\(1\over6\)

\(\underset { x\rightarrow \infty }{ lim } \left( \cfrac { { x }^{ 2 }+5x+3 }{ { x }^{ 2 }+x+3 } \right) ^{ x }\)is

(a)

e⁴

(b)

e²

(c)

e³

(d)

1

\(\lim _{ x\rightarrow \frac { \pi }{ 2 } }{ \frac { \sin { x } }{ x } } =\)

(a)

\(\pi \)

(b)

\(\frac { \pi }{ 2 } \)

(c)

\(\frac { 2 }{ \pi } \)

(d)

1

If y = f(x²+2) and f '(3) = 5, then \({dy\over dx}\) at x = 1 is

(a)

5

(b)

25

(c)

15

(d)

10

\(\int \frac{\sec x}{\sqrt{\cos 2 x}} d x\) is

(a)

tan^-1 (sin x)+c

(b)

2sin^-1(tan x)+c

(c)

tan^-1(cos x)+c

(d)

sin ^-1(tan x)+c

\(\int { \frac { \left( log{ x } \right) ^{ 3 } }{ x } } \) dx = _________+c.

(a)

\(\frac { \left( log{ x } \right) ^{ 4 } }{ 4 } \)

(b)

(sin^-1 x)⁴

(c)

(log x)⁴

(d)

\(\frac { 1 }{ 3logx } \)

A matrix is chosen at random from a set of all matrices of order 2, with elements 0 or 1 only. The probability that the determinant of the matrix chosen is non zero will be

(a)

\({3\over 16}\)

(b)

\({3\over 8}\)

(c)

\({1\over 4}\)

(d)

\({5\over 8}\)

The probabilities of a student getting I. II and III class in an examination are \(\frac { 1 }{ 10 } ,\frac { 3 }{ 5 } \) and \(\frac { 1 }{ 4 } \) respectively. The probability that the student fails in the examination is

(a)

\(\frac { 197 }{ 200 } \)

(b)

\(\frac { 27 }{ 100 } \)

(c)

\(\frac { 83 }{ 100 } \)

(d)

none of these

Find the number of subsets of A if A = \(\{x :x = 4n + 1, 2 \le n \le 5, n \in N\}.\)

Prove that cos (A + B) cos C - cos (B + c) cos A = sin B sin (C - A)

A group consists of 4 girls and 7 boys. In how many ways can a team of 5 members be selected, if the team has at least three girls

Determine the number of terms in the G. P {T_n} if T₁ = 3, T_n = 96 and S_n = 189.

Find the value of k and b, if the points P(-3, 1) and Q(2, b) lie on the locus of x² - 5x + ky = 0.

Find the direction cosines and direction ratios for the following vectors \(\hat{j}\)

\(If\lim _{ x\rightarrow 2 }{ \frac { { x }^{ n }-{ 2 }^{ n } }{ x-2 } } =80\quad and\quad n\in N,\quad find\quad n.\)

Find y''' if y = \({1\over x}\)

Integrate the following with respect to x : \({1\over \sqrt{1-25 x^2}}\)

Events A and B are such that P(A) = \(\frac { 1 }{ 2 } \) , P(B) = \(\frac { 7 }{ 12 } \) and P(not A or not B) = \(\frac { 1 }{ 4 } \). State whether A and B are independent? 

By taking suitable sets A, B, C, verify the following results:
(A\(\times\) B)\(\cap \)(B\(\times\)A) = (A\(\cap \)B) \(\times\) (B\(\cap \)A)

Ravi obtained 70 and 75 marks in first two unit tests. Find the minimum marks he should get in the third test to have an average of at least 60 marks.

Prove that cos A cos 2A cos 2² A cos2³ A ..... cos2^n-1A\(=\frac{sin2^nA}{2^nsinA}.\)

The first three terms in the expansion of (1 + ax)ⁿ are 1 + 12x + 64x². Find n and a

The normal boiling point of water is 100°C or 212°F· and the freezing point of water is 0 °C or 32°F.
(i) Find the linear relationship between C and F.
(ii) Find the value of C for 98.6°F and 
(iii) Find the value of F for 38°C.

Identify the singular and non-singular matrices:\(\begin{bmatrix} 2&-3 &5 \\ 6 & 0 &4 \\ 1 & 5 & -7 \end{bmatrix}\)

Do the limits of following functions exist as x\(\rightarrow 0?\) State reasons for your answer.\(x \left\lfloor x \right\rfloor \over sin |x|\)

Differentiate \(\log { (1+{ x }^{ 2 } } )\) with respect to \(\tan ^{ -1 }{ x } \)

Integrate the following functions with respect to x : e ^{8 - 7x}

The chances of A, B, and C becoming manager of a certain company are 5 : 3: 2. The probabilities that the office canteen will be improved if A, B, and C become managers are 0.4, 0.5 and 0.3 respectively. If the office canteen has been improved, what is the probability that B was appointed as the manager?

1. For the given curve, \(y=x^{1\over 3}\)given in  figure draw
   (i) \(y=-x^{ \left( \frac { 1 }{ 3 } \right) }\)
   (ii) ​\(y=x^{ \left( \frac { 1 }{ 3 } \right) }+1\)
   (iii)​ \(y=x^{ \left( \frac { 1 }{ 3 } \right) }-1\)
   (iii) ​\(y=(x+1)^{1\over 3}\)​
   

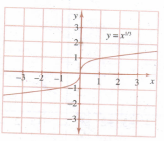
2. Determine the region in the Plane determined by the inequalities 3x+2y≤12,x≥1,y≥2

1. Prove that \(sinx+sin2x+sin3x=sin2x(1+2cosx)\)

2. Determine the number of 5 card combinations out of a deck of 52 cards if there is exactly three aces in each combination.

1. Compute the sum of first n terms of the following series 6 + 66 + 666 + .......

2. A photocopy store charges Rs. 1.50 per copy for the first 10 copies and Rs. 1.00 per copy after the 10th copy. Let x be the number of copies, and let y be the total cost of photocopying.
   (i) Draw graph of the cost as x goes from 0 to 50 copies 
   (ii) Find the cost of making 40 copies.

1. Find the points on the line x + y = 4 which lie at a unit distance from the line 4x + 3y = 10.

2. Without expanding the determinants, show that | B | = 2| A |.
   Where B =\(\begin{bmatrix} b+c & c+a & a+b \\ c+a & a+b &b+c \\a+b & b+c & c+a \end{bmatrix}\)and A =\(\begin{bmatrix} a& b & c \\ b & c & a \\ c & a & b \end{bmatrix}\)

1. If G is the centroid of a triangle ABC, prove that \(\overrightarrow{GA}\) + \(\overrightarrow{GB}\)  + \(\overrightarrow{GC}\) = \(\overrightarrow{0}\).

2. If the limit of f(x) as x approaches 2 is 4, can you conclude anything about f(2)? Explain reasoning.

1. If \(\log { ({ x }^{ 2 }+{ y }^{ 2 }) } =2\tan ^{ -1 }{ \frac { y }{ x } , } \) Show that \(\frac { dy }{ dx } =\frac { x+y }{ x-y } .\)

2. Find the integrals of the following : \({1\over \sqrt{x^2-4x+5}}\)

1. Evaluate \(\int { \frac { 1 }{ { x }^{ \frac { 1 }{ 2 } }+{ x }^{ \frac { 1 }{ 3 } } } } \)dx

2. In answering a question on a multiple choice test, a student either knows the answer or guesses. Let \(\frac { 3 }{ 4 } \) be the probability that he knows the answer and \(\frac { 1 }{ 4 } \) be the probability that he guesses. Assuming that a student who guesse at the answer will be correct with probability \(\frac { 1 }{ 4 } \). What is the probability that the student knows the answer given that he answered it correctly?