A vehicle is moving along the positive x direction, if sudden brake is applied, then

A particle of mass m sliding on the smooth double inclined plane (shown in figure) will experience

A ball with an initial momentum P collides normally with a rigid wall. If P₁ is the linear momentum after the perfectly elastic collision, then ____________.

A ball of 300 g mass moving with a speed of 20 m/s rebounds after striking normally a perfectly elastic wall. The change in momentum of a ball is ______________.

A batsman hits a ball straight in the direction of the bowler without change in its initial speed of 10 ms^-1. If the mass of the ball is 200 g, then change in momentum of the ball is ______________.

A block B is pushed momentarily along a horizontal surface with initial velocity v- μ coefficient of friction between B and surface, block B will come to rest after a time ___________.

A body of mass 'm' rests on a horizontal plane. It the angle of friction is ፀ, the least force required to move the body along the plane is (g = acceleration due to gravity).

The blocks of masses m and M connected by a spring are kept on a smooth horizontal frictionless table. A force f is applied to the mass M. If the acceleration of mass m is 'a' the acceleration of mass M will be ______________.

A bullet is fired from a gun. The force on the bullet is given by F = 600 - 2\(\times\)10⁵ t where, F is in newton and t in seconds. The force on the bullet becomes zero as soon as it leaves the barrel. What is the average impulse imparted to the bullet?

Three masses is in contact as shown. If force F is applied to mass m₁, the acceleration of three masses is _____________.

A body of mass 4 m is lying in xy-plane at rest. It suddenly explodes into three pieces. Two pieces each of mass m move perpendicular to each other with equal speed v. The total kinetic energy generated due to explosion is

What is the minimum velocity with which a body of mass m must enter a vertical loop of radius R so that it can complete the loop?

The work done by the conservative force for a closed path is

If the potential energy of the particle is \(\alpha -\frac { \beta }{ 2 } { x }^{ 2 }\), then force experienced by the particle is

A particle is placed at the origin and a force F = kx is acting on it (where k is a positive constant). If U (0) = 0, the graph of U(x) versus x will be (where U, is the potential , energy function)

The force on a particle as the function of displacement x is given by F = 9 + 0.3x. The work done corresponding to displacement of 6. particle from x = 0 to x = 2 unit is

A car is accelerated on a levelled road and attains a velocity 3 times of its initial velocity. In this process the potential energy of the car ________________.

If kinetic energy of a body is increased by 300% then percentage change in momentum will be ___________.

When a body moves with a constant, speed along a circle _______________.

In a gravitational field, the work done in moving a body from one point into another depends on _____________.

Why do passengers fall in backward direction when a bus suddenly starts moving from the rest position?

Find the acceleration when multiple forces act on the body?

Draw the graph for the variation of both static and kinetic frictional forces with external applied force?

State Aristotelian law of motion. What is flaw in this law.

What is frictional force?

Write the expression for centripetal force. Give two examples.

Proper inflation of tyres of vehicles saves fuel. Why?

Consider an object of mass 2 kg resting on the floor. The coefficient of static friction between the object and the floor is μ_s = 0.8. What force must be applied on the object to move it?

A passenger sitting in a car at rest, pushes the car from within. The car doesn't move, why?

Three blocks of masses 10 kg, 7 kg and 2 kg are placed in contact with each other on a frictionless table. A force of 50 N is applied on the heaviest mass. What is the acceleration of the system?

Define instantaneous power.

Can a body have energy without momentum?

Draw a graph showing the variation of potential energy of an object thrown vertically upward by a boy with respect to its height.

If energy is neither created nor destroyed, what happens to the so much energy spent against friction?

State the factors on which the work done by the force depends on.

Express a unit of electrical energy in terms of joule.

A box is pulled with a force of 25 N to produce a displacement of 15 m. If the angle between the force and displacement is 30°, find the work done by the force.

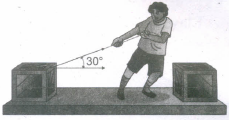
A variable force F = kx² acts on a particle which is initially at rest. Calculate the work done by the force during the displacement of the particle from x = 0 m to x = 4 m. (Assume the constant k = 1 N m^-2)

A light body and a heavy body have the same linear momentum. Which one has greater K.E?

Define spring constant of a spring.

Identify the forces acting on blocks A, B and C shown in the figure

Can the coefficient of friction be more than one?

A body of 3.5 kg in acted upon by two forces of magnitudes 3N and 5N making an angle of 90° with each other. Calculate the magnitude if net acceleration experience by the body is?

A rocket of mass 7000 kg is fired vertically. The acceleration of the rocket is 30 ms^-2 and the exhaust speed is 700 m/s. find the amount of gas ejected per second.

The force applied on both the objects is same, but the acceleration experienced by each object differs Why? Give an example.

A bus starts from rest accelerating uniformly with 4 ms^-2 At t= 10s, a stone is dropped out of a window of the bus 2m high. What are the
(i) magnitude of velocity and
(ii) acceleration of the stone at 10.2s? Take g = 10 ms^-2.

As shown in the diagram, three masses m, 3m and 5m connected together lie on a frictionless horizontal surface and pulled to the left by a force F. The tension T₁ in the first string is 24N. Find

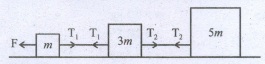
(i) acceleration of the system
(ii) tension in the second string and
(iii) force F

If the net force acting upon the particle is zero, show that its linear momentum remains constant.

The motion of a particle of mass m is described by h = ut + 1/2 gt². Find the force acting on particle.

A block of mass 500 g is at rest on a horizontal table. What steady force is required to give the block a velocity of 200 cms^-1 in 4 s?

How will you measure the work done? When
(i) the force acts along the direction of motion of the body and,
(ii) the force is inclined to the direction of motion of the body?

What is Non-conservative force? Give example.

One coolie takes 1 min to raise a box through a height 2m. Another takes 30 m/s for the same job and does the same amount of work. Which one of these two has a greater power?

An object of mass m = 1 kg is sliding from top to bottom in the frictionless inclined plane of inclination angle θ = 30° and the length of inclined plane is 10 m as shown in the figure. Calculate the work done by gravitational force and normal force on the object. Assume acceleration due to gravity, g = 10 m s^-2

A bullet of mass 0.02 kg is moving with a speed of 10 ms^-1. It can penetrate 10 cm of a wooden block, and comes to rest. If the thickness of the target would be 6 cm only, find the K.E. of the bullet when it comes out.

An elevator which can carry a maximum load of 1800 kg (elevator + passengers) is moving up at a constant speed of 2 ms^-1. The frictional force opposing the motion is 4000 N. Determine the minimum power delivered by the motor to the elevator in watts as well as in horsepower.

To simulate car accidents, auto manufacturers study the collisions of moving cars with mounted springs of different spring constants. Consider a typical simulation with a car of mass 1000 kg moving with a speed 18.0 kmh^-1 on a smooth road and colliding with a horizontally mounted spring of spring constant 6.25\(\times\)10^-3 Nm^-1. What is the maximum compression of the spring?

A gun fires 8 bullets per second into a target X. If the mass of each bullet is 3 g and its speed 600 s^-1. Then, calculate the power delivered by the bullets.

A pump on the ground floor of a building can pump up water to fill a tank of volume 30 m³ in 15 min. If the tank is 40 m above the ground, how much electric power is consumed by the pump. The efficiency of the pump is 30%.

State Newton's three laws and discuss their significance.

Briefly explain 'centrifugal force' with suitable examples.

Two masses m₁ and m₂ are connected with a string passing over a frictionless pulley fixed at the corner of the table as shown in the figure. The coefficient of static friction of mass m₁ with the table is μ_s, Calculate the minimum mass m₃ that may be placed on m₁ to prevent it from sliding. Check if m₁= 15 kg, m₂ = 10 kg, m₃ = 25 and μ_s = 0.2.

Find the impulse of a constant force and variable force with diagrams.

People often say "For every action there is an equivalent opposite reaction". Here they meant 'action of a human'. Is it correct to apply Newton's third law to human actions? What is mean by 'action' in Newton third law? Give your arguments based on Newton's laws.

What happens to the object at rest if
(i) f_s = 0 
(ii) fs = Fext 
(iii) fs = max.

Using Newton's laws calculate the tension acting on the mango (mass m = 400g) hanging from a tree.

Briefly explain how is a horse able to pull a cart.

Two masses m₁ and m₂ m₁ > m₂ or in contact with each other on a smooth horizontal surface. Calculate the magnitude of contact force between them.

As shown In the diagram, three blocks connected together lie on a horizontal frictionless table and pulled to the right with a force F = 50N. If m₁ = 5 kg, m₂ = 10 kg and m₃ = 15 kg. Find the tensions T₁ and T₂.

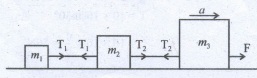
State and explain work energy principle. Mention any three examples for it.

Derive an expression for the velocity of the body moving in a vertical circle. And also find a tension at the bottom and the top of the circle.

Two different unknown masses A and B collide. A is initially at rest when B has a speed v. After collision B has a speed v/2 and moves at right angles to its original direction of motion. Find the direction in which A moves after collision?

A body of mass of 3 kg initially at rest makes under the action of an applied horizontally force of 10 N on a table with co-efficient of kinetic friction = 0.3, then what is the workdone by the applied force in 10s:

Find the workdone if a particle moves from position \(\vec { { r }_{ 1 } } =(2\overset { \wedge }{ i } +\overset { \wedge }{ j } -\overset { \wedge }{ 3k } )\) to a position \(\vec { { r }_{ 2 } } =(4\overset { \wedge }{ i } +6\overset { \wedge }{ j } -7\overset { \wedge }{ k } )\) under the effect of force \(\vec {F } =(3\overset { \wedge }{ i } +2\overset { \wedge }{ j } -\overset { \wedge }{ 4k } )\)N.

Calculate work done to move a boy of mass 20 kg along an inclined plane (\(\theta\) = 45°) with constent velocity through a distance of 10 m.

A 10kg ball and 20kg ball approach each other with velocities 20 ms^-1 and 10 ms^-1 respectively. What are their velocities after collision if the collision is perfectly elastic?

A shot travelling at the rate of 100 ms^-1 is just able to pierce a plank 4cm thick. What velocity is required to just pierce a plank 9cm thick?

Derive an expression for the potential energy of an elastic stretched spring.

If an object of mass 2 kg is thrown up from the ground reaches a height of 5 m and falls back to the Earth (neglect the air resistance). Calculate
(a) The work done by gravity when the object reaches 5 m height
(b) The work done by gravity when the object comes back to Earth
(c) Total work done by gravity both in upward and downward motion and mention the physical significance of the result.