New ! Physics MCQ Practise Tests



  1. Home
  2. 11th Standard
  3. Physics
  4. 11th Standard Physics Model Revision Test Question Paper 2018

Updated 11th Standard Physics Study Materials 2022-2023 Exams

11th Standard Physics Model Revision Test Question Paper 2018 Feb-18 , 2019

11th Public Model Exam 2019

11th Standard Physics Model Revision Test Question Paper 2018

11th Public Model Exam 2019

11th Standard

    Reg.No. :
  •  
  •  
  •  
  •  
  •  
  •  

Physics

Time : 02:30:00 Hrs
Total Marks : 70
    15 x 1 = 15

  1. If the length and time period of an oscillating pendulum have errors of 1% and 3% respectively then the error in measurement of acceleration due to gravity is

    (a)

    4%

    (b)

    5%

    (c)

    6%

    (d)

    7%

  2. An object is dropped in an unknown planet from height 50 m, it reaches the ground in 2 s. The acceleration due to gravity in this unknown planet is

    (a)

    g = 20 ms-2

    (b)

    g = 25 ms-2

    (c)

    g = 15 ms-2

    (d)

    g = 30 ms-2

  3. A butterfly and stone (mass of later is greater than earlier) is moving with same velocity. Momentum of the stone is____________ than the momentum of butterfly.

    (a)

    equal

    (b)

    greater

    (c)

    lesser

    (d)

    lesser (or) equal to

  4. Force acting on the particle moving with constant speed is

    (a)

    always zero

    (b)

    need not be zero

    (c)

    always non zero

    (d)

    cannot be concluded

  5. If the potential energy of the particle is \(\alpha -\frac { \beta }{ 2 } { x }^{ 2 }\), then force experienced by the particle is

    (a)

    F = \(\frac { \beta }{ 2 } { x }^{ 2 }\)

    (b)

    F = βx

    (c)

    F = -βx

    (d)

    F = -\(\frac { \beta }{ 2 } { x }^{ 2 }\)

  6. A round object of mass M and radius R rolls down without slipping along an inclined plane. The fractional force, 

    (a)

    dissipates kinetic energy as heat

    (b)

    decreases the rotational motion

    (c)

    decreases the rotational and transnational motion

    (d)

    converts transnational energy into rotational energy

  7. Analogue of mass in rotational motion is ___________.

    (a)

    M.l.

    (b)

     Angular momentum

    (c)

    Gyration

    (d)

    Torque

  8. The magnitude of the Sun’s gravitational field as experienced by Earth is

    (a)

    same over the year

    (b)

    decreases in the month of January and increases in the month of July

    (c)

    decreases in the month of July and increases in the month of January

    (d)

    increases during day time and decreases during night time

  9. For a given material, the rigidity modulus is \(\left( \frac { 1 }{ 3 } \right) \)rd of Young’s modulus. Its Poisson’s ratio is

    (a)

    0

    (b)

    0.25

    (c)

    0.3

    (d)

    0.5

  10. Amount of energy required to change liquid to gas and vice versa without any change in temperature is termed as __________________.

    (a)

    Latent Heat and Fusion

    (b)

    Latent Heat of Yaporis ation

    (c)

    Heat capacity

    (d)

    Specific heat capacity

  11. A hot cup of coffee is kept on the table. After some time it attains a thermal equilibrium with the surroundings. By considering the air molecules in the room as a thermodynamic system, which of the following is true

    (a)

    ΔU > 0, Q = 0

    (b)

    ΔU > 0, W < 0

    (c)

    ΔU > 0, Q > 0

    (d)

    ΔU = 0, Q > 0

  12. An ideal gas is maintained at constant pressure. If the temperature of an ideal gas increases from 100K to 1000K then the rms speed of the gas molecules

    (a)

    increases by 5 times

    (b)

    increases by 10 times

    (c)

    remains same

    (d)

    increases by 7 times

  13. The length of a second’s pendulum on the surface of the Earth is 0.9 m. The length of the same pendulum on surface of planet X such that the acceleration of the planet X is n times greater than the Earth is

    (a)

    0.9n

    (b)

    \(\frac{0.9}{n}\)m

    (c)

    0.9n2m

    (d)

    \(\frac{0.9}{n^2}\)

  14. What is time period of a pendulum hanged in a satellite? (T is time period on earth)

    (a)

    zero

    (b)

    T

    (c)

    infinite

    (d)

    \(\frac { T }{ \sqrt { 6 } } \)

  15. An air column in a pipe which is closed at one end, will be in resonance with the vibrating body of frequency 83Hz. Then the length of the air column is

    (a)

    1.5 m

    (b)

    0.5 m

    (c)

    1.0 m

    (d)

    2.0 m

    16. 6x 2 = 12

  16. In an ocean surveillance system of ship fitted with a (RADAR), the time delay between generation of a radio waves reflected from an enemy ship is observed to be 5.6s. Calculate the distance of the enemy ship from the surveillance ship.

  17. A particle is projected at an angle of θ with respect to the horizontal direction. Match the following for the above motion.
    (a) vx - decreases and increases
    (b) vy - remains constant
    (c) Acceleration - varies
    (d) Position vector - remains downward

  18. Why it is not possible to push a car from inside?

  19. If energy is neither created nor destroyed, what happens to the so much energy spent against friction?

  20. A small particle of mass m is projected with an initial velocity v at an angle \(\theta\) with x-axis in X-Y plane as shown in Figure.

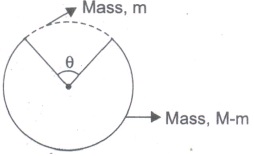
    Find the angular momentum of the particle.

  21. What do you mean by capillarity or capillary action?

  22. In a petrol engine, (internal combustion engine) air at atmospheric pressure and temperature of 20°C is compressed in the cylinder by the piston to 1/8 of its original volume. Calculate the temperature of the compressed air. (For air \(\gamma \) = 1.4)

  23. What is meant by periodic and nonperiodic motion? Give any two examples, for each motion.

  24. What is Echo?

    25. 6 x 3 = 18

  25. The vernier scale of a travelling microscope has 50 divisions which coincide with 49 main scale divisions. If each main scale division is 0.5 mm. Calculate the minimum inaccuracy in the measurement of distance.

  26. A person initially at rest starts to walk 2 m towards north, then 1 m towards east, then 5 m towards south and then 3 m towards west. What is the position vector of the person at the end of the trip?

  27. Explain various types of friction. Suggest a few methods to reduce friction.

  28. Which of the two kilowatt hour or electron volt is a bigger unit of energy and by what factor?

  29. Three masses 3 kg, 4 kg and 5 kg are located at the corners of an equilateral triangle of side 1 m. Locate the center of mass of the system.

  30. Find the expression of the orbital speed of satellite revolving around the earth.

  31. Two students want to increase the temperature of a gas without adding heat to it. Is it possible to increase the temperature of a gas without adding heat to it?

  32. At what temperature the rms speed of oxygen atom equal to rms speed of helium gas atom at 10°C?
    Atomic mass of helium = 4
    Atomic mass of oxygen = 32

  33. Two vibrating tuning forks produce waves whose equation is given by y1 = 5 sin(240\(\pi\)t) and y2 = 4 sin(244πt). Compute the number of beats per second.

    34. 5 x 5 = 25

    1. Check the correctness of the equation\(\frac { 1 }{ 2 } \)mv2 = mgh using dimensional analysis method.

    2. Two bodies A and B are moving with velocities VA and VB making an 'θ' with each other. Determine the relative velocity of A with respect to B. What will be the relative velocity.
      (i) When 2 bodies are moving in the same direction.
      (ii) When 2 bodies are moving in the opposite direction.
      (iii) When 2 bodies are moving at right angle to each other.

    1. An object of mass 10 kg moving with a speed of 15 ms-1 hits the wall and comes to rest within
      (a) 0.03 second
      (b) 10 second.
      Calculate the impulse and average force acting on the object in both the cases.

    2. Derive an expression for the gravitational potential energy of a body of mass 'm' raised to a height 'h' above the earth's surface.

    1. Derive the relation between rotational KE and angular momentum.

    2. Explain the variation of g with lattitude.

    1. Derive an expression for the elastic energy stored per unit volume of a wire.

    2. Explain the second law of thermodynamics in terms of entropy.

    1. From a certain apparatus, the diffusion rate of hydrogen has an average value of 28.7 cm3/s, The diffusion of another gas under the same condition is measured to have an average rate of 7.2 cm3/s. Identify the gas.

    2. Consider a particle undergoing simple harmonic motion. The velocity of the particle at position x1 is v1 and velocity of the particle at position x2 is v2. Show that the ratio of time period and amplitude is 
      \(\frac { T }{ A } =2\pi \sqrt { \frac { { x }_{ 2 }^{ 2 }-{ x }_{ 1 }^{ 2 } }{ { { v }_{ 1 }^{ 2 }x }_{ 2 }^{ 2 }-{ { v }_{ 2 }^{ 2 }x }_{ 1 }^{ 2 } } } \)

*****************************************

Reviews & Comments about 11th Standard Physics Model Revision Test Question Paper 2018

viswa 24-Feb-2019

how to download these questions from qb365

Bala 16-May-2019

How to download the solution for this question paper

Write your Comment