Explain in detail the various types of errors. 

Write short notes on the following.
(a) Unit

In a submarine equipped with sonar, the time delay between the generation of a pulse and its echo after reflection from an enemy submarine is observed to be 80 sec. If the speed of sound in water is 1460 ms^-1. What is the distance of enemy submarine?

Assuming that the frequency \(\gamma\) of a vibrating string may depend upon
(i) applied force (F) 
(ii) length (I)
(ill) mass per unit length (m), prove that \(\gamma\alpha{{1}\over{l}}\sqrt{{{F}\over{m}}}\) using dimensional analysis.

The measurement value of length of a simple pendulum is 20cm known with 2mm accuracy. The time for 50 oscillations was measured to be 40 s within is resolution. Calculate the percentage accuracy in the determination of acceleration due to gravity 'g' from the above measurement.

Write to causes of errors in measurement.

How will you determine the distance of moon from earth using parallax method?

Briefly explain the different types of errors and their causes with an example. How can these error be minimised?

Explain the propagation of errors in subtraction, quotient and power of a quantity.

The value Gin CGS system is 6.67\(\times\)10^-8 dyne cm² g^-2. Calculate the value in SI units.

Check the dimensional consistency of the following equations.
(i) de-Broglie wavelength, \(\lambda ={h\over mv}\)
(ii) Escape velocity, v = \({\sqrt{2GM\over R}}\)

A planet moves around the sun in nearly circular orbit. Its period of revolution 'T' depends upon.
(i) radius 'r' of orbit 
(ii) mass 'm' of the sun and
(iii) The gravitational constant G Show dimensionally that T² \(\propto\)r³.

In a series of successive measurements in an experiment, the readings of the period of oscillation of a simple pendulum were found to be 2.63s, 2.56s, 2.42s, 2.71s, and 2.80s.
Calculate
(i) the mean value of the period of oscillation
(ii) the absolute error in each measurement
(iii) the mean absolute error
(iv) the relative error
(v) the percentage error.
(vi) Express the result in proper form.

Arrive at Einstein's mass-energy relation by dimensional method (E = mc²).

Two resistors of resistances R₁= 150 ± 2 Ohm and R₂ = 220 ± 6 Ohm are connected in parallel combination. Calculate the equivalent resistance.
Hint:\(\frac{1}{R'}=\frac{1}{R_1}+\frac{1}{R_2}\)

Convert 76 cm of mercury pressure into Nm^-2 using the method of dimensions.

One mole of an ideal gas at STP occupies 22.4 L. What is the ratio of molar volume to atomic volume of a mole of hydrogen? Why is the ratio so large? Take radius of hydrogen molecule to be 1^oA.

The frequency of vibration of a string depends of on,
(i) tension in the string
(ii) mass per unit length of string
(iii) vibrating length of the string
Establish dimensionally the relation for frequency.

Explain the principle of homogeniety of dimensions. What are its uses? Give example

A particle has its position moved from \(\overset { \rightarrow }{ { r }_{ 1 } } =3\hat { i } +4\hat { j } \) to \(\overset { \rightarrow }{ { r }_{ 2 } } =\hat { i } +2\hat { j } \) Calculate the displacement vector (\(\Delta \)\(\overrightarrow { r } \)) and draw the \(\overrightarrow { { r }_{ 1 } } \), \(\overrightarrow { { r }_{ 2 } } \) and \(\Delta \overrightarrow { r } \) vector in a two dimensional cartesian coordinate system.

An object at an angle such that the horizontal range is 4 times of the maximum height. What is the angle of projection of the object?

A foot-ball player hits the ball with speed 20 ms^-1 with angle 30° with respect to horizontal direction as shown in the figure. The goal post is at distance of 40 m from him. Find out whether ball reaches the goal post.

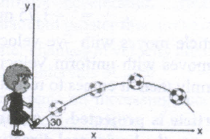
A car moving uniform motion with speed 120 kmh^-2 is brought to a stop within a distance of 200 m. How long does it take for the car to stop?

Calculate the vector which has to be added to the resultant of \(\overrightarrow { A } =2\overrightarrow { i } -3\overrightarrow { j } -4\overrightarrow { k } \) and \(\overrightarrow { B } =6\hat { i } -4\hat { j } -4\hat { k } \)  gives unit vector along x - direction.

A person travels along a straight road for the first half distance 4 m with a velocity 1 ms^-1 and the second half distance 3 m with a velocity 0.7 ms^-1 What is the mean velocity of the person.

The position of a particle is given by r = 2.00t \(\hat { i } -1.00{ t }^{ 2 }\hat { j } +3.00\hat { k } \) where t is in seconds and the coefficients have the proper units for r to be in metres. Find the velocity and acceleration of a particle then what is the magnitude and direction of velocity of the particle at t = 2 s?

Explain the resolution of vectors in three dimensional co-ordinates.

Define and illustrate the following terms.
(i) Equal vectors
(il) Parallel vectors
(iii) Anti-parallel vectors
(iv) Unit vector.

A projectile is fired horizontally with a velocity u. Show that its trajectory is a parabola. Also obtain the expression for
(i) Time of flight.
(ii) Horizontal range.

A van is moving along x-axis. As shown in the figure, it moves from 0 to P in 18s and returns from P to Q in 6s. What are the average velocity and average speed of the van in going from 
(i) from O to P
(ii) from O to P and back to Q?

A ball is thrown upward with an initial velocity of 100ms^-1. After how much time will it return? Draw velocity-time graph for the ball and find from the graph.
(i) the maximum height attained by the ball
(ii) the height of the ball after 15 s. Take g= 10 ms^-2.

On a certain day, rain was falling vertically with a speed of 35 ms^-1. A wind started biowing after sometime with a speed of 12 ms^-1 in east to west direction. In which direction should boy waiting at a bus stop hold his umbrella?

Consider the function Y = x². Calculate the derivative \(\frac{dy}{dx}\) using the concept of limit. at the point x = 2.

Explain the types of motion with example.

Explain the concept of relative velocity in one and two dimensional motion.

Shows that the path of horizontal projectile is a parabola and derive an expression for
(i) Time of flight
(ii) Horizontal range
(iii) resultant relative and any instant
(iv) speed of the projectile when it hits the ground?

Derive the relation between Tangential acceleration and angular acceleration.

A three storey building of height 100m is located on Earth and a similar building is also located on Moon. If two people jump from the top of these buildings on Earth and Moon simultaneously, when will they reach the ground and at what speed? (g = 10m s^-2)

Draw the resultant direction of the two unit vectors \(\hat i\) and \(\hat j\). Use a 2-dimensional Cartesian co-ordinate system. is \(\hat i+\hat j\) a unit vector?

Describe the method of measuring angle of repose.

Calculate the centripetal acceleration of Moon towards the Earth.

Two masses m₁ and m₂ are connected with a string passing over a frictionless pulley fixed at the corner of the table as shown in the figure. The coefficient of static friction of mass m₁ with the table is μ_s, Calculate the minimum mass m₃ that may be placed on m₁ to prevent it from sliding. Check if m₁= 15 kg, m₂ = 10 kg, m₃ = 25 and μ_s = 0.2.

Write the Salient features of Static and Kinetic friction

Imagine that the gravitational force between Earth and Moon is provided by an invisible string that exists between the Moon and Earth. What is the tension that exists in this invisible string due to Earth's centripetal force? (Mass of the Moon = 7.34\(\times\)10²² kg, Distance between Moon and Earth = 3.84 \(\times\) 10⁸m).

Give some examples for centripetal force.

Describe Galileo's experiments concerning motion of objects on inclined planes?

Prove Impulse - Momentum equation.

Show how impulse force can be measured graphically.

What happens to the object at rest if
(i) f_s = 0 
(ii) fs = Fext 
(iii) fs = max.

Using Newton's laws calculate the tension acting on the mango (mass m = 400g) hanging from a tree.

Briefly explain how is a horse able to pull a cart.

Derive an expression for the acceleration of the body sliding down a frictionless surface.

Two masses m₁ and m₂ m₁ > m₂ or in contact with each other on a smooth horizontal surface. Calculate the magnitude of contact force between them.

Briefly explain how is a vehicle able to go round a level curved track. Determine the maximum speed with which the vehicle can negotiate this curved track safely.

As shown In the diagram, three blocks connected together lie on a horizontal frictionless table and pulled to the right with a force F = 50N. If m₁ = 5 kg, m₂ = 10 kg and m₃ = 15 kg. Find the tensions T₁ and T₂.

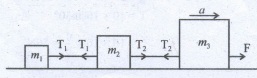
Identify the internal and external forces acting on the following systems.
(a) Earth alone as a system
(b) Earth and Sun as a system
(c) Our body as a system while walking
(d) Our body + Earth as a system

Two bodies of masses 7 kg and 5 kg are connected by a light string passing over a smooth pulley at the edge of the table as shown in the figure. The coefficient of static friction between the surfaces (body and table) is 0.9. Will the mass m₁ = 7 kg on the surface move? If not what value of m₂ should be used so that mass 7 kg begins to slide on the table?

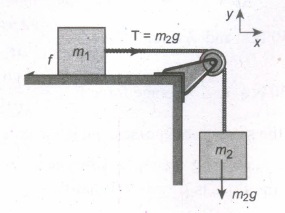
A block 1 of mass m₁, constrained to move along a plane. inclined at angle e to the horizontal, is connected via a massless inextensible string that passes over. a massless pulley, to a second block 2 of mass m₂. Assume the coefficient of static friction between the block and the inclined plane is and μ_s the coefficient of kinetic friction is μ_s.
What is the relation between the masses of block 1 and block 2 such that the system just starts to slip?

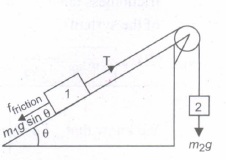
In the section 3.7.3 (Banking of road) we have not included the friction exerted by the road on the car. Suppose the coefficient of static friction between the car tyre and the surface of the road is, calculate the minimum speed with which the car can take safe turn? When the car takes turn in the banked road, the following three forces act on the car.
(1) The gravitational force mg acting downwards.
(2) The normal force N acting perpendicular to the surface of the road.
(3) The static frictional force f acting on the car along the surface.