\(lim_{x\rightarrow\infty}{sin \ x \over x} \)

If f(x) = x(-1)^{\(\left\lfloor 1\over x \right\rfloor \)}, \(x\le0\), then the value of \(lim_{x\rightarrow 0}f(x)\) is equal to

If \(lim_{x \rightarrow 0}{sin \ px\over tan \ 3x}=4\) , then the value of p is

\(lim_{x \rightarrow 0}{e^{tan \ x}-e^x\over tan x-x}=\)

The function \(f(x)= \begin{cases}\frac{x^{2}-1}{x^{3}+1} & x \neq-1 \\ P & x=-1\end{cases}\)is not defined for x = −1. The value of f(−1) so that the function extended by this value is continuous is

Consider the function f(x) = \(\sqrt{x},x\ge0.\) Does\(lim_{x\rightarrow0}f(x)\) exist?

In problems 1-6, using the table estimate the value of the limit.
\(lim_{x\rightarrow 2}{x-2\over x^2-x-2}\)

In problem, using the table estimate the value of the limit
\(lim_{x\rightarrow{-3}}{\sqrt{1-x}-2\over x+3}\)

Compute \(lim_{x\rightarrow1}{x^3-1\over x-1}\)

\(f(x)=tan \ x \ at \ x={\pi\over 2}.\)

Evaluate the following limits :\(lim_{x\rightarrow \infty}(1+{k\over x})^{m\over x} \)

Examine the continuity of the following: x + sin x

Examine the continuity of the following:cot x + tan x

Calculate \(\lim _{ x\rightarrow0}{|x| } \).

Use the graph to find the limits (if it exists). If the limit does not exist, explain why?
\(lim_{x\rightarrow2}f(x)\)

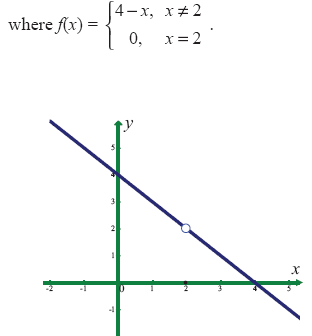
Use the graph to find the limits (if it exists). If the limit does not exist, explain why?
\(lim_{x\rightarrow5}{|x-5|\over x-5}\)

Check if \(lim_{x\rightarrow-58}f(x)\)exists or not, where \(f(x)=\left\{\begin{array}{cc} \frac{|x+5|}{x+5} & , \text { for } x \neq-5 \\ 0, & \text { for } x=-5 \end{array}\right.\)

Write a brief description of the meaning of the notation \(lim_{x\rightarrow8}f(x)=25.\)

Evaluate the following limits :
\(lim_{x\rightarrow5}{\sqrt{x-1}-2\over x-5}\)

A tomato wholesaler finds that the price of a newly harvested tomatoes is Rs. 0.16 per kg if he purchases fewer than 100 kgs each day. However, if he purchases at least 100 kgs daily, the price drops to Rs. 0.14 per kg. Find the total cost function and discuss the cost when the purchase is 100 kgs.