New ! Physics MCQ Practise Tests



  1. Home
  2. 11th Standard
  3. Physics
  4. 11th Standard Physics - Oscillations Model Question Paper

Updated 11th Standard Physics Study Materials 2022-2023 Exams

11th Standard Physics - Oscillations Model Question Paper Oct-09 , 2019

Oscillations 

11th Standard Physics - Oscillations Model Question Paper

Oscillations Model Question Paper

11th Standard

    Reg.No. :
  •  
  •  
  •  
  •  
  •  
  •  

Physics

Time : 02:00:00 Hrs
Total Marks : 60
    8 x 1 = 8

  1. In a simple harmonic oscillation, the acceleration against displacement for one complete oscillation will be

    (a)

    an ellipse

    (b)

    a circle

    (c)

    a parabola

    (d)

    a straight line

  2. The length of a second’s pendulum on the surface of the Earth is 0.9 m. The length of the same pendulum on surface of planet X such that the acceleration of the planet X is n times greater than the Earth is

    (a)

    0.9n

    (b)

    \(\frac{0.9}{n}\)m

    (c)

    0.9n2m

    (d)

    \(\frac{0.9}{n^2}\)

  3. A spring is connected to a mass m suspended from it and its time period for vertical oscillation is T. The spring is now cut into two equal halves and the same mass is suspended from one of the halves. The period of vertical oscillation is

    (a)

    T'= \(\sqrt{2}\)T

    (b)

    \(T'=\frac { T }{ \sqrt { 2 } } \)

    (c)

    T'=\(\sqrt{2T}\)

    (d)

    \(T'=\sqrt { \frac { T }{ 2 } } \)

  4. An ideal spring of spring constant k, is suspended from the ceiling of a room and a block of mass M is fastened to its lower end. If the block is released when the spring is un-stretched, then the maximum extension in the spring is

    (a)

    4\(\frac { Mg }{ k } \)

    (b)

    \(\frac { Mg }{ k } \)

    (c)

    2\(\frac { Mg }{ k } \)

    (d)

    \(\frac { Mg }{ 2k } \)

  5. A pendulum is hung in a very high building oscillates to and fro motion freely like a simple harmonic oscillator. If the acceleration of the bob is 16 ms−2 at a distance of 4 m from the mean position, then the time period is

    (a)

    2 s

    (b)

    1 s

    (c)

    2\(\pi\)s

    (d)

    \(\pi\)s

  6. The damping force on an oscillator is directly proportional to the velocity. The units of the constant of proportionality are

    (a)

    kgms−1

    (b)

    kgms−2

    (c)

    kgs−1

    (d)

    kgs

  7. A mass of 3 kg is attached at the end of a spring moves with simple harmonic motion on a horizontal frictionless table with time period 2π and with amplitude of 2m, then the maximum fore exerted on the spring is

    (a)

    1.5 N

    (b)

    3 N

    (c)

    6 N

    (d)

    12 N

  8. The x-t graph of a particle undergoing simple harmonic motion is shown. The acceleration of the particle at t =\(\frac{4}{3}\) is ______________.

    (a)

    \(\frac { \sqrt { 3 } }{ 32 } \pi \) cm/s2

    (b)

    \(\frac { -{ \pi }^{ 2 } }{ 32 } \) cm/s2

    (c)

    \(\frac { { \pi }^{ 2 } }{ 32 } \) cm/s2

    (d)

    \(\frac { -\sqrt { 3 } }{ 32 } \)

    9. 10 x 2 = 20

  9. Classify the following motions as periodic and non-periodic motions?
    a. Motion of Halley’s comet.
    b. Motion of clouds.
    c. Moon revolving around the Earth

  10. A mass m moves with a speed v on a horizontal smooth surface and collides with a nearly massless spring whose spring constant is k. If the mass stops after collision, compute the maximum compression of the spring.

  11. What is meant by force constant of a spring?

  12. What is an epoch?.

  13. State the laws of simple pendulum?

  14. What is meant by free oscillation?

  15. Explain damped oscillation. Give an example.

  16. Compute the time period for the following system if the block of mass m is slightly displaced vertically down from its equilibrium position and then released. Assume that the pulley is light and smooth, strings and springs are light.

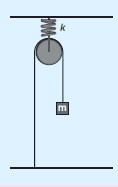
  17. What is Oscillatory motion?

  18. Define S.H.M.

    19. 4 x 3 = 12

  19. A nurse measured the average heart beats of a patient and reported to the doctor in terms of time period as 0.8 s. Express the heart beat of the patient in terms of number of beats measured per minute.

  20. Consider two springs with force constants 1 N m−1 and 2 N m−1 connected in parallel. Calculate the effective spring constant (kp) and comment on kp.

  21. Write down the kinetic energy and total energy expressions in terms of linear momentum, For one-dimensional case.

  22. State five characteristics of SHM.

    23. 4 x 5 = 20

  23. Consider a particle undergoing simple harmonic motion. The velocity of the particle at position x1 is v1 and velocity of the particle at position x2 is v2. Show that the ratio of time period and amplitude is 
    \(\frac { T }{ A } =2\pi \sqrt { \frac { { x }_{ 2 }^{ 2 }-{ x }_{ 1 }^{ 2 } }{ { { v }_{ 1 }^{ 2 }x }_{ 2 }^{ 2 }-{ { v }_{ 2 }^{ 2 }x }_{ 1 }^{ 2 } } } \)

  24. What is meant by simple harmonic oscillation? Give examples and explain why every simple harmonic motion is a periodic motion whereas the converse need not be true.

  25. Discuss the simple pendulum in detail.

  26. Discuss in detail the energy in simple harmonic motion.

*****************************************

Reviews & Comments about 11th Standard Physics - Oscillations Model Question Paper

Write your Comment