- State Board
-
12th Standard
-

Biology
-

Computer Applications
-

Computer Science
-

Business Maths and Statistics
-

Commerce
-

Economics
-

Maths
-

Chemistry
-

Physics
-

Computer Technology
-

History
-

Accountancy
-

Tamil
-

Maths
-

Chemistry
-

Physics
-

Biology
-

Computer Science
-

Business Maths and Statistics
-

Economics
-

Commerce
-

Accountancy
-

History
-

Computer Applications
-

Computer Technology
-

English
12th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
11th Standard
-

Maths
-

Biology
-

உயிரியல் - தாவரவியல்
-

Economics
-

Physics
-

Chemistry
-

History
-

Business Maths and Statistics
-

Computer Science
-

Accountancy
-

Commerce
-

Computer Applications
-

Computer Technology
-

Tamil
-

Maths
-

Commerce
-

Economics
-

Biology
-

Business Maths and Statistics
-

Accountancy
-

Computer Science
-

Physics
-

Chemistry
-

Computer Applications
-

History
-

Computer Technology
-

Tamil
-

English
11th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
9th Standard
-

-

-

-

-

-

-

Maths
-

Science
-

Social Science
-

Maths
-

Science
-

Social Science
9th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
6th Standard
-

Maths
-

Science
-

Social Science
-

Maths
-

Science
-

Social Science
6th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
10th Standard
-

Maths
-

Science
-

Social Science
-

Tamil
-

Maths
-

Science
-

Social Science
-

English
-

English
10th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
7th Standard
-

Maths
-

Science
-

Maths
-

Science
-

Social Science
7th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
8th Standard
-

கணிதம் - old
-

Science
-

Social Science
-

கணிதம்
-

Maths
-

Science
-

Social Science
8th Standard stateboard question papers & Study material
தமிழ் Subjects
English Subjects
-
-
12th Standard
- CBSE Board
-
12th Standard CBSE
-

Biology
-

Physics
-

Chemistry
-

Maths
-

Accountancy
-

Introductory Micro and Macroeconomics
-

Business Studies
-

Economics
-

Computer Science
-

Geography
-

English
-

History
-

Indian Society
-

Physical Education
-

Sociology
-

Tamil
-

Bio Technology
-

Engineering Graphics
-

Entrepreneurship
-

Hindi Core
-

Hindi Elective
-

Home Science
-

Legal Studies
-

Political Science
-

Psychology
12th Standard CBSE Subject Question Paper & Study Material
-
-
11th Standard CBSE
-

Mathematics
-

Chemistry
-

Biology
-

Physics
-

Business Studies
-

Accountancy
-

Economics
-

Computer Science
-

Bio Technology
-

English
-

Enterprenership
-

Geography
-

Hindi
-

History
-

Home Science
-

Physical Education
-

Political Science
-

Psychology
-

Sociology
-

Applied Mathematics
11th Standard CBSE Subject Question Paper & Study Material
-
- 10th Standard CBSE
-
9th Standard CBSE
-

Mathematics
-

Social Science
-

Science
-

English
-

Hindi
9th Standard CBSE Subject Question Paper & Study Material
-
-
8th Standard CBSE
-

Science
-

Social Science
-

Mathematics
-

English
8th Standard CBSE Subject Question Paper & Study Material
-
-
7th Standard CBSE
-

Mathematics
-

Science
-

Social Science
-

English
7th Standard CBSE Subject Question Paper & Study Material
-
-
6th Standard CBSE
-

Mathematics
-

Science
-

Social Science
-

English
6th Standard CBSE Subject Question Paper & Study Material
-
-
12th Standard CBSE
- Free Online Test
- News
- Study Materials
-
Students
-

Stateboard Tamil Nadu
-

CBSE Board
-

Free Online Tests
-

Educational News
-

Scholarships
-

Entrance Exams India
-

Video Materials
Study Materials , News and Scholarships
-
-
Students
Term II Model Question Paper
12th Standard
-
Reg.No. :
Business Maths
Time :
02:30:00 Hrs
Total Marks :
90
-
The rank of the unit matrix of order n is ________.
(a)n −1
(b)n
(c)n +1
(d)n2
-
Cramer’s rule is applicable only to get an unique solution when _______.
(a)\({ \triangle }_{ z }\neq 0\)
(b)\({ \triangle }_{ x }\neq 0\)
(c)\({ \triangle } \neq 0\)
(d)\({ \triangle }_{ y }\neq 0\)
-
The value of \(\int _{ -\frac{\pi}{2}}^{ \frac{\pi}{2}}\) cos x dx is _______.
(a)0
(b)2
(c)1
(d)4
-
The marginal revenue and marginal cost functions of a company are MR = 30 − 6x and MC = −24 + 3x where x is the product, then the profit function is ________.
(a)9x2 + 54x
(b)9x2 − 54x
(c)54x - \(\frac { { 9x }^{ 2 } }{ 2 } \)
(d)54x - \(\frac { { 9x }^{ 2 } }{ 2 } \) + k
-
The complementary function of (D2+ 4)y = e2x is ______.
(a)(Ax +B)e2x
(b)(Ax +B)e−2x
(c)A cos 2x + B sin 2x
(d)Ae−2x+ Be2x
-
If c is a constant then Δc = _______.
(a)c
(b)Δ
(c)Δ2
(d)0
-
E f (x)= _______.
(a)f(x− h)
(b)f (x)
(c)f(x+ h)
(d)f(x+ 2h)
-
If c is a constant, then E(c) is ________.
(a)0
(b)1
(c)c f (c)
(d)c
-
The probability density function p(x) cannot exceed ________.
(a)zero
(b)one
(c)mean
(d)infinity
-
In a parametric distribution the mean is equal to variance is ________.
(a)binomial
(b)normal
(c)poisson
(d)all the above
-
If P(Z > z) = 0.5832 what is the value of z (z has a standard normal distribution)?
(a)-0.48
(b)0.48
(c)1.04
(d)-0.21
-
A _______is one where each item in the universe has an equal chance of known opportunity of being selected.
(a)Parameter
(b)random sample
(c)statistic
(d)entire data
-
An estimator is said to be ________ if it contains all the information in the data about the parameter it estimates.
(a)efficient
(b)sufficient
(c)unbiased
(d)consistent
-
The standard error of sample mean is ______.
(a)\(\frac { \sigma }{ \sqrt { 2n } } \)
(b)\(\frac { \sigma }{ { n } } \)
(c)\(\frac { \sigma }{ \sqrt { n } } \)
(d)\(\frac { { \sigma }^{ 2 } }{ \sqrt { n } } \)
-
The value of ‘b’ in the trend line y = a + bx is ________.
(a)Always positive
(b)Always negative
(c)Either positive or negative
(d)Zero
-
Consumer price index are obtained by: ________.
(a)Paasche’s formula
(b)Fisher’s ideal formula
(c)Marshall Edgeworth formula
(d)Family budget method formula
-
\(\overset {-}{X}\) chart is a ________.
(a)attribute control chart
(b)variable control chart
(c)neither Attribute nor variable control chart
(d)both Attribute and variable control chart
-
The Penalty in VAM represents difference between the first ________.
(a)Two largest costs
(b)Largest and Smallest costs
(c)Smallest two costs
(d)None of these
-
If number of sources is not equal to number of destinations, the assignment problem is called______.
(a)balanced
(b)unsymmetric
(c)symmetric
(d)unbalanced
-
A type of decision –making environment is _______.
(a)certainty
(b)uncertainty
(c)risk
(d)all of the above
-
Find the rank of each of the following matrices.
\(\left( \begin{matrix} 5 & 6 \\ 7 & 8 \end{matrix} \right) \) -
If f'(x) = x + b, f(1)= 5 and f(2) = 13, then find f(x)
-
Integrate the following with respect to x
\(\sqrt { { 1+x+x }^{ 2 } } \) -
If the marginal cost function of x units of output is \(\frac { a }{ \sqrt { ax+b } } \) and if the cost of output is zero. Find the total cost as a function of x.
-
Find the area of the region bounded by the curve y2 = 27x3 and the lines x = 0, y = 1 and y = 2.
-
Solve: \(\frac { dy }{ dx } \) = y sin 2x
-
Solve yx2dx + e − xdy = 0
-
Find the rank of the matrix \(\begin{pmatrix} -5 & -7 \\ 5 & 7 \end{pmatrix}\)
-
Evaluate \(\int \sqrt{2 x+1} \ d x\)
-
The demand function of a commodity is y = 36 − x2. Find the consumer’s surplus for y0 = 11
-
Solve the differential equation \(\frac { dy }{ dx } =\frac { x-y }{ x+y } \)
-
From the following table find the missing value
x 2 3 4 5 6 f(x) 45.0 49.2 54.1 - 67.4 -
A pair of dice is thrown 4 times. If getting a doublet is considered a success, find the probability of 2 successes.
-
Fit a trend line by the method of semi-averages for the given data.
Year 1990 1991 1992 1993 1994 1995 1996 1997 Sales 15 11 20 10 15 25 35 30 -
Solve the equations 2x + 3y = 7, 3x + 5y = 9 by Cramer’s rule.
-
Evaluate \(\int _{ \frac { \pi }{ 6 } }^{ \frac { \pi }{ 3 } }{ \sin x } \) dx
-
From the following table find the number of students who obtained marks less than 45.
Marks 30-40 40-50 50-60 60-70 70-80 No. of Students 31 42 51 35 31 -
One fifth percent of the the blades produced by a blade manufacturing factory turn out to be defective. The blades are supplied in packets of 10. Use Poisson distribution to calculate the approximate number of packets containing no defective, one defective and two defective blades respectively in a consignment of 1,00,000 packets (e–0.2 =.9802)
-
The mean weekly sales of soap bars in departmental stores were 146.3 bars per store. After an advertising campaign the mean weekly sales in 400 stores for a typical week increased to 153.7 and showed a standard deviation of 17.2. Was the advertising campaign successful at 95% confidence limit?
-
Construct the cost of living index number for 2011 on the basis of 2007 from the given data using family budget method.
Commodities Price Weights 2007 2011 A 350 400 40 B 175 250 35 C 100 115 15 D 75 105 20 E 60 80 25 -
Solve the following assignment problem.
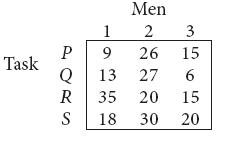
20 x 1 = 20
7 x 2 = 14
7 x 3 = 21
7 x 5 = 35
*****************************************
12th Standard Business Maths and Statistics Videos
TN 12th Business Maths Applications of Matrices and Determinants 50 Important 1 Marks Questions With
TN Class 12th 2024 Business Maths and Statistics Applications of Matrices and Determinants Study Materials TN State Board / Matriculation 12th Business Maths and Statistics Subject - Applications of Matrices and Determinants One Mark Question and Answers






 12th Standard Business Maths and Statistics Syllabus
12th Standard Business Maths and Statistics Syllabus  12th Standard Business Maths and Statistics Study Materials
12th Standard Business Maths and Statistics Study Materials 12th Standard Business Maths and Statistics MCQ Practise Tests
12th Standard Business Maths and Statistics MCQ Practise Tests 

Reviews & Comments about 12th Business Maths - Term II Model Question Paper
Write your Comment