The inverse of f(x) = \(\begin{cases} x\quad if\quad x<1 \\ { x }^{ 2 }\quad if\quad 1\le x\le 4 \\ 8\sqrt { x } \quad if\quad x>4 \end{cases}\) is

(a)

\({ f }^{ -1 }(x)=\begin{cases} x\quad if\quad x<1 \\ \sqrt { x } \quad if\quad 1\le x\le 16 \\ \frac { { x }^{ 2 } }{ 64 } \quad if\quad x>16 \end{cases}\)

(b)

\({ f }^{ -1 }(x)=\begin{cases} -x\quad if\quad x<1 \\ \sqrt { x } \quad if\quad 1\le x\le 16 \\ \frac { { x }^{ 2 } }{ 64 } \quad if\quad x>16 \end{cases}\)

(c)

\({ f }^{ -1 }(x)=\begin{cases} { x }^{ 2 }\quad if\quad x<1 \\ \sqrt { x } \quad if\quad 1\le x\le 16 \\ \frac { { x }^{ 2 } }{ 64 } \quad if\quad x>16 \end{cases}\)

(d)

\({ f }^{ -1 }(x)=\begin{cases} { 2x }\quad if\quad x<1 \\ \sqrt { x } \quad if\quad 1\le x\le 16 \\ \frac { { x }^{ 2 } }{ 8 } \quad if\quad x>16 \end{cases}\)

The shaded region in the adjoining diagram represents.

(a)

A\B

(b)

B\A

(c)

AΔB

(d)

A'

If \(f:[-2,2]\rightarrow A\) is given by f(x) = 3³ then f is onto, if A is ___________

(a)

[3, 3]

(b)

(3, 3)

(c)

[-24,24]

(d)

(-24, 24)

Which one of the following statements is false? The graph of the function \(f(x)={1\over x}\)

(a)

exist is the first and third quadrant only

(b)

is a reciprocal function

(c)

is defined at x = 0

(d)

it is symmetric about y = x and y = - x.

Which one of the following is false?

(a)

A⋂(BΔ\C) = (A ⋂ B)Δ(A ∩ C)

(b)

A∩(B - C) = (A ∩B) \ (A∩C)

(c)

(A U B), = A' ∩ B'

(d)

(A \ B) U B = A ⋂ B

The solution set of the following inequality |x-1| \(\ge\) |x-3| is

(a)

[0, 2]

(b)

\([2,\infty)\)

(c)

(0, 2)

(d)

\((-\infty,2)\)

The number of roots of (x + 3)⁴+ (x + 5)⁴ = 16 is

(a)

4

(b)

2

(c)

3

(d)

0

\(\sqrt [ 4 ]{ 11 } \) is equal to ___________

(a)

\(\sqrt [ 8 ]{ 11^{ 2 } } \)

(b)

\(\sqrt [ 8 ]{ 11^{ 4 } } \)

(c)

\(\sqrt [ 8 ]{ 11^{ 8 } } \)

(d)

\(\sqrt [ 8 ]{ 11^{ 6 } } \)

Let \(\alpha\) and \(\beta\) are the roots of a quadratic equation px² + qx + r = 0 then ___________

(a)

\(\alpha +\beta=-{p\over r}\)

(b)

\(\alpha\beta={p\over r}\)

(c)

\(\alpha +\beta={-q\over p}\)

(d)

\(\alpha \beta =r\)

\(\left( 1+cos\frac { \pi }{ 8 } \right) \left( 1+cos\frac { 3\pi }{ 8 } \right) \left( 1+cos\frac { 5\pi }{ 8 } \right) \left( 1+cos\frac { 7\pi }{ 8 } \right) \) =

(a)

\(\frac { 1 }{ 8 } \)

(b)

\(\frac { 1 }{ 2 } \)

(c)

\(\frac { 1 }{ \sqrt { 3 } } \)

(d)

\(\frac { 1 }{ \sqrt { 2 } } \)

If cosec x + cot x = \(\frac { 11 }{ 2 } \) then tan x = ___________

(a)

\(\frac { 21 }{ 22 } \)

(b)

\(\frac { 15 }{ 16 } \)

(c)

\(\frac { 44 }{ 117 } \)

(d)

\(\frac { 117 }{ 44 } \)

The quadratic equation whose roots are tan 75° and cot 75° is _______________

(a)

x²+4x+ 1 = 0

(b)

4x²-x+ 1 = 0

(c)

4x²+ 4x - 1 = 0

(d)

x² - 4x + 1 = 0

If sin θ = sin \(\alpha\), then the angles θ and \(\alpha\) are related by _______________

(a)

\(\theta=n\pi\pm\alpha\)

(b)

\(\theta=2n\pi+(-1)^n\alpha\)

(c)

\(\alpha=n\pi\pm(-1)^n\theta\)

(d)

\(\theta=(2n+1)\pi+\alpha\)

Area of triangle ABC is _______________

(a)

\(\frac{1}{2}\)ab cos C

(b)

\(\frac{1}{2}\)ab sin C

(c)

\(\frac{1}{2}\)ab cos B

(d)

\(\frac{1}{2}\)bc sin B

The number of five digit telephone numbers having at least one of their digits repeated is

(a)

90000

(b)

10000

(c)

30240

(d)

69760

The number of ways to average the letters of the word CHEESE are _________

(a)

120

(b)

240

(c)

720

(d)

6

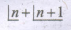is _________

(a)

\(\lfloor{n}(n+2)\)

(b)

(c)

(d)

none of these

A candidate is required to answer 7 question out of 12 questions, which are divided into two groups each containing 6 questions. He is not permitted to attempt more than 5 questions from either group. Find the number of different ways of doing questions.

(a)

779

(b)

781

(c)

780

(d)

782

If ⁿC₁₀ = ⁿC₆, then ⁿC₂ =  _________

(a)

16

(b)

4

(c)

120

(d)

240

The value of the series\(\frac { 1 }{ 2 } +\frac { 7 }{ 4 } +\frac { 13 }{ 8 } +\frac { 19 }{ 16 } +\).....is

(a)

14

(b)

7

(c)

4

(d)

6

The first and last term of an A.P. are 1 and 11. If the sum of its terms is 36, then the number of terms will be ______________

(a)

5

(b)

6

(c)

7

(d)

8

The series for log \(\left( \frac { 1+x }{ 1-x } \right) is\) ______________

(a)

\(x+\frac { { x }^{ 3 } }{ 3 } +\frac { { x }^{ 5 } }{ 5 } +...+\infty \)

(b)

\(2\left[ x+\frac { { x }^{ 3 } }{ 3 } +\frac { { x }^{ 5 } }{ 5 } +...+\infty \right] \)

(c)

\(\frac { { x }^{ 2 } }{ 2 } +\frac { { x }^{ 4 } }{ 4 } +\frac { { x }^{ 6 } }{ 6 } +...+\infty \)

(d)

\(2\left[ \frac { { x }^{ 2 } }{ 2 } +\frac { { x }^{ 4 } }{ 4 } +\frac { { x }^{ 6 } }{ 6 } +...+\infty \right] \)

Expansion of \(log(\sqrt \frac{1+x}{1-x})\) is ______________

(a)

\(x+\frac{x^3}{3}+\frac{x^5}{5}+...\)

(b)

\(1.\frac{x^2}{2}+\frac{x^4}{4}+...\)

(c)

\(1-x+\frac{x^2}{2}+\frac{x^3}{5}+...\)

(d)

\(x-\frac{x^2}{3}+\frac{x^3}{3}+...\)

If an A.P the sum of terms equidistant from the beginning and end is equal to ______________

(a)

first term

(b)

second term

(c)

sum of first and last term

(d)

last term

Which of the following equation is the locus of (at², 2at)

(a)

\(\frac{x^2}{a^2}-\frac{y^2}{b^2}=1\)

(b)

\(\frac{x^2}{a^2}+\frac{y^2}{b^2}=1\)

(c)

x² + y² = a²

(d)

y² = 4ax

If the two straight lines x + (2k -7)y + 3 = 0 and 3kx + 9y - 5 = 0 are perpendicular then the value of k is

(a)

k = 3

(b)

\(k=\frac13\)

(c)

\(k=\frac23\)

(d)

\(k=\frac32\)

Find the point of intersection of the lines 2x²+ xy -y²- 5x + 3y + 2 = 0 ______________

(a)

(-1, -1)

(b)

(1, 1)

(c)

(1, 0)

(d)

(0, 1)

The length of perpendicular from the origin to a line is 12 and the line makes an angle of 120° with the positive direction of y-axis. then the equation of line is ______________

(a)

\(x+y\sqrt 3=24\)

(b)

\(x+y=12\sqrt 2\)

(c)

x + y = 24

(d)

\(x+y=12\sqrt 3\)

The angle between the lines 2x - y + 5 = 0 and 3x + y + 4 = 0 is ______________

(a)

45⁰

(b)

30⁰

(c)

60⁰

(d)

90⁰

The locus of a point which is collinear with the points (a, 0) and (0, b) is ______________

(a)

x + y = 1

(b)

\(\frac{x}{a}+\frac{y}{b}=1\)

(c)

x + y = ab

(d)

\(\frac{x}{a}-\frac{y}{b}=1\)

If \(\triangle\) = \(\begin{vmatrix} a&b &c \\ x & y & z \\ p &q &r \end{vmatrix}\), then \(\begin{vmatrix} ka&kb &kc \\ kx & ky & kz \\k p &kq &kr \end{vmatrix}\) is

(a)

\(\triangle\)

(b)

k\(\triangle\)

(c)

3k\(\triangle\)

(d)

k³\(\triangle\)

A matrix which is not a square matrix is called a_________matrix.

(a)

singular

(b)

non-singular

(c)

non-square

(d)

rectangular

The value of \(\left| \begin{matrix} x+1 & x+2 & x+a \\ x+2 & x+3 & x+b \\ x+3 & x+4 & x+c \end{matrix} \right| \) =_____________ 0, where a, b, c are in AP is 

(a)

(x+1)(x+2)(x+3)

(b)

I

(c)

0

(d)

(x+a)(x+b)(x+c)

The vectors \(\overrightarrow{a}-\overrightarrow{b},\overrightarrow{b}-\overrightarrow{c},\overrightarrow{c}-\overrightarrow{a}\) are

(a)

parallel to each other

(b)

unit vectors

(c)

mutually perpendicular vectors

(d)

coplanar vectors.

\(lim_{\alpha \rightarrow {\pi/4}}{sin \alpha -cos \alpha \over \alpha -{\pi\over 4}}\) is

(a)

\(\sqrt{2}\)

(b)

\(1\over \sqrt{2}\)

(c)

1

(d)

2

\(\lim _{ x\rightarrow \frac { \pi }{ 2 } }{ \frac { \sin { x } }{ x } } =\)

(a)

\(\pi \)

(b)

\(\frac { \pi }{ 2 } \)

(c)

\(\frac { 2 }{ \pi } \)

(d)

1

The slop of the curve \(y={ x }^{ 3 }-7{ x }^{ 2 }+5x+10\) at x=1 is

(a)

6

(b)

-6

(c)

5

(d)

15

If y = cos (sin x²), then \({dy\over dx}\) at x = \(\sqrt{\pi\over 2}\) is

(a)

-2

(b)

2

(c)

\(-2\sqrt{\pi\over 2}\)

(d)

0

The number of points in R in which the function \(f(x)=|x-1|+|x-3|+sin \ x\) is not differentiable, is

(a)

3

(b)

2

(c)

1

(d)

4

 Choose the correct or the most suitable answer from the given four alternatives.
If \(y=\log _{ a }{ x } \) then \(\frac { dy }{ dx } \) is ______

(a)

\(\frac { 1 }{ x } \)

(b)

\(\frac { 1 }{ x\log _{ e }{ a } } \)

(c)

\(\log { _{ e }^{ a } } \)

(d)

\(\frac { 1 }{ \log _{ a }{ x } } \)

Choose the correct or the most suitable answer from the given four alternatives.
If, \(y=a+b{ x }^{ 2 }\) where a, b are arbitrary constants, then ____

(a)

\(\frac { d^{ 2 }y }{ d{ x }^{ 2 } } =2xy\)

(b)

\(x\frac { d^{ 2 }y }{ d{ x }^{ 2 } } ={ y }_{ 1 }\)

(c)

\(x\frac { d^{ 2 }y }{ d{ x }^{ 2 } } -\frac { dy }{ dx } +y=0\)

(d)

\(x\frac { d^{ 2 }y }{ d{ x }^{ 2 } } =2xy\)

\(\int \frac{e^{6 \log x}-e^{5 \log x}}{e^{4 \log x}-e^{3 \log x}} d x\) is

(a)

x+c

(b)

\({x^3\over 3}+c\)

(c)

\({3\over x^3}+c\)

(d)

\({1\over x^2}+c\)

\(\int \sin \sqrt{x} d x\) is

(a)

\(2(-\sqrt{x}cos\sqrt{x}+sin\sqrt{x})+c\)

(b)

\(2(-\sqrt{x}cos\sqrt{x}-sin\sqrt{x})+c\)

(c)

\(2(-\sqrt{x}sin\sqrt{x}-cos\sqrt{x})+c\)

(d)

\(2(-\sqrt{x}sin\sqrt{x}+cos\sqrt{x})+c\)

\(\int { { tan }^{ 3 } } 2sec2x\) dx = ___________+c.

(a)

\(\frac { 1 }{ 6 } \) sec³ 2x

(b)

\(\frac { 1 }{ 6 } \) sec³2x - \(\frac { 1 }{ 2 } \) sec 2x

(c)

\(\frac { 1 }{ 2 } \) sec 2x

(d)

\(\frac { 1 }{ 6 } \) sec³ 2x + \(\frac { 1 }{ 2 } \) sec 2x

\(\int { \frac { x }{ 4+{ x }^{ 4 } } } \) dx is equal to________+c.

(a)

\(\frac { 1 }{ 4 } \) tan^-1 (x²)

(b)

\(\frac { 1 }{ 4 } \) tan^-1 \(\left( \frac { { x }^{ 2 } }{ 2 } \right) \)

(c)

\(\frac { 1 }{ 2 } \) tan^-1 \(\left( \frac { { x }^{ 2 } }{ 2 } \right) \)

(d)

none of these

A man has 3 fifty rupee notes, 4 hundred rupees notes, and 6 five hundred rupees notes in his pocket. If 2 notes are taken at random, what are the odds in favour of both notes being of hundred rupee denomination?

(a)

1:12

(b)

12:1

(c)

13:1

(d)

1:13

If A and B are two events such that A ⊂ B and P(B)\(\neq o\), then which of the following is correct?

(a)

\(P(A/B)={P(A)\over P(B)}\)

(b)

P(A/B)

(c)

P(A/B)\(\ge\)P(A)

(d)

P(A/B)>P(B)

If m is a number such that m \(\le\) 5, then the probability that quadratic equation 2x² + 2mx + m + 1 = 0 has real roots is

(a)

\({1\over 5}\)

(b)

\({2\over 5}\)

(c)

\({3\over 5}\)

(d)

\({4\over 5}\)

A bag contains 5 black balls, 4 white balls and 3 red balls. If a ball is selected at random, the probability that it is black or red ball is

(a)

\(\frac { 1 }{ 3 } \)

(b)

\(\frac { 1 }{ 4 } \)

(c)

\(\frac { 5 }{ 12 } \)

(d)

\(\frac { 2 }{ 3 } \)

A flash light has 8 batteries out of which 3 are dead. If 2 batteries are selected without replacement and tested, the probability that both are dead is

(a)

\(\frac { 3 }{ 28 } \)

(b)

\(\frac { 1 }{ 14 } \)

(c)

\(\frac { 9 }{ 64 } \)

(d)

\(\frac { 33 }{ 56 } \)