12th Standard CBSE Physics Current Electricity Important Questions
By QB365
12th Standard CBSE Physics Current Electricity Important Questions
QB365 - Question Bank Software
Current Electricity Important Questions
12th Standard CBSE
-
Reg.No. :
Physics
-
At a particular point, electric field depends upon
(a)Source charge Q only
(b)test charge qo only
(c)both Q and q0
(d)neither Q nor qo
-
The SI unit of electric field intensity is
(a)N
(b)N/C
(c)C/m2
(d)N/m2
-
Electric field due to a single charge is
(a)asymmetric
(b)cylindrically symmetric
(c)spherically symmetric
(d)None of the above
-
Electric dipole moment is
(a)scalar
(b)neither scalar vector
(c)a vector directed from -q to +q
(d)a vector directed from +q to -q
-
Electric field intensity (E) due to an electric dipole varies with distance (r) of the point from the centre of dipole as:
(a)\(E\alpha {1\over r}\)
(b)\(E\alpha{1\over r^4}\)
(c)\(E\alpha{1\over r^2}\)
(d)\(E\alpha {1\over r^3}\)
-
At a given distance from the centre of electic dipole, field intensity on axial line is k times the field intensity on equatorial line, where K =
(a)2
(b)3
(c)4
(d)1
-
Potential energy of an electric dipole held at an angle \(\theta\) in a uniform electric field is zero when \(\theta=\)
(a)\(0^o\)
(b)90o
(c)180o
(d)360o
-
Force \(\overrightarrow { F } \) acting on a test charge qo in a uniform electric field \(\overrightarrow { E } \) is
(a)\(\overrightarrow { F } =q_{ o }\overrightarrow { E } \)
(b)\(\overrightarrow { F } =\frac { \overrightarrow { E } }{ q_{ o } } \)
(c)\(\overrightarrow { F } =\frac { \overrightarrow { q_o } }{\overrightarrow { E } } \)
(d)\(\overrightarrow { F } =q_{ o }^{ 2 }\overrightarrow { E } \)
-
Electrostatic potential V at a point, distant r from a charge q varies as
(a)q/r2
(b)q2/r
(c)q/r
(d)q2/r2
-
Work done in carrying an electron from A to B lying on an equipotential surface of one volt potential is
(a)1 eV
(b)10 eV
(c)1 volt
(d)Zero
-
Twenty million electrons reaches from point X to point Y in two micro second as shown in the figure. Direction and magnitude of the current is
 (a)
(a)1.5 x 10-10 A from X to Y
(b)1.6 x 10-6 A from Y to X
(c)1.5 x 10-13 A from Y to X
(d)1.6 x 10-4 A from X to Y
-
A resistor has a colour code of green, blue, brown, and silver. What is its resistance?
(a)5600 Ω ± 10%
(b)560 Ω ± 5%
(c)560 Ω ± 10%
(d)56 Ω ± 5%
-
Kirchhoff's current law is consequence of conservation of
(a)energy
(b)momentum
(c)charge
(d)mass
-
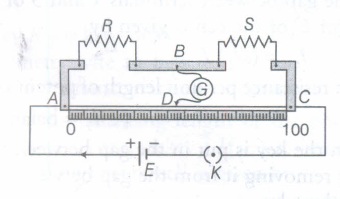
In a meter bridge, the null point is found at a distance of 33.7 cm from A. If a resistance of 12\(\Omega \) is connected in parallel with S, the null point occurs at 51.9 cm. Determine the values of R and S.
 (a)
(a) -
A negligibly small current is passed through a wire length 15 m and uniform cross-section \(6.0\times { 10 }^{ -7 }m^{ 2 }\) and its resistance is measured to be \(5.0\Omega \). What is the resistivity of the material at the temperature of the experiment?
(a) -
A conductor of length L is connected to a dc source of emf \(\epsilon\). If this conductor is replaced by another conductor of the same material and same area of cross-section but of length 3 L, how will the draft velocity change?
(a) -
If the current flowing in a copper wire be allowed to flow in another copper wire of the same length but of double the radius, then what will be the effect on the drift velocity of the electrons. If the same current is allowed to flow in an iron wire of the same thickness, then?
(a) -
An electric current is passed through a circuit containing two wires of the same material connected in parallel. If the length and radii of the wires are in the ratio 2/3 and 4/3, then find the ratio of the currents passing through the wires.
(a) -
At 0oC, the resistance of a conductor B is n times that of conductor A. The temperature coefficients of resistance of A and B are \(\alpha_1\) and \(\alpha_2\) respectively. For the series combination of the two conductors find (a) the resistance at 0oC (b) the temperature coefficient of resistance.
(a) -
What is terminal potential difference of a cell? Can its value be greater than the emf of a cell? Explain.
(a) -
A car has a fresh storage battery of emf 12 V and internal resistance \(5.0 \times 10^{-2} \ \Omega\). If the starter motor draws a current of 90 A, what is the terminal voltage of the battery when the starter is on?
(a) -
What is the difference between heating wire and fuse wire?
(a) -
An immersion heater is rated 836 watt. In what time, it should heat 1 litre of water from 20oC to 40o C? J = 4.18 J/cal.
(a) -
A cell of emf 'E' and internal resistance 'r' is connected across a variable resistor 'R'. Plot a graph showing variation of terminal voltage 'V' of the cell versus the current 'I'. Using the plot, show how the emf of the cell and its internal resistance can be determined.
Draw the plots of the terminal voltage V versus (i) R and (ii) the current I(a) -
Estimate the average drift speed of conduction electrons in a copper wire of cross-sectional area 1.0 x10-7 m2 carrying a current of 1.5 A. Assume the density of conduction electrons to be 9 x 1028 m-3.
(a) -
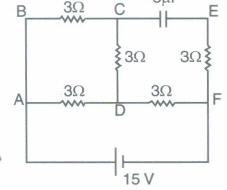
In the circuit shown in the figure, find the total resistance of the circuit an d the current in the arm CD.
 (a)
(a) -
According to Ohm's law, the current flowing through a conductor is directly proportional to the potential difference across the ends of the conductor i.e \(I \propto V \Rightarrow \frac{V}{I}=R\) where R is resistance of the conductor Electrical resistance of a conductor is the obstruction posed by the conductor to the flow of electric current through it. It depends upon length, area of cross-section, nature of material and temperature of the conductor We can write \(R \propto \frac{l}{A} \text { or } R=\rho \frac{l}{A}\) where \(\rho\) is electrical resistivity of the material of the conductor.
(i) Dimensions of electric resistance is\(\text { (a) }\left[\mathrm{ML}^{2} \mathrm{~T}^{-2} \mathrm{~A}^{-2}\right]\) \(\text { (b) }\left[M L^{2} T^{-3} A^{-2}\right]\) \(\text { (c) }\left[\mathrm{M}^{-1} \mathrm{~L}^{-2} \mathrm{~T}^{-1} \mathrm{~A}\right]\) \(\text { (d) }\left[M^{-1} L^{2} T^{2} A^{-1}\right]\) (ii) If \(1 \mu \mathrm{A}\) current flows through a conductor when potential difference of2 volt is applied across its ends, then the resistance of the conductor is
\(\text { (a) } 2 \times 10^{6} \Omega\) \(\text { (b) } 3 \times 10^{5} \Omega\) \(\text { (c) } 1.5 \times 10^{5} \Omega\) \(\text { (d) } 5 \times 10^{7} \Omega\) (iii) Specific resistance of a wire depends upon
(a) length (b) cross-sectional area (c) mass (d) none of these (iv) The slope of the graph between potential difference and current through a conductor is
(a) a straight line (b) curve (c) first curve then straight line (d) first straight line then curve (v) The resistivity of the material of a wire 1.0 m long, 0.4 mm in diameter and having a resistance of 2.0 ohm is
\(\text { (a) } 1.57 \times 10^{-6} \Omega \mathrm{m}\) \(\text { (b) } 5.25 \times 10^{-7} \Omega \mathrm{m}\) \(\text { (c) } 7.12 \times 10^{-5} \Omega \mathrm{m}\) \(\text { (d) } 2.55 \times 10^{-7} \Omega \mathrm{m}\) (a) -
Metals have a large number of free electrons nearly 1028 per cubic metre. In the absence of electric field, average terminal speed of the electrons in random motion at room temperature is of the order of 105 m s-1 When a potential difference V is applied across the two ends of a given conductor, the free electrons in the conductor experiences a force and are accelerated towards the positive end of the conductor. On their way, they suffer frequent collisions with the ions/atoms of the conductor and lose their gained kinetic energy. After each collision, the free electrons are again accelerated due to electric field, towards the positive end of the conductor and lose their gained kinetic energy in the next collision with the ions/atoms of the conductor. The average speed of the free electrons with which they drift towards the positive end of the conductor under the effect of applied electric field is called drift speed of the electrons.
(i) Magnitude of drift velocity per unit electric field is(a) current density (b) current (c) resistivity (d) mobility (ii) The drift speed of the electrons depends on
(a) dimensions of the conductor (b) number density of free electrons in the conductor (c) both (a) and (b) (d) neither (a) nor (b) (iii) We are able to obtain fairly large currents in a conductor because
(a) the electron drift speed is usually very large (b) the number density of free electrons is very high and this can compensate for the low values of the 6 electron drift speed and he very small magnitude of the electron charge (c) the number density of free electrons as well as the electron drift speeds are very large and these compensate for the very small magnitude of the electron charge (d) the very small magnitude of the electron charge has to be divided by the still smaller product of the number density and drift speed to get the electric current (iv) Drift speed of electrons in a conductor is very small i.e., i = 10-4 m s-1. The Electric bulb glows immediately. When the switch is closed because
(a) drift velocity of electron increases when switch is closed (b) electrons are accelerated towards the negative end of the conductor (c) the drifting of electrons takes place at the entire length of the conductor (d) the electrons of conductor move towards the positive end and protons of conductor move towards negative end of the conductor (v) The number density offree electrons in a copper conductor is 8.5 x 1028 m-3. How long does an electron take to drift from one end of a wire 3.0 m long to its other end? The area of cross-section of the wire is 2.0 x 10-6m2 and it is carrying a current of 3.0 A.
(a) 8.1 x 104 s (b) 2.7 x 104 s (c) 9 x 103 s (d) 3 x 103 S (a) -
A single cell provides a feeble current. In order to get a higher current in a circuit, we often use a combination of cells A combination of cells is called a battery, Cells can be joined in series, parallel or in a mixed way.
Two cells are said to be connected in series when negative terminal of one cell is connected to positive terminal of the other cell and so on. Two cells are said to be connected in parallel if positive terminal of each cell is connected to one point and negative terminal of each cell connected to the other point. In mixed grouping of cells, a certains number of identical cells are joined in series, and all such rows are then connected in parallel with each other.
(i) To draw the maximum current from a combination of cells, how should the cells be grouped?(a) Parallel (b) Series (c) Mixed grouping (d) Depends upon the relative values of internal and external resistances (ii) The total emf of the cells when n identical cells each of emf e are connected in parallel is
\(\text { (a) } n \varepsilon\) \(\text { (b) } n^{2} \varepsilon\) (c) E \(\text { d) } \frac{\varepsilon}{n}\) (iii) 4 cells each of emf 2 V and internal resistance of \(1 \Omega\) are connected in parallel to a load resistor of \(2 \Omega\). Then the current through the load resistor is
(a) 2 A (b) 1.5 A (c) 1 A (d) 0.888 A (iv) If two cells out of n number of cells each of internal resistance 'r' are wrongly connected in series, then total resistance of the cell is
(a) 2nr (b) nr - 4r (c) nr (d) r (v) Two identical non-ideal batteries are connected in parallel. Consider the following statements.
(i). The equivalent emf is smaller than either of the two emfs.
(ii) The equivalent internal resistance is smaller than either of the two internal resistances(a) Both (i) and (ii) are correct. (b) (i) is correct but (ii) is wrong (c) (ii) is correct but (i) is wrong. (d) Both (i) and (ii) are wrong. (a) -
In 1942, a German physicist Kirchhoff extended Ohm's law to complicated circuits and gave two laws, which enable us to determine current in any part of such a circuit. According to Kirchhoff's first rule, the algebraic sum of the currents meeting at a junction in a closed electric circuit is zero. The current flowing in a conductor towards the junction is taken as positive and the current flowing away from the junction is taken as negative. According to Kirchhoff's second rule, in a closed loop, the algebraic sum of the emf's and algebraic sum of the products of current and resistance in the various arms of the loop is zero. While traversing a loop, if negative pole of the cell is encountered first, then its emf is negative, otherwise positive.
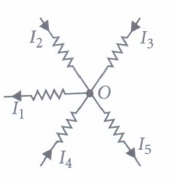
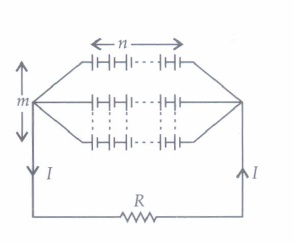
(i) Kirchhoff's Ist law follows(a) law of conservation of energy (b) law of conservation of charge (c) law of conservation of momentum (d) Newton's third law of motion (ii) The value of current I in the given circuit is
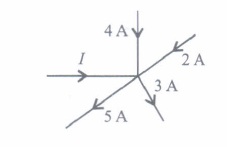
(a) 4.5 A (b) 3.7 A (c) 2.0 A (d) 2.5 A (iii) Kirchhoff's IInd law is based on
(a) law of conservation of momentum of electron (b) law of conservation of charge and energy (c) law of conservation of energy (d) none of these. (iv) Point out the right statements about the validity of Kirchhoff's Junction rule.
(a) The current flowing towards the junction are taken as positive. (b) The currents flowing away from the junction are taken as negative. (c) bending or reorienting the wire does not change the validity of Kirchhoff's Junction rule (d) All ofthe above (v) Potential difference between A and B in the circuit shown here is
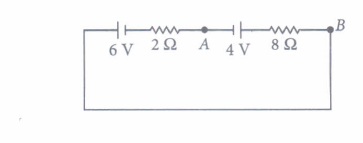
(a) 4 V (b) 5.6 V (c) 2.8 V (d) 6 V (a)