Class 11th Applied Mathematics - Calculus Case Study Questions and Answers 2022 - 2023
By QB365
QB365 provides a detailed and simple solution for every Possible Case Study Questions in Class 11 Applied Mathematics Subject - Calculus, CBSE. It will help Students to get more practice questions, Students can Practice these question papers in addition to score best marks.
QB365 - Question Bank Software
Calculus Case Study Questions With Answer Key
11th Standard CBSE
-
Reg.No. :
Applied Mathematics
-
On the basis of the following graph of function f answer the questions below:
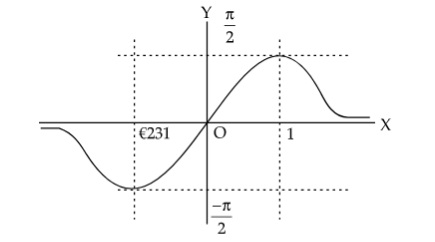
(i) Domain of the function f is(a) (–∞, 0) (b) (– 1,∞) (c) (–∞,∞) (d) (-1,1) (ii) Range of the function f is
(a) \(\left[-\frac{\pi}{2}, \frac{\pi}{4}\right]\) (b) \(\left[-\frac{\pi}{2}, \frac{\pi}{2}\right]\) (c) \(\left[-\frac{\pi}{6}, \frac{\pi}{2}\right]\) (d) \(\left[-\frac{\pi}{2}, \frac{\pi}{4}\right]\) (iii) Function is continuous for all x where x belongs to
(a) (–∞, 0) (b) (–∞, 1) (c) (-1,1) (d) (–∞,∞) (iv) Function is increasing in
(a) (–1, 0) (b) (–2, 1) (c) (0, 1) (d) (–1, 1) (v) Function is decreasing in
(a) (–∞, -1) (b) (–∞, 1) ∪(0,∞) (c) (–∞, 0)∪(0,∞) (d) (–∞, –1)∪(1,∞) (a) -
The graph of the polynomial function (f), graph answer the questions given below:
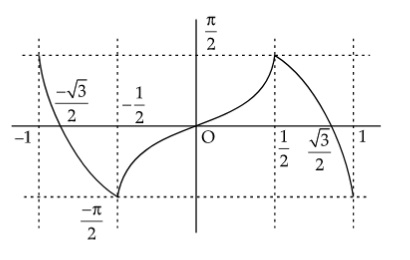
(i) The domain of the given function f is R and the range of the function f is(a) \(\left(-\frac{\pi}{2}, \frac{\pi}{2}\right)\) (b) (– 1,0) (c) (1,0) (d) \(\left(-\frac{\pi}{4}, \frac{\pi}{4}\right)\) (ii) Function is continuous for all x∈R and hence the function is differentiable for
(a) \(x \in \mathbf{R}^{+}-\{-1,1\}\) (b) \(x \in \mathbf{R}^{-}-\{-1,1\}\) (c) \(x \in \mathbf{R}-\{-0,1\}\) (d) \(x \in \mathbf{R}-\{-1,1\}\) (iii) The function is increasing in
(a) \(\left(-\frac{1}{2}, \frac{1}{2}\right)\) (b) \(\left(-\frac{1}{2}, \frac{1}{3}\right)\) (c) \(\left(\frac{1}{2}, \frac{3}{2}\right)\) (d) \(\left(-\frac{1}{2}, 1\right)\) (iv) Function is decreasing in
(a) \((-1,0) \cup\left(\frac{1}{2}, 1\right)\) (b) \(\left(-1,-\frac{1}{3}\right) \cup\left(\frac{1}{2}, 1\right)\) (c) \(\left(-1,-\frac{1}{2}\right) \cup\left(\frac{1}{5}, 1\right)\) (d) \(\left(-1,-\frac{1}{2}\right) \cup\left(\frac{1}{2}, 1\right)\) (v) Function is continuous from
(a) (0,1) (b) (– 1,1) (c) (–1,0) (d) (-1,2) (a) -
In a class of XI standard, Mathematics teacher was explaining limits to students. In case of existence of limit if left hand and right-hand limits are equal and both exist then limit exists. It is also shown below.
Left-hand limit is \(f(a-0)=\lim _{x \rightarrow a^{-}} f(x)=\lim _{h \rightarrow 0} f(a-h)\)
Similarly, right hand limit is \(f(a+0)=\lim _{x \rightarrow a^{+}} f(x)=\lim _{h \rightarrow 0} f(a+h)\)
\(\text { Existence of Limit: } \lim _{x \rightarrow a} f(x) \text { exists, if }\)
\(\text { (i) } \lim _{x \rightarrow a^{-}} f(x) \text { and } \lim _{x \rightarrow a^{+}} f(x) \text { both exist }\)
\(\text { (ii) } \lim _{x \rightarrow a^{-}} f(x)=\lim _{x \rightarrow a^{+}} f(x)\)
On the basis of this information teacher ask students various questions as mentioned below:
(i) Evaluate the left-hand limit of the given function
\(\left\{\begin{array}{l} 1+x^{2} ; 0 \leq x \leq 1 \\ 2-x^{+} x>1 \end{array} \text { at } x=1\right.\)(a) 1 (b) 2 (c) 3 (d) 4 (ii) Evaluate the right-hand limit of the given function
\(\left\{\begin{array}{l} 1+x^{2} ; 0 \leq x \leq 1 \\ 2-x ; x>1 \end{array} \text { at } x=1\right.\)(a) 1 (b) 2 (c) 3 (d) 4 (iii) \(\text { Let } f(x)=\left\{\begin{array}{l} \cos x ; x>0 \\ x+k ; x<0 \end{array}\right.\) If limit exist find k.
(a) 2 (b) -1 (c) 0 (d) 1 (iv) Which of the following graph is continuous
(v) In order for a function to be continuous at a point which of the following must be true.
(a) The limit must exist at that point (b) The function must be defined at that point (c) The limit and the function must have equal values at that point (d) All the above
(a) -
Reena and Lilly, student of class XI, were discussing on the topic 'differentiability'. They prepared the note of their discussion which are as follows:
\(f^{\prime}\left(x_{0}\right)=\lim _{\Delta x \rightarrow 0} \frac{f\left(x_{0}+\Delta x\right)-f\left(x_{0}\right)}{\Delta x} \text { exist if and only }\) \(\text { both } f^{\prime}\left(x_{0}^{-}\right)=\lim _{\Delta x \rightarrow 0^{-}} \frac{f\left(x_{0}+\Delta x\right)-f\left(x_{0}\right)}{\Delta x} \text { and }\)
\(\begin{aligned} &f^{\prime}\left(x_{0}^{+}\right)=\lim _{\Delta x \rightarrow 0^{+}} \frac{f\left(x_{0}+\Delta x\right)-f\left(x_{0}\right)}{\Delta x} \text { exist and } f^{\prime}\left(x_{0}^{-}\right)=f^{\prime}\left(x_{0}^{+}\right) . \end{aligned}\)
Therefore, f '(x0) \(=\lim _{\Delta x \rightarrow 0} \frac{f\left(x_{0}+\Delta x\right)-f\left(x_{0}\right)}{\Delta x}\)
if and only if \(f^{\prime}\left(x_{0}^{-}\right)=f^{\prime}\left(x_{0}^{+}\right)\)
If any one of the condition fails, then f is not differ entiable at x0.
\(\begin{aligned} &f^{\prime}\left(x_{0}^{+}\right)=\lim _{k \rightarrow 0} \frac{f\left(x_{0}+h\right)-f\left(x_{0}\right)}{h} \text {and}\ f^{\prime}\left(x_{0}^{-}\right)=\lim _{k \rightarrow 0} \frac{f\left(x_{0}-h\right)-f\left(x_{0}\right)}{h} . \end{aligned}\)
On the basis of this information answer the following questions:
(i) If left hand derivative is not equal to right hand derivative then function is(a) not differentiable (b) differentiable (c) data insufficient (d) none of these (ii) If a function is continuous, then it is differentiable. This statement is
(a) True (b) False (c) can’t say (d) None of these (iii) If a function is differentiable then it is continuous. This statement is
(a) True (b) False (c) can’t say (d) None of these (iv) Differentiation of \(\left(\sqrt{x}+\frac{1}{\sqrt{x}}\right)^{2} \text { is }\)
\(\text { (a) } 3+\frac{1}{x^{2}}\) \(\text { (b) } 1+\frac{1}{x^{2}}\) \(\text { (c) } 1-\frac{1}{x^{2}}\) \(\text { (d) } 1-\frac{1}{2 x^{2}}\) (v) f (x) = | x | is continuous function and limit exists. This statement is
(a) True (b) False (c) Data insufficient (d) None of these (a) -
Riya is studying in class XI. Today her brother Mayank is teaching her the topic of Maths ‘Tangent and Normal’. Mayank prepared the following notes on ‘Tangent and Normal’ for Riya.
(i) Slope of gradient of a line: If a line makes an angle q is called the slope of gradient of the line.
(ii) Pictorial representation of tangent & normal:
(iii) Facts about the slope of aline:
(a) If a line is parallel to the x-axis (or perpendicular to y-axis), then its slope is 0 (Zero).
(b) If a line is parallel to the y-axis (or perpendicular x-axis), then its slope is \(\frac{1}{0}\) i.e., not defined.
(c) If two lines are perpendicular, then product of their slope equals – 1 i.e., m1 × m2 =– 1. Whereas, for two parallel lines, their slopes are equal i.e., m1 = m2. (Here in both the cases, m1 and m2 represent the slope of the respective line.
(iv)Equation of Tangent at (x1, y1):(y – y1) = mT(x– x1), where mT is the slope of normal such that mT \(=\left[\frac{d y}{d x}\right]_{\mathrm{at}\left(x_{1}, y_{1}\right)}\)
(v)Equation of Normal at (x1, y1):(y – y1) = mN(x– x1), where mN is the slope of normal such that mN \(=\frac{-1}{\left[\left.\frac{d y}{d x}\right|_{\mathrm{at}\left(x_{1}, y_{1}\right)}\right.}\)
(vi) Acute angle between the two curves whose slope m1 and m2 are known \(\tan \theta=\left|\frac{m_{2}-m_{1}}{1+m_{1} \cdot m_{2}}\right| \text { or } \tan ^{-1}\left|\frac{m_{2}-m_{1}}{1+m_{1} \cdot m_{2}}\right|\).
(vii) Finding the slope of a line ax + by + c = 0STEP 1: Express the given line in the standard intercept form y = mx + c i.e., y = \(\left(-\frac{a}{b}\right) x-\frac{c}{b}\)
STEP 2: By comparing to the standard form y = mx + c , we can conclude \(-\frac{a}{b}\) is the slope of given line ax + by + c = 0 .On the basis of the above information answer the following questions:
(i) What is the equation of the tangent at a specific point of y2 = 4 ax at (0, 0) ?(a )x = 0 (b) x = 1 (c) x = 2 (d) x = 3 (ii) If X and Y are given as current co-ordinates, what is the equation of the tangent at a specific point of x3 – 3a xy + y3 = 0 at (x, y)?
(a) (x2 – ay) X + (y2 – ax) Y = – 2axy (b) (x2 – ay) X + (y2 – ax) Y = 2axy (c) (x2 – ay) X + (y2 – ax) Y = axy (d) (x2 – ay) X + (y2 – ax) Y = – ax (iii) Find the slope of the normal to the curve y = 4x2 – 14 x + 5 at x = 5.
(a) \(-\frac{1}{26}\) (b) \(\frac{1}{26}\) (c) 26 (d) -26 (iv) Find the tangent to the curve y = 5x4 – 3x2 + 2x – 1 at x = 1.
(a) 15 (b) 14 (c) 16 (d) 17 (v) Find the equation of all the lines having slope 0 which are tangent to the curve y = 6x2– 7x.
(a) \(\frac{24}{49}\) (b) \(-\frac{24}{49}\) (c) \(\frac{49}{24}\) (d) \(-\frac{49}{24}\) (a) -
The shape of a toy is given as f(x) = 6(2x4 – x2). To make the toy beautiful 2 sticks which are perpendicular to each other were placed at a point (2, 3), above the toy.
(i) What is slope of tangent at (2, 3)?
(a) 360 (b) -360 (c) \(\frac{1}{360}\) (d) \(-\frac{1}{360}\) (ii) Find the equation of tangent if it passes through (2, 3)?
(a) x + 360y = 1082 (b) y = 360 x – 717 (c) x + 717y = 360 (d) none (iii) Find the slope of the normal based on the position of the stick.
(a) 360 (b) -360 (c) \(\frac{1}{360}\) (d) \(\frac{-1}{360}\) (iv) Find the equation of normal if it passes through (2, 3)?
(a) x + 360 y – 1082 = 0 (b) 360 x + y – 1082 = 0 (c) x + 360 y + 1082 = 0 (d) 360 x + y + 1082 = 0 (v) Find is slope of tangent at (3, 2)?
(a) 1260 (b) -1260 (c) \(\frac{1}{1260}\) (d) \(-\frac{1}{1260}\) (a)
Case Study
*****************************************
Answers