CBSE 10th Standard Maths Subject Areas Related to Circles HOT Questions 2 Mark Questions With Solution 2021
By QB365 on 29 May, 2021
QB365 Provides the HOT Question Papers for Class 10 Maths, and also provide the detail solution for each and every HOT Questions. HOT Questions will help to get more idea about question pattern in every exams and also will help to get more marks in Exams
QB365 - Question Bank Software
CBSE 10th Standard Maths Subject Areas Related to Circles HOT Questions 2 Mark Questions With Solution 2021
10th Standard CBSE
-
Reg.No. :
Maths
-
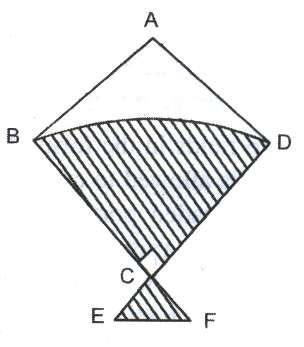
The figure given shows a kite, in which BCD is in the shape of a quadrant of a circle of radius 42 cm. ABCD is a square and \(\triangle CEF\) is an isosceles right-angled triangle whose equal sides are 6 cm long. Find the area of the shaded region.
 (a)
(a) -
Four cows are tethered at the four corner of a squares plot of size 50 m. So that they just cannot reach one another. What area will be left ungrazed?
(a) -
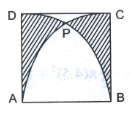
In the adjoining figure, ABCD is a square of side 6cm.Find the area of the shaded region.
 (a)
(a) -
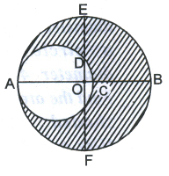
In the figure alongside, crescent is formed by two circles which touch at the point A, O is the centre of the point A, O is the centre of the bigger circle.If CB=9cm and ED=5cm, find the area of the shaded region.[Take \(\pi\)=3.14]
 (a)
(a) -
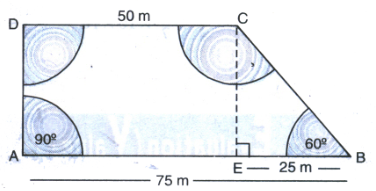
ABCD is a field in the shape of a trapezium.AB||DC and \(\angle\)ABC=600,\(\angle\)DAB=900.Four sector are formed with centres A, B, C and D.THe radius of each sector is 17.5m.Find:
(i)the total area of the four sectors
(ii)the area of remaining portion, given that AB=75m and CD=50m.
 (a)
(a)
*****************************************
CBSE 10th Standard Maths Subject Areas Related to Circles HOT Questions 2 Mark Questions With Solution 2021 Answer Keys
-
1404 cm2
-
535.71 cm2
-
From P, draw PQ⊥AB, join PA and PB,
In ΔAPQ and Δ BPQ, we have
AP = BP = 6cm
PQ = PQ
ㄥAQP = ㄥBQP=900
⇒ ΔAPQ ~ Δ BPQ
Therefore, \(AQ = BQ={1\over 2}AB\)
\(⇒\ AQ=BQ=3cm\)
Let ㄥPBQ=θ
-S.png)
∴ In rt. Δ QBP, ㄥQ=900
\(⇒\ cos\theta={QB\over PB}\)
\(cos\theta={3\over 6}={1\over 2}\)
⇒ cos θ = cos600
⇒ θ=600
Also, \({PQ\over PB}=sin60^0\)
\(PQ=6\times{\sqrt3\over2}=5.196cm...(i)\)
Now, area of sector BPA=\({60^0\over360^0}\times{22\over 7}\times6\times6\)
=18.857cm2
Area of the portion APB
= 2 X [Area of sector BPA - Area of ΔBPQ]
= 2 x [18.857 - 7.7941= 22.126cm2
Area of the shaded portion = 2 x [Area of quadrant ABC — Area of the portion APB]
\(=2\times\left[{90^0\over 360^0}\times{22\over 7}\times6\times6-22.126\right]\)
=2 x [28.286-22.126]
=12.32cm2
Hence, the required area of the shaded portion is 12.32cm2 -
Suppose R and r be the radii of bigger and smaller circles, respectively
⇒ AB - AC = CB
⇒ 2R-2r=9
⇒ R-r=\(9\over 2\)= 4.5 cm ...(i)
-S.png)
Join AD and CD
ΔAOD ~ ΔDOC
\(⇒\ {OD\over OA}={OC\over OD}\)
⇒ OD2 = OA x OC
⇒ (R-5)2=R x (R-9)
⇒R2+25-10R=R2-9R
⇒ R=25cm
From (i), we have R-r = 4.5
=25 - 4.5 = 20.5cm
Now, area of the shaded portion = πR2-πr2
=π(R2-r2)
=π(R+r)(R-r)
=3.14 x (25+20.5)(25-20.5)
=3.14 x 45.5 x 4.5
= 642.915 cm2
Hence, the required area of the shaded portion is 642.915cm2 -
Let ABCD be a trapezium with AB 75 m,
DC = 50 m, ㄥABC = 600 and ㄥDAB = 900.
Draw CE ⊥AB.
∴ AE = 50m and EB = 75-50 = 25m
We know that sum of angles of a trapezium is 3600.
∴ Area of four sectors = πr2
\(={22\over 7}\times17.5\times17.5\)
=962.5m2
In rt .ㄥed ΔCEB,
\({EC\over EB}=tan60^0\)
\(⇒ {EC\over 25}=\sqrt{3}\)
⇒ EC=25√3m
Now, area of trapezium\(={1\over 2}(AB+DC)\times EC\)
\(={1\over 2}(75+50)\times25\sqrt3\)
\(={1\over 2}\times125\times25\sqrt3\)
=2706.25
Area of remaining portion = 2706.25 - 962.5
=1743.75m2

























