CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions 2021 Part - II
By QB365 on 29 May, 2021
QB365 Provides the HOT Question Papers for Class 10 Maths, and also provide the detail solution for each and every HOT Questions. HOT Questions will help to get more idea about question pattern in every exams and also will help to get more marks in Exams
QB365 - Question Bank Software
CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions 2021 Part - II
10th Standard CBSE
-
Reg.No. :
Maths
-
If the sum of the first n terms of an A.P. is given by 3n2+5n, find the common difference of the A.P.
(a) -
A man on the deck of a ship is 10m above water level.He observes that the angle of elevation of the top of a hill is 600 and the angle of depression of the base of the hill is 300.Calculate the distance of the hill from the ship and the height of the hill.
(a) -
A bird is sitting on the top of a tree which is 60m high.The angle of elevation of the bird from a point on the ground is 450.the bird flies away from the point o observation horizontally and remains at a constant height.After 2seconds, the angle of elevation of the bird from the point of observation becomes 300 .Find the speed of flying of bird.
(a) -
In the given figure, AB is a diameter of the circle, with centre O and AT is a tangent. Calculate the numerical value of x.
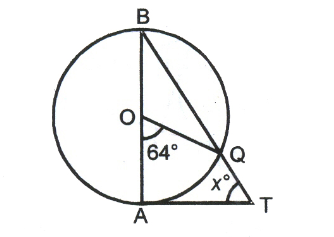 (a)
(a) -
Draw an equilateral triangle of altitude 4 cm. Construct another triangle similar to it such that its sides are \(\frac { 2 }{ 3 } \) of the given triangle.
(a) -
Draw a circle of diameter 8 cm. From a point P, 7 cm away from its centre, construct a pair of tangents to the circle. Measure the lengths of the tangent segments.
(a) -
Find the area of the shaded region of the figure along side.
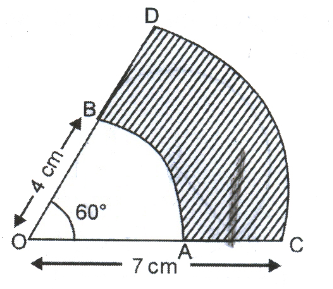 (a)
(a) -
ABCD is a field in the shape of a trapezium.AB||DC and \(\angle\)ABC=600,\(\angle\)DAB=900.Four sector are formed with centres A, B, C and D.THe radius of each sector is 17.5m.Find:
(i)the total area of the four sectors
(ii)the area of remaining portion, given that AB=75m and CD=50m.
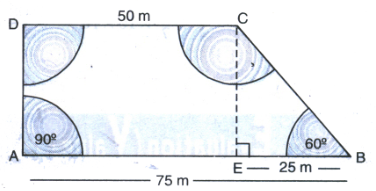 (a)
(a) -
A vessel is in form of an inverted cone.Its height is 8cm and the radius of its top, which is open, is 5cm.It is filled with water up to the brim.When lead shots, each of which is a sphere of radius 0.5cm are dropped into the vessel, one-fourth of the water flows out.Find the number of lead shots dropped in the vessel.
(a) -
In a bag-A, there are four cards numbered 1,3,5 and 7 respectively.In another bag-B, there are three cards numbered 2, 4 and 6 respectively.A card is drawn at random from each bag.
(i)Write the possible outcomes i.e., sample space
(ii)Find the probability that the sum of these two cards drawn is:
(a)7
(b)even
(c)odd
(d)more than 7(a)
*****************************************
CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions 2021 Part - II Answer Keys
-
Since the sum of the first n terms of an A.P. is given as 3n2 + 5n
\(\therefore\) Sn = 3n2 + 5n ....(i)
\(\therefore\) Sn-1 = 3( n - 1)2 + 5 ( n - 1 )
= 3( n2 + 1 - 2n ) + 5( n - 1 )
= 3n2 + 3 - 6n + 5n - 5
= 3n2 - n - 2 ...(ii)
Now, an = Sn - Sn-1
= 3n2 + 5n - 3n2 + n + 2
an = 6n + 2
Put n = 1 and n = 2, we have
a1 = 8 and a2 = 14
Hence, the common difference is 6. -
17.32m, 40m
-
21.96m/s
-
\(x={ 58 }^{ \circ }\)
-
Steps of Construction :
1. Draw any line l and take any point X on it.
2. At X, construct a right angle.
3. With X as centre and of radius 4 cm, draw an arc intersecting XY in A.
4. At A and initial line AX, draw angle of 30o to each sides and let these lines intersect line I in B and C.
5. Join AB and AC to get the given triangle.
6. At B, construct an acute angle ㄥCBZ (<90o).
7. Mark three points on BZ such that BB1= B1B2 = B2B3.
8. Join B3C.
9. Through B2, draw B2C' || B3C, intersecting line I in C'.
10. Through C', draw C'A' || CA, intersecting BA in A'. Thus, ΔA'BC' is the required triangle. -
5.7 cm
-
17.29 cm2
-
Let ABCD be a trapezium with AB 75 m,
DC = 50 m, ㄥABC = 600 and ㄥDAB = 900.
Draw CE ⊥AB.
∴ AE = 50m and EB = 75-50 = 25m
We know that sum of angles of a trapezium is 3600.
∴ Area of four sectors = πr2
\(={22\over 7}\times17.5\times17.5\)
=962.5m2
In rt .ㄥed ΔCEB,
\({EC\over EB}=tan60^0\)
\(⇒ {EC\over 25}=\sqrt{3}\)
⇒ EC=25√3m
Now, area of trapezium\(={1\over 2}(AB+DC)\times EC\)
\(={1\over 2}(75+50)\times25\sqrt3\)
\(={1\over 2}\times125\times25\sqrt3\)
=2706.25
Area of remaining portion = 2706.25 - 962.5
=1743.75m2 -
Given, height of the cone=8 cm and radius of the cone = 5 cm
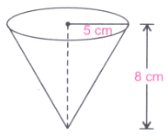
Volume of cone=\(\frac{1}{3}\)πr2h
=\(\frac{1}{3}\)π(5)28 cm3=\(\frac{200}{3}\)π cm3
Radius of one spherical lead shot=0.5 cm
Volume of one spherical lead shot=\(\frac{4}{3}\)πr 3=\(\frac{4}{3}\)π(0.5)3 cm3
=\(\frac{4\times{0.125}}{3}\)π cm3=\(\frac{0.5}{3}\)π cm3
When spherical lead are dropped in the vessel, one fourth of water flows out
Let number of lead shots be n
Volume of n spherical shots = \(\frac{1}{4}\) volume of conical vessel
n\(\left(\frac{0.5}{3}\pi\right)\)=\(\frac{1}{4}\left(\frac{200}{3}\pi\right)\)⇒n(0.5)=50⇒ n=\(\frac{50\times{10}}{5}\)=100 -
There are 12 (4 x 3) possible outcomes as listed below
(1,2), (1,4), (1,6), (3, 2), (3,4), (3, 6), (5, 2), (5,4), (5,6), (7,2). (7, 4) (7, 6) (ii) (a) only (1, 6), (3,.4), (5, 2) given sum as 7
\(\therefore\)Required probability = \(\frac {2} {13}=\frac {1} {4}\)
(b) There is no even sum
\(\therefore\)Required probability= \(\frac {0} {12}=0\)
(c) All the sums are odd
\(\therefore\)Required probability =\(\frac {12} {12} =1\)
(d) only (3, 6), (5, 4), (5, 6), (7, 2). (7, 4) and (7, 6) gives sum as more than 7
\(\therefore\)Required probability = \(\frac {6} {12}\)
=\(\frac {1} {2}\)

























