CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions With Solution 2021
By QB365 on 29 May, 2021
QB365 Provides the HOT Question Papers for Class 10 Maths, and also provide the detail solution for each and every HOT Questions. HOT Questions will help to get more idea about question pattern in every exams and also will help to get more marks in Exams
QB365 - Question Bank Software
CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions With Solution 2021
10th Standard CBSE
-
Reg.No. :
Maths
-
If a1, a2, a3, ....., be an A.P. of non-zero terms, prove that: \(\frac { 1 }{ { a }_{ 1 }{ a }_{ 2 } } +\frac { 1 }{ { a }_{ 2 }{ a }_{ 3 } } +......+\frac { 1 }{ { a }_{ n-1 }{ a }_{ n } } =\frac { n-1 }{ { a }_{ 1 }{ a }_{ n } } .\)
(a) -
The angles of depression of top and bottom of tower as seen from the top of a 100m high cliff are 300 and 600 respectively.Find the height of the tower.
(a) -
Draw a triangle PQR, with PQ = 4 cm, angle \(\angle\) Q = 60o and the median PL = 3.6 cm. Draw another triangle PQ'R' similar to given triangle \(\Delta\)PQR, such that PQ' = \(\frac { 4 }{ 3 } \) PQ.
(a) -
Construct a triangle whose perimeter is 13.5 cm and the ratio of the three sides is 2 : 3 : 4.
(a) -
In the given figure, from each corner of a square ABCD, of side 4 cm, quadrant of a circle of radius 1 cm each is cut and a circle of radius 1 cm is cut from the centre. Find the area of the shaded region.
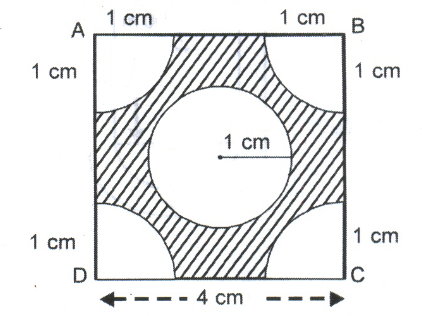 (a)
(a) -
In the adjoining figure, PQR is an equilateral triangle inscribed in a circle of radius 7 cm. Find the area of the shaded region.
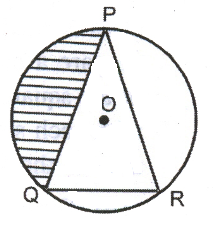 (a)
(a) -
Calculate the area of the shaded portion of the figure alongside.
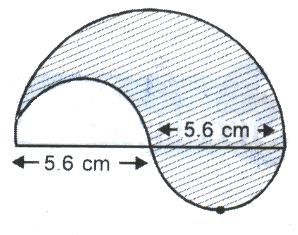 (a)
(a) -
In the figure alongside, crescent is formed by two circles which touch at the point A, O is the centre of the point A, O is the centre of the bigger circle.If CB=9cm and ED=5cm, find the area of the shaded region.[Take \(\pi\)=3.14]
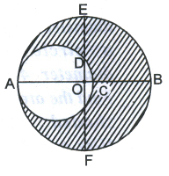 (a)
(a) -
A vessel is in form of an inverted cone.Its height is 8cm and the radius of its top, which is open, is 5cm.It is filled with water up to the brim.When lead shots, each of which is a sphere of radius 0.5cm are dropped into the vessel, one-fourth of the water flows out.Find the number of lead shots dropped in the vessel.
(a) -
From a group of 3 Girls and 2 Boys, two children are selected at random.Find the probability such that at least one boy is selected.
(a)
*****************************************
CBSE 10th Standard Maths Subject HOT Questions 2 Mark Questions With Solution 2021 Answer Keys
-
Here, a1, a2, a3, ....., an be the given A.P. with a1 as first term and d as the common difference
\(\therefore\) a2 = a1 + d
a3 = a1 + 2d
and an = a1 + ( n - 1 )d
Now, L.H.S = \(\frac{1}{{a}_{1}{a}_{2}}+\frac{1}{{a}_{2}{a}_{3}}+...+\frac{1}{{a}_{n-1}{a}_{n}}\)
\(=\frac{1}{{a}_{1}({a}_{1}+d)}+\frac{1}{({a}_{1}+d)({a}_{1}+2d)}+...+\frac{1}{[{a}_{1}+(n-2)d][{a}_{1}+(n-1)d]}\)\(\frac{1}{d}\left[ \frac{1}{{a}_{1}}-\frac{1}{{a}_{1}+d} \right]+\frac{1}{d}\left[ \frac{1}{{a}_{1}+d} -\frac{1}{{a}_{1}+2d}\right]+....+\frac{1}{d}\left[ \frac{1}{{a}_{1}+(n-2)s}-\frac{1}{{a}_{1}+(n-1)d} \right]\)
\(-=\frac{1}{d}\left[ \frac{1}{{a}_{1}}-\frac{1}{{a}_{1}+d}+\frac{1}{{a}_{1}+d}-\frac{1}{{a}_{1}+2d} +....+\frac{1}{{a}_{1}+(n-2)d} -\frac{1}{{a}_{1}+(n-1)d}\right]\)
\(=\frac{1}{d}\left[ \frac{1}{{a}_{1}}-\frac{1}{{a}_{1}+(n-1)d} \right]\)
\(=\frac{1}{d}\left[ \frac{{a}_{1}+(n-1)d-{a}_{1}}{{a}_{1}({a}_{1}+(n-1)d)} \right]\)
\(=\left[ \frac{(n-1)d}{{a}_{1}{a}_{n}} \right]\)
\(=\frac{n-1}{{a}_{1}{a}_{n}}\) = R.H.S. -
66.67m
-
Steps of Construction :
1. Draw a line segment PQ = 4 cm.
2. At Q, construct an ㄥPQY = 60o.
3. Through P, draw an arc of radius 3.6 cm, intersecting QY in S.
4. With S as centre and radius equal to SQ, draw an arc to intersect QY in R.
5. Join PR to get ΔPQR.
6. At P, construct an acute angle ㄥQPX ( < 90o).
7. Mark four points on PX such that PP1 = P1P2 = P2P3 = P3P4.
8. Join P3Q.
9. Through P4, draw P4Q' || P3Q, intersecting PQ in Q', when produced.
10. Through Q', draw Q'R' || QR intersecting PR in PR', when produced.
Thus, ΔPO'R' is the required triangle. -
Steps of Construction :
1. Draw a line segment AB = 13.5 cm.
2. Through A, construct an acute angle ㄥBAX ( < 90o).
3. Mark nine points (2 + 3 + 4 = 9) at equal distances on AX Such that AA1= A1A2 = A2A3 = A3A4 = A4A5 = A5A6 = A6A7 = A7A8 = A8A9.
4. Join A9B.
5. Through A2 and A5 draw A5R || A9B and A2Q || A9B, intersecting AB in Q and R.
6. With Q as centre draw an arc of radius AQ.
7. With R as centre draw another arc of radius RB, intersecting previous arc in P.
8. Join PQ and PR
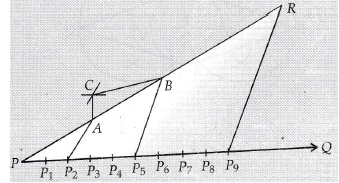
Thus, ΔPQR is the required triangle. -
9.72 cm2
-
30.38 cm2
-
49.28 cm2
-
Suppose R and r be the radii of bigger and smaller circles, respectively
⇒ AB - AC = CB
⇒ 2R-2r=9
⇒ R-r=\(9\over 2\)= 4.5 cm ...(i)
-S.png)
Join AD and CD
ΔAOD ~ ΔDOC
\(⇒\ {OD\over OA}={OC\over OD}\)
⇒ OD2 = OA x OC
⇒ (R-5)2=R x (R-9)
⇒R2+25-10R=R2-9R
⇒ R=25cm
From (i), we have R-r = 4.5
=25 - 4.5 = 20.5cm
Now, area of the shaded portion = πR2-πr2
=π(R2-r2)
=π(R+r)(R-r)
=3.14 x (25+20.5)(25-20.5)
=3.14 x 45.5 x 4.5
= 642.915 cm2
Hence, the required area of the shaded portion is 642.915cm2 -
Given, height of the cone=8 cm and radius of the cone = 5 cm
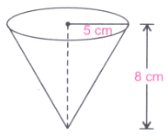
Volume of cone=\(\frac{1}{3}\)πr2h
=\(\frac{1}{3}\)π(5)28 cm3=\(\frac{200}{3}\)π cm3
Radius of one spherical lead shot=0.5 cm
Volume of one spherical lead shot=\(\frac{4}{3}\)πr 3=\(\frac{4}{3}\)π(0.5)3 cm3
=\(\frac{4\times{0.125}}{3}\)π cm3=\(\frac{0.5}{3}\)π cm3
When spherical lead are dropped in the vessel, one fourth of water flows out
Let number of lead shots be n
Volume of n spherical shots = \(\frac{1}{4}\) volume of conical vessel
n\(\left(\frac{0.5}{3}\pi\right)\)=\(\frac{1}{4}\left(\frac{200}{3}\pi\right)\)⇒n(0.5)=50⇒ n=\(\frac{50\times{10}}{5}\)=100 -
Let G1, G2, G3 and B1, B2 be three girls and two boys respectively.
Since two children are selected at random, therefore the following are the possible groups:
B1B2, B1G1, B1G2, B1G3, B2G1, B2G2, B2G3, G1G2, G1G3, G2G3
Total number of cases = 10
Now, at least one boy is selected in the following ways:
B1B2, B1G1, B1G2, B1G3, B2G1, B2G2, B2G3
Total number of favourable cases = 7
\(\therefore\) Required probability = \(\frac {7} {10}\)

























