CBSE 10th Standard Maths Subject Surface Areas and Volumes Case Study Questions 2021
By QB365 on 22 May, 2021
QB365 Provides the updated CASE Study Questions for Class 10 Maths, and also provide the detail solution for each and every case study questions . Case study questions are latest updated question pattern from NCERT, QB365 will helps to get more marks in Exams
QB365 - Question Bank Software
CBSE 10th Standard Maths Subject Surface Areas and Volumes Case Study Questions 2021
10th Standard CBSE
-
Reg.No. :
Maths
-
Ajay is a Class X student. His class teacher Mrs Kiran arranged a historical trip to great Stupa of Sanchi. She explained that Stupa of Sanchi is great example of architecture in India. Its base part is cylindrical in shape. The dome of this stupa is hemispherical in shape, known as Anda. It also contains a cubical shape part called Hermika at the top. Path around Anda is known as Pradakshina Path.

Based on the above information, answer the following questions.
(i) Find the lateral surface area of the Hermika, if the side of cubical part is 8 m.(a) 128 m2 (b) 256 m2 (c) 512 m2 (d) 1024 m2 (ii) The diameter and height of the cylindrical base part are respectively 42 m and 12 m. If the volume of each brick used is 0.01 m3, then find the number of bricks used to make the cylindrical base.
(a) 1663200 (b) 1580500 (c) 1765000 (d) 1865000 (iii) If the diameter of the Anda is 42 m, then the volume of the Anda is
(a) 17475 m3 (b) 18605 m3 (c) 19404 m3 (d) 18650 m3 (iv) The radius of the Pradakshina path is 25 m. If Buddhist priest walks 14 rounds on this path, then find the distance covered by the priest.
(a) 1860 m (b) 3600 m (c) 2400 m (d) 2200 m (v) The curved surface area of the Anda is
(a) 2856 m2 (b) 2772 m2 (c) 2473 m2 (d) 2652 m2 (a) -
One day Rinku was going home from school, saw a carpenter working on wood. He found that he is carving out a cone of same height and same diameter from a cylinder. The height of the cylinder is 24 ern and base radius is 7 cm. While watching this, some questions came into Rinkus mind. Help Rinku to find the answer of the following questions.
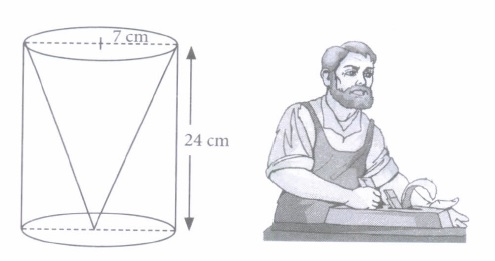
(i) After carving out cone from the cylinder,(a) Volume of the cylindrical wood will decrease. (b) Height of the cylindrical wood will increase. (c) Volume of cylindrical wood will increase. (d) Radius of the cylindrical wood will decrease. (ii) Find the slant height of the conical cavity so formed.
(a) 28 cm (b) 38 cm (c) 35 cm (d) 25 cm (iii) The curved surface area of the conical cavity so formed is
(a) 250 cm2 (b) 550 cm2 (c) 350 cm2 (d) 450 cm2 (iv) External curved surface area of the cylinder is
(a) 876 cm2 (b) 1250 cm2 (c) 1056 cm2 (d) 1025 cm2 (v) Volume of conical cavity is
(a) 1232 cm3 (b) 1248 cm3 (c) 1380 cm3 (d) 999 cm3 (a) -
To make the learning process more interesting, creative and innovative, Amayras class teacher brings clay in the classroom, to teach the topic - Surface Areas and Volumes. With clay, she forms a cylinder of radius 6 ern and height 8 cm. Then she moulds the cylinder into a sphere and asks some questions to students.
(i) The radius of the sphere so formed is(a) 4 cm (b) 6 cm (c) 7 cm (d) 8 cm (ii) The volume of the sphere so formed is
(a) 905.14 cm3 (b) 903.27 cm3 (c) 1296.5 cm3 (d) 1156.63 cm3 (iii) Find the ratio of the volume of sphere to the volume of cylinder.
(a) 2:1 (b) 1:2 (c) 1:1 (d) 3: 1 (iv) Total surface area of the cylinder is
(a) 528 cm2 (b) 756 cm2 (c) 625 cm2 (d) 636 cm2 (v) During the conversion of a solid from one shape to another the volume of new shape will
(a) be increase (b) be decrease (c) remain unaltered (d) be double (a) -
A carpenter used to make and sell different kinds of wooden pen stands like rectangular, cuboidal, cylindrical, conical. Aarav went to his shop and asked him to make a pen stand as explained below. Pen stand must be of the cuboidal shape with three conical depressions, which can hold 3 pens. The dimensions of the cuboidal part must be 20 cm x 15 cm x 5 cm and the ffrlog radius and depth of each conical depression must be 0.6 cm and 2.1 cm respectively.
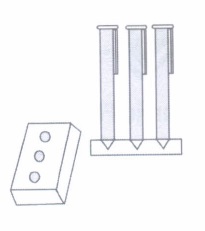
Based on the above information, answer the following questions.
(i) The volume of the cuboidal part is(a) 1250 cm3 (b) 1500 cm3 (c) 1625 cm3 (d) 1500 cm3 (ii) Total volume of conical depressions is
(a) 2.508 cm3 (b) 1.5 cm3 (c) 2.376 cm3 (d) 3.6 cm3 (iii) Volume of the wood used in the entire stand is
(a) 631.31 cm3 (b) 3564 cm3 (c) 1502.376 cm3 (d) 1497.624 cm3 (iv) Total surface area of cone of radius r is given by
\((a) \pi r l+\pi r^{2}\) \((b) 2 \pi r l+\pi r^{2}\) \((c) \pi r^{2} l+\pi r^{2}\) \((d) \pi r l+2 \pi r^{3}\) (v) If the cost of wood used is Rs 5 per cm3, then the total cost of making the pen stand is
(a) Rs 8450.50 (b) Rs 7480 (c) Rs 9962.14 (d) Rs 7488.12 (a) -
Meera and Dhara have 12 and 8 coins respectively each of radius 3.5 cm and thickness 0.5 cm. They place their coins one above the other to form solid cylinders .

Based on the above information, answer the following questions.
(i) Curved surface area of the cylinder made by Meera is(a) 144 cm2 (b) 132 cm2 (c) 154 cm2 (d) 142 cm2 (ii) The ratio of curved surface area of the cylinders made by Meera and Dhara is
(a) 2: 5 (b) 3: 2 (c) 1: 2 (d) 2: 7 (iii) The volume of the cylinder made by Dhara is
(a) 154 cm3 (b) 144 cm3 (c) 132 cm3 (d) 142 cm3 (iv) The ratio of the volume of the cylinders made by Meera and Dhara is
(a) 1:2 (b) 2: 5 (c) 3: 2 (d) 4: 3 (v) When two coins are shifted from Meeras cylinder to Dhara's cylinder, then
(a) Volume of two cylinder become equal (b) Volume of Meera's cylinder> Volume of Dharas cylinder (c) Volume of Dhara's cylinder> Volume of Meeras cylinder (d) None of these (a)
Case Study Questions
*****************************************
CBSE 10th Standard Maths Subject Surface Areas and Volumes Case Study Questions 2021 Answer Keys
-
(i) (b): Lateral surface area of Hermika which is cubical in shape = 4a2 = 4 x (8)2 = 256 m2
(ii) (a): Diameter of cylindrical base = 42 m
\(\therefore\) Radius of cylindrical base (r) = 21 m
Height of cylindrical base (h) = 12 m
\(\therefore\) Number of bricks used \(=\frac{\frac{22}{7} \times 21 \times 21 \times 12}{0.01}\)
= 1663200
(iii) (c) : Given, diameter of Anda which is
hemispherical in shape = 42 m
\(\Rightarrow\) Radius of Anda (r) = 21 m
\(\therefore \quad \text { Volume of } A n d a =\frac{2}{3} \pi r^{3}=\frac{2}{3} \times \frac{22}{7} \times 21 \times 21 \times 21 \)
\(=44 \times 21 \times 21=19404 \mathrm{~m}^{3}\)
(iv) (d): Given, radius of Pradakshina Path (r) = 25 m
\(\therefore\) Perimeter of path = \(2\pi r\)
\(=\left(2 \times \frac{22}{7} \times 25\right) \mathrm{m}\)
· . Distance covered by priest \(=14 \times 2 \times \frac{22}{7} \times 25\)
= 2200 m
(v) (b):\(\because\) Radius of Anda (r) = 21 m
\(\therefore\) Curved surface area of Anda = \(2\pi r^{2}\)
\(=2 \times \frac{22}{7} \times 21 \times 21=2772 \mathrm{~m}^{2}\) -
(i) (a)
(ii) (d): Slant height of conical cavity \(l=\sqrt{h^{2}+r^{2}}\)
\(=\sqrt{(24)^{2}+(7)^{2}}=\sqrt{576+49}=\sqrt{625}=25 \mathrm{~cm}\)
(iii) (b): Curved surface area of conical cavity = \(\pi r l\)
\(=\frac{22}{7} \times 7 \times 25=550 \mathrm{~cm}^{2}\)
(iv) (c) : External curved surface area of cylinder
\(=2 \pi r h=2 \times \frac{22}{7} \times 7 \times 24=1056 \mathrm{~cm}^{2}\)
(v) (a): Volume of conical cavity \(=\frac{1}{3} \pi r^{2} h\)
\(=\frac{1}{3} \times \frac{22}{7} \times 7 \times 7 \times 24=1232 \mathrm{~cm}^{3}\) -
(i) (b): Since, volume of sphere = volume of cylinder
\(\Rightarrow \frac{4}{3} \pi R^{3}=\pi r^{2} h\)
where R, r are the radii of sphere and cylinder respectively.
\(\Rightarrow R^{3}=\frac{6 \times 6 \times 8 \times 3}{4}=(6)^{3} \Rightarrow R=6 \mathrm{~cm}\)
\(\therefore\) Radius of sphere = 6 cm
(ii) (a): Volume of sphere \(=\frac{4}{3} \pi R^{3}\)
\(=\frac{4}{3} \times \frac{22}{7} \times 6 \times 6 \times 6=905.14 \mathrm{~cm}^{3}\)
(iii) (c): \(\because\) Volume of sphere = Volume of cylinder
\(\therefore\) Required ratio = 1 : 1
(iv) (a): Total surface area of the cylinder \(=2 \pi r(r+h)\)
\(=2 \times \frac{22}{7} \times 6(6+8)=2 \times \frac{22}{7} \times 6 \times 14=528 \mathrm{~cm}^{2}\)
(v) (c) -
(i) (b): Volume of cuboidal part = 1 x b x h = (20 x 15 x 5) cm3 = 1500 cm3
(ii) (c): Radius of conical depression, r = 0.6 cm
Height of conical depression, h = 2.1 cm
\(\therefore\) Total volume of conical depressions \(=3 \times \frac{1}{3} \pi r^{2} h\)
\(=\frac{22}{7} \times 0.6 \times 0.6 \times 2.1=\frac{2.376}{1000}-2.376 \mathrm{~cm}^{3}\)
(iii) (d): Volume of wood used in the entire stand = Volume of cuboidal part - Total volume of conical depressions
= 1500 - 2.376 = 1497.624 cm3
(iv) (a)
(v) (d): Cost of wood per cm3 = Rs 5
\(\therefore\) Total cost of making the pen stand
= Rs (5 x 1497.624) = Rs 7488.12 -
We have, radius of each coin = 3.5 cm
\(=\frac{35}{10} \mathrm{~cm}=\frac{7}{2} \mathrm{~cm}\)
Thickness of each coin \(=0.5 \mathrm{~cm}=\frac{1}{2} \mathrm{~cm}\)
So,height of cylinder made by Meera (h1) \(=12 \times \frac{1}{2}=6 \mathrm{~cm}\)
and height of cylinder made by Dhara (h2)
\(=8 \times \frac{1}{2}=4 \mathrm{~cm}\)
(i) (b): Curved surface area of cylinder made by Meera \(=2 \times \frac{22}{7} \times \frac{7}{2} \times 6=132 \mathrm{~cm}^{2}\)
(ii) (b): Required ratio
\(=\frac{\text { Curved surface area of cylinder made by Meera }}{\text { Curved surface area of cylinder made by Dhara }}\)
\(=\frac{2 \pi r h_{1}}{2 \pi r h_{2}}=\frac{h_{1}}{h_{2}}=\frac{6}{4}=\frac{3}{2} \text { i.e., } 3: 2\)
(iii) (a): Volume of cylinder made by Dhara \(=\pi r^{2} h_{2}\)
\(=\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2} \times 4=154 \mathrm{~cm}^{3}\)
(iv) (c): Required ratio
\(=\frac{\text { Volume of cylinder made by Meera }}{\text { Volume of cylinder made by Dhara }} \)
\(=\frac{\pi r^{2} h_{1}}{\pi r^{2} h_{2}}=\frac{h_{1}}{h_{2}}=\frac{6}{4}=\frac{3}{2} \text { i.e., } 3: 2\)
(v) (a): When two coins are shifted from Meera's cylinder to Dhara's cylinder, then length of both cylinders become equal. So, volume of both cylinders become equal
Case Study Questions

























