(a) Here, height(h)=1 km = 1000m, g = 10m/s²
Velocity attained by the rain drop in freely falling through a height h.
\(v=\sqrt { 2gh } =\sqrt { 2\times 10\times 1000 }\)
\( \\ =100\sqrt { 2 } m/s\)
\(\\ =100\sqrt { 2 } \times \frac { 60\times 60 }{ 1000 } Km/h\)
\(\\ =360\sqrt { 2 } km/h\approx 510km/h\) 
(b) Diameter of the drop(d) = 2r = 4mm
Radius of the drop(r) = 2mm = 2 x 10^-3m
Mass of a rain drop(m)=Vx\(\rho \)
\(=\frac { 4 }{ 3 } \pi { r }^{ 3 }\rho =\frac { 4 }{ 3 } \times \frac { 22 }{ 7 } \times (2\times { 10 }^{ -3 })^{ 3 }\times { 10 }^{ 3 }\)
\( \approx 3.4\times { 10 }^{ -5 }kg\)
\(\\ Momentum \ of \ the \ rain \ drop(\rho )=mv \)
\(\\ =3.4\times { 10 }^{ -5 }\times 100\sqrt { 2 }\)
\(\\ \approx 4.7\times { 10 }^{ -3 }{ kg }^{ -m/s }\)
(c) Time required to flatten the drop
= time taken by the drop to travel the distance equal to the diameter of the drop near the ground
\(t=\frac { d }{ v } =\frac { 4\times { 10 }^{ -3 } }{ 100\sqrt { 2 } } =0.028\times { 10 }^{ -3 }s\)
\(\\ =2.8\times { 10 }^{ -5 }s\)
(d) Force exerted by a rain drop
\(F=\frac { Change \ in \ momentum }{ Time } \)
\(=\frac { \rho -0 }{ t } =\frac { 4.7\times { 10 }^{ -3 } }{ 2.8\times { 10 }^{ -5 } } \approx 168N\)
(e) Radius of the umbrella(R) = \(\frac { 1 }{ 2 } m\)
\(\therefore \ Area \ of \ the \ umbrella(A)=\pi { R }^{ 2 }\)
\(\\ =\frac { 22 }{ 7 } \times { (\frac { 1 }{ 2 } ) }^{ 2 }=\frac { 22 }{ 28 } =\frac { 11 }{ 14 } \approx 0.8{ m }^{ 2 }\)
Number of drops striking the umbrella simultaneously with average of 5cm or 5 x 10^-2m
\(=\frac { 0.8 }{ { (5\times { 10 }^{ -2 }) }^{ 2 } } =320\)
Net force exerted on umbrella 
= 320 x 168 = 53760 N

On smooth inclined plane Acceleration of a body sliding down a smooth inclined plane, a =g\(\sin { \theta } \)
Here,    \(\theta =4{ 5 }^{ o }\)
\( \therefore \quad a=g\sin { 45^{ 0 } } =\frac { g }{ \sqrt { 2 } } \)
Let the travelled distance be s.
using the equation of motion, s = ut + \(\frac { 1 }{ 2 } \) at², we get
s = \(0.t+\frac { 1 }{ 2 } \frac { g }{ \sqrt { 2 } } { T }^{ 2 }\quad or\quad s=\frac { g{ T }^{ 2 } }{ \sqrt [ 2 ]{ 2 } } \)
On rough inclined plane
Acceleration of the body, 
 \(a\quad =g(\sin { \theta -\mu \cos { \theta ) } } \)
\( =g(sin45^{ 0 }-\mu \cos { 45^{ 0 }) } \)
\(=\frac { g(1-\mu ) }{ 2 } \left[ as\sin { 45^{ 0 }=\frac { 1 }{ \sqrt { 2 } } } \right] \)
Again using equation of motion,  s = ut + \(\frac { 1 }{ 2 } \)  at ²,  we get
\(s=0(pT)+\frac { 1 }{ 2 } \frac { g(1-\mu ) }{ \sqrt { 2 } } (pT)^{ 2 }\)
\( s=\frac { g(1-\mu ){ p }^{ 2 }{ T }^{ 2 } }{ \sqrt [ 2 ]{ 2 } } \)
From Eqs.(i) and (ii) we get
\(\frac { gT^{ 2 } }{ \sqrt [ 2 ]{ 2 } } =\frac { g(1-\mu ){ p }^{ 2 }{ T }^{ 2 } }{ \sqrt [ 2 ]{ 2 } } \)
\(or\quad (1-\mu ){ p }^{ 2 }=1\ or\ 1-\mu =\frac { 1 }{ { p }^{ 2 } } \)
\(\\ or\quad \mu =\left( 1\frac { 1 }{ { p }^{ 2 } } \right) \) 

(a) As no external torque is involved with child + turntable system, so angular momentum of system remains constant.
or   \({ I }_{ i }{ \omega }_{ i }={ I }_{ f }{ \omega }_{ f }\)
\({ I }_{ i }{ \omega }_{ i }\) = initial angular momentum, \({ I }_{ f }{ \omega }_{ f }\) = final angular momentum
Here,      \({ \omega }_{ i }=40\quad rpm\Rightarrow { I }_{ f }=\frac { 2 }{ 5 } { I }_{ i }\)
Substituting, we get
\({ I }_{ i }\times40=\frac { 2 }{ 5 } { I }_{ i }\times{ \omega }_{ f }\)
or  \({ \omega }_{ f }=\frac { 5\times40 }{ 2 } =100\quad rpm\)
(b) \(\frac{\text { Final Kinetic Energy of rotation }}{\text { Initial Kinetic Energy of rotation }}=\frac{\frac{1}{2} I_{2} \omega_{2}^{2}}{\frac{1}{2} I_{1} \omega_{1}^{2}}=\frac{\frac{1}{2} I_{2}\left(2 \pi v_{2}\right)^{2}}{\frac{1}{2} I_{1}\left(2 \pi v_{1}\right)^{2}}\)
\(=\frac{I_{2} v_{2}^{2}}{I_{1} v_{1}^{2}}=\frac{\frac{2}{5} I_{1} \times(100)^{2}}{\frac{2}{5} I_{1} \times(40)^{2}}\)
= 2.5
Clearly, final (K.E) becomes more because the child used his internal energy when he folds his hands to increase the kinetic energy.

Young's modulus \((Y)=\frac { Stress }{ Longitudinal\ strain } \)
For same longitudinal strain \(Y\alpha stress\)
\(\frac { { Y }_{ stress } }{ { Y }_{ rubber } } =\frac { { (stress) }_{ steel } }{ { (stress) }_{ rubber } } \)
But          \({ Y }_{ steel }>{ Y }_{ rubber }\)
\(\frac { { Y }_{ steel } }{ { Y }_{ rubber } } >1\)
Therefore from Eq(i) we get
\(\frac { { (stress) }_{ steel } }{ { (stress) }_{ rubber } } >1\)
\(or { (stress) }_{ steel }>(stress)_{ rubber }\)

(i) Here, \(\mu =5.0,\ T={ 7 }^{ \circ }C=273+7\ =280K\)
Number of atoms =\(\mu { N }_{ A }=5.0\times 6.02\times { 10 }^{ 23 }\approx 30\times { 10 }^{ 23 }\)
(ii) Average kinetic energy per molecule = \(\frac { 3 }{ 2 } { k }_{ B }T\)
Total internal energy = \(\frac { 3 }{ 2 } { k }_{ B }T\times N\)
= \(\frac { 3 }{ 2 } \times 30\times { 10 }^{ 23 }\times 1.38\times { 10 }^{ -23 }\times 280=1.74\times { 10 }^{ 4 }\quad J\) 

\(Given,\ v=150\ km.h,\ V=20\times 20\times 1.5,\)
\(\\ N=10,\ d=2\times 10=20\ m\)
\(\\ Time\ taken\ by\ plane,\ t=\lambda /v\ and\ meanj\ free\ path\)
\(\\ i.e., \lambda =\frac { 1 }{ \sqrt { 2\pi { d }^{ 2 }n } }\)
\( \\ where,d\ =diameter\ and\ n=\ number\ of\ density\)
\(\\ So,\ n=\frac { N }{ V } =\frac { 10 }{ 20\times 20\times 1.5 } =0.0167\quad { km }^{ -3 }\)
\(\\ and\ time\ elapse\ between\ collision,\)
\(\\ t=\frac { 1 }{ \sqrt { 2\pi { d }^{ 2 }(N/V)\times v } } \)
\(\\ =\frac { 1 }{ 1.414\times 3.14\times \left( 20 \right) ^{ 2 }\times 0.0167\times { 10 }^{ -3 }\times 150 } \) 
= 225 hours.

Given equation is \(y=2\cos { 2\pi } \left( 10t-0.0080x+3.5 \right) \)  
Comparing with standard equation,
\(y=2\cos { 2\pi } \left( 10t-0.0080x+3.5 \right) \)   
\(\\ y=a\cos { \left( \omega t-kx+\phi \right) } \)
\(a=2cm,\omega =\frac { 2\pi }{ T } =20\pi ,\ T=0.1s\)
\(k=\frac { 2\pi }{ \lambda } =0.008\times 2\pi \Rightarrow \lambda =\frac { 2\pi }{ 2\pi \times 0.008 } =1.25m\)
\(\\ \phi =2\pi \times 3.5=7\pi rad\)
When x = \(\frac { \lambda }{2 } \)
\({ \phi }_{ 3 }=\frac { 2\pi }{ \lambda } \times \lambda /2=\pi rad\).        

Given equation is \(y=2\cos { 2\pi } \left( 10t-0.0080x+3.5 \right) \)  
Comparing with standard equation,
\(y=2\cos { 2\pi } \left( 10t-0.0080x+3.5 \right) \)
\(y=a\cos { \left( \omega t-kx+\phi \right) } \)
\( a=2cm,\omega =\frac { 2\pi }{ T } =20\pi ,\ T=0.1s\)
\( k=\frac { 2\pi }{ \lambda } =0.008\times 2\pi \Rightarrow \lambda =\frac { 2\pi }{ 2\pi \times 0.008 } =1.25m\)
\(\\ \phi =2\pi \times 3.5=7\pi rad\)
\(At\ t=T;\phi =\frac { 2\pi }{ T } =\frac { 2\pi }{ 0.1 } =20\pi rad\) 
\(\\ and\ at\ t=5s;{ \phi }^{ ' }=\frac { 2\pi }{ 0.1 } \times 5.100\pi rad\)
\(\therefore phase\ difference\ { \phi }^{ ' }-\ \phi =100\pi rad-20\pi rad=8020\pi rad\).

A: Find amplitude of the given wave.
Given, progressive wave,
y=5sin(100πt−0.4πx)
So, amplitude of the given wave,
a=5 m
Find wavelength of the given wave.
Since we know
k=2π/λ
According to the given wave equation,
Angular wavenumber, k=0.4π
2π/λ=0.4π
λ=20/4=5 m
Final answer: 5m;5m
B: Find frequency of the wave
Given, wave equation,
y=5sin(100πt−0.4πx)
From the given waveequation, angular frequency,
ω=100π
Since ω=2πf
So,frequency,
f=ω²/π
\(\omega =2\pi v=100\pi v=50Hz\)
f=100π/2π=50 Hz
Find wave velocity of the wave.
Given, wavelength of the wave,
λ=5m
Wave velocity, v=fλ
v=50×5=250m/s
Final Answer: 50 Hz;250m/s
C: Particle velocity: Particle velocity is the velocity with which particles vibrate during the wave propagation.
Given, wave equation,
y=5sin(100πt−0.4πx)
Velocity of the particle by, \(\frac{\partial_{\mathrm{y}}}{\partial_{\mathrm{t}}}=\frac{\partial}{\partial_{\mathrm{t}}}(5 \sin (100 \pi \mathrm{t}-0.4 \pi \mathrm{t})) \)
\( \frac{\partial_y}{\partial_t}=500 \pi \cos (100 \pi t-0.4 \pi x)\)
So, particle velocity amplitude = \(500 \pi \mathrm{m} / \mathrm{s}\)
Final Answer: \(500 \pi \mathrm{m} / \mathrm{s}\)

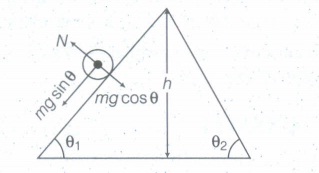
(a) Using law of conservation of energy

\(\frac { 1 }{ 2 } mv^{ 2 }+\frac { 1 }{ 2 } I{ \omega }^{ 2 }\)=mgh
or  \(\frac { 1 }{ 2 } mv^{ 2 }+\frac { 1 }{ 2 } \left( \frac { 2 }{ 5 } m{ R }^{ 2 } \right) \frac { { v }^{ 2 } }{ { R }^{ 2 } } =mgh\)
or \(\frac { 7 }{ 10 } v^{ 2 }=gh\) or v =\(\sqrt { \frac { 10gh }{ 7 } } \)
Since h is same for both the inclined planes therefore v is the same
(b) 

\(l=\frac { 1 }{ 2 } \left( \frac { g\quad sin\quad \theta }{ 1+\frac { { K }^{ 2 } }{ { R }^{ 2 } } } \right) { t }^{ 2 }=\frac { g\quad sin\theta }{ 2\left( 1+\frac { 2 }{ 5 } \right) } { t }^{ 2 }=\frac { 5gsin\theta }{ 14 } { t }^{ 2 }\)
or \(t=\sqrt { \frac { 14l }{ 5g\quad sin\quad \theta } } \)
Now, sin \(\theta\)=\(\frac { h }{ l } \) or l =\(\frac { h }{ sin\theta } \)
∴ \(t=\frac { 1 }{ sin\quad \theta } \sqrt { \frac { 14h }{ 5g } } \)
Lesser the value of,\(\theta\) more will be t.
(c) Clearly, the solid sphere will take longer to roll down the plane with smaller inclination.

Here, m = 5.30\(\times\)10^-26 kg
I = 1.94\(\times\)10^-46kg m²
v =500 m/s
If \(\frac { m }{ 2 } \)is mass of each atom of oxygen and 2r is distance between the two atoms as shown in Fig.then
\(I=\frac { m }{ 2 } { r }^{ 2 }+\frac { m }{ 2 } { r }^{ 2 }={ mr }^{ 2 }\)
\(r=\sqrt { \frac { 1 }{ m } } =\sqrt { \frac { 1.94\times { 10 }^{ -46 } }{ 5.30\times { 10 }^{ -26 } } } \)
= 0.61\(\times\)10^-10 m
As K.E. of rotation= \(\frac { 2 }{ 3 } \)K.E of translation
∴ \(\frac { 1 }{ 2 } I{ \omega }^{ 2 }=\frac { 2 }{ 3 } \times \frac { 1 }{ 2 } { m\omega }^{ 2 }\)
\(\frac { 1 }{ 2 } ({ mr }^{ 2 }){ \omega }^{ 2 }=\frac { 1 }{ 2 } { mv }^{ 2 }\)
\(\omega =\sqrt { \frac { 2 }{ 3 } } \frac { \upsilon }{ r } =\sqrt { \frac { 2 }{ 3 } } \times \frac { 500 }{ 0.61\times { 10 }^{ -10 } } \) = 6.7 10¹² rad/s

Given, speed of the car as well as truck = 72km/h
\(=72 \times \frac{5}{18} \mathrm{~m} / \mathrm{s}=20 \mathrm{~m} / \mathrm{s}\)
Retarded motion for truck
v = u + a_t t
0 = 20+ a_t x 5
or a_t = -4 m/s²
Retarded motion for the car
v = u + a_c t
0 = 20+ a_c x 3
or   \(a_{c}=-\frac{20}{3} \mathrm{~m} / \mathrm{s}^{2}\)
Let car be at a distance x from truck, when truck gives the signal and t be the time taken to cover this distance.
As human response time is 0.5 s, therefore time of retarded motion of car is (t - 0.5)s.
Velocity of car after time t ,
\(v_{c}=u-a t=20-\left(\frac{20}{3}\right)(t-0.5)\)
Velocity of truck after time t,
v_t = 20 - 4t
To avoid the car bumb onto the truck, v_c = v_t
\(20-\frac{20}{3}(t-0.5)=20-4 t\)
or    \(4 t=\frac{20}{3}(t-0.5)\)
or    \(t=\frac{5}{3}(t-0.5)\)
or    3t = 5t - 2.5
or    \(t=\frac{2.5}{2}=\frac{5}{4} \mathrm{~s}\)
Distance travelled by the truck in time t,
\(s_{t}=u_{t} t+\frac{1}{2} a_{t} t^{2}\)
\(=20 \times \frac{5}{4}+\frac{1}{2} \times(-4) \times\left(\frac{5}{4}\right)^{2}\)
S_t = 25-3.125= 21.875m
Distance travelled by the car in time t = Distance travelled by the car in 0.5 s (without retardation) + Distance travelled by car in (t - 0.5)s (with retardation)
\(s_{c}=(20 \times 0.5)+20\left(\frac{5}{4}-0.5\right)-\frac{1}{2}\left(\frac{20}{3}\right)\left(\frac{5}{4}-0.5\right)^{2}\)
= 23125 m
\(\therefore\) S_c - S_t = 23.125- 21.87
= 1.250 m
Therefore, to avoid the bumb onto the truck, the car must maintain a distance from the truck more than 1.250 m.