CBSE 12th Standard Physics Subject Alternating Current Ncert Exemplar 2 Mark Questions With Solutions 2021
By QB365 on 22 May, 2021
QB365 Provides the updated NCERT Exemplar Questions for Class 12, and also provide the detail solution for each and every case study questions. NCERT Exemplar questions are latest updated question pattern from NCERT, QB365 will helps to get more marks in Exams
QB365 - Question Bank Software
CBSE 12th Standard Physics Subject Alternating Current Ncert Exemplar 2 Mark Questions With Solutions 2021
12th Standard CBSE
-
Reg.No. :
Physics
-
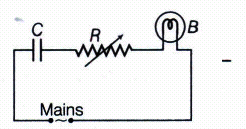
A capacitor C, a variable resistance R and a bulb B are connected in series to the AC mains in circuit as shown in the figure.The bulb glows of the bulb change, if
(i) a dielectric slab is introduced between the plates of the capacitor, keeping resistance R to be same;
(ii) the resistance R is increased keeping will capacitance?
 (a)
(a) -
Show that heat produced in a cycle of AC is same as the heat produced by DC with \(I={ I }_{ rms }\).
(a) -
In series L-C-R circuit, the plot of Imax\(\omega \) versus is shown in the figure. Find the bandwidth and mark in the figure.
 (a)
(a) -
A series L-C-R circuit with \(R=20\Omega \), L = 1.5H and \(C=5\mu F\) is connected to a variable frequency of 200 V AC supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit, what is the average complete cycle?
(a) -
Obtain the resonant frequency \(({ \omega }_{ r })\) of a series L-C-R circuit with L = 2.0 H, \(C=32\mu F \) and \(R=10\Omega \) . What is the Q-value of this circuit?
(a)
*****************************************
CBSE 12th Standard Physics Subject Alternating Current Ncert Exemplar 2 Mark Questions With Solutions 2021 Answer Keys
-
(i) As the electric slab is introduced between the plates of the capacitor, its capacitance. Hence, the potential drop across the capacitor will i.e \(V=\frac { Q }{ C } \)
As a result, the potential drop across the bulb will increase as they are connected in series. Thus its brightness will increase.
(ii) As a resistance R is increased, the potential drop across the resistor will increase. As a result, the potential drop across the bulb will decrease as they are connected in series. Thus, its brightness will decrease. -
For an AC, \({ I }_{ t }={ I }_{ 0 }\sin { \omega t } \)
Heat produced in a resistance in small time dt,
\(dU={ I }_{ t }^{ 2 }Rdt\)
\(=\left( { I }_{ 0 }\sin { \omega t } \right) Rdt\)
\(\therefore \) Heat produced during a full cycle of AC,
\(=\int { dU } ={ I }_{ 0 }^{ 2 }R\int _{ 0 }^{ T }{ \sin ^{ 2 }{ \omega t } dt }\)
\( =\frac { { I }_{ 0 }^{ 2 } }{ 2 } R\left[ T \right] \)
\(\left[ \because \omega T=2\pi \right] \)
\(\Rightarrow \ U={ I }_{ rms }^{ 2 }RT\ \left[ \therefore { I }_{ rms }=\frac { { I }_{ 0 } }{ \sqrt { 2 } } \right] \)
Thus, we see that AC produces same heating effect as DC of value \(I={ I }_{ rms }\). -
Consider the diagram.
Bandwidth = \(\omega _{ 2 }-{ \omega }_{ 1 }\),
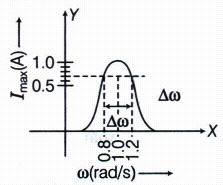
Where , \(\omega_1\) and \(\omega_2\) correspond to frequencies at which magnitude of current is \(\frac { 1 }{ \sqrt { 2 } } \) times of maximum value.
\({ I }_{ rms }=\frac { { I }_{ max } }{ \sqrt { 2 } } =\frac { 1 }{ \sqrt { 2 } } \approx 0.7A\)
Clearly, from the diagram, the corresponding frequencies are 0.8 rad/s and rad/s.
\(\triangle \omega \) = B and width = 1.2 - 0.8 = 0.4 rad/s -
When the frequency of the supply equals the natural frequency of the circuit, resonance occurs.
\({ Z }_{ r }=R=20\Omega \)
\({ I }_{ rms }=\frac { { V }_{ rms } }{ { Z }_{ r } } =\frac { 200 }{ 20\quad } =10A\)
Average power transferred in one cycle,
\({ P }_{ av }={ V }_{ rms }{ I }_{ rms }\cos { \phi } \)
= 200 x 10 x cos 00 [\(\phi ={ 0 }^{ 0 }\)]
= 2000W = 2kW -
Given, L = 2.0 H, C = 32 x 10-6 F,
\(R=10\Omega \)
\({ \omega }_{ r }\) = ? Q = ?
\({ \omega }_{ r }=\frac { 1 }{ \sqrt { LC } } =\frac { 1 }{ \sqrt { 2.0\times 32\times { 10 }^{ -6 } } } \)
\(\frac { { 10 }^{ 3 } }{ 8 } =125rad/s\)
\( Q=\frac { 1 }{ R } \sqrt { \frac { L }{ C } } =\frac { 1 }{ 10 } \sqrt { \frac { 2 }{ 32\times { 10 }^{ -6 } } } \)
\(\frac { 1 }{ 10\times 4\times { 10 }^{ -3 } } =25\)






































