CBSE 12th Standard Physics Subject Atoms Chapter Case Study Questions With Solution 2021
By QB365 on 21 May, 2021
QB365 Provides the updated CASE Study Questions for Class 12 , and also provide the detail solution for each and every case study questions . Case study questions are latest updated question pattern from NCERT, QB365 will helps to get more marks in Exams
QB365 - Question Bank Software
CBSE 12th Standard Physics Subject Atoms Case Study Questions With Solution 2021
12th Standard CBSE
-
Reg.No. :
Physics
-
Niels Bohr introduced the atomic Hydrogen model in 1913. He described it as a positively charged nucleus, comprised of protons and neutrons, surrounded by a negatively charged electron cloud. In the model, electrons orbit the nucleus in atomic shells. The atom is held together by electrostatic forces between the positive nucleus and negative surroundings.
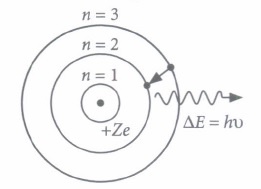
Bohr correctly proposed that the energy and radii of the orbits of electrons in atoms are quantized, with energy for transitions between orbits given by
\(\Delta E=h v=E_{i}-E_{f}\) Where \(\Delta E\) is the change in energy between the initial and final orbits and hv is the energy of an absorbed or emitted photon.
(i) In the Bohr model of the hydrogen atom, discrete radii and energy states result when an electron circles the atom in an integer number of(a) de Broglie wavelengths (b) wave frequencies (c) quantum numbers (d) diffraction patterns. (ii) The angular speed of the electron in the nth orbit of Bohr's hydrogen atom is
(a) directly proportional to n (b) inversely proportional to \(\sqrt{n}\) (c) inversely proportional to n2 (d) inversely proportional to n3 (iii) When electron jumps from n = 4 level to n = 1 level, the angular momentum of electron changes by
\(\text { (a) } \frac{h}{2 \pi}\) \(\text { (b) } \frac{h}{\pi}\) \(\text { (c) } \frac{3 h}{2 \pi}\) \(\text { (d) } \frac{2 h}{\pi}\) (iv) The lowest Bohr orbit in hydrogen atom has
(a) the maximum energy (b) the least energy (c) infinite energy (d) zero energy (v) Which of the following postulates of the Bohr modelled to the quantization of energy of the hydrogen atom?
(a) The electron goes around the nucleus in circular orbits. (b) The angular momentum of the electron can only be an integral multiple of h/2\(\pi\) (c) The magnitude of the linear momentum of the electron is quantized. (d) Quantization of energy is itself a postulate of the Bohr model. (a) -
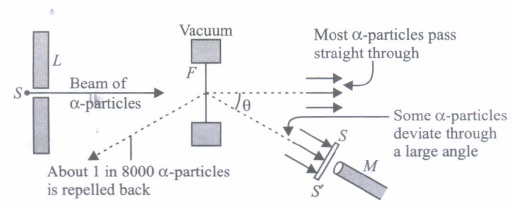
In 1911, Rutherford, along with his assistants, H. Geiger and E. Marsden, performed the alpha particle scattering experiment. H. Geiger and E. Marsden took radioactive source \(\left(\begin{array}{c} 214 \\ 83 \end{array} \mathrm{Bi}\right)\) for a-particles. A collimated beam of a-particles of energy 5.5 MeV was allowed to fall on 2.1 x 10-7 m thick gold foil. The a-particles were observed through a rotatable detector consisting of a Zinc sulphide screen and microscope. It was found that a-particles got scattered. These scattered a-particles produced scintillations on the zinc sulphide screen. Observations of this experiment are as follows.
(I) Most of the a-particles passed through the foil without deflection.
(II) Only about 0.14% of the incident a-particles scattered by more than 1°.
(III) Only about one a-particle in every 8000 a-particles deflected by more than 90°.
These observations led to many arguments and conclusions which laid down the structure of the nuclear model of an atom.
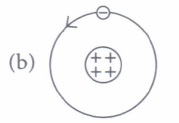
(i) Rutherford's atomic model can be visualised as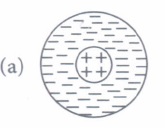
(ii) Gold foil used in Geiger-Marsden experiment is about 10-8 m thick. This ensures
(a) gold foil's gravitational pull is small or possible (b) gold foil is deflected when a-particle stream is not incident centrally over it (c) gold foil provides no resistance to passage of a-particles (d) most a-particle will not suffer more than 1° scattering during passage through gold foil (iii) In Geiger-Marsden scattering experiment, the trajectory traced by an a-particle depends on
(a) number of collision (b) number of scattered a- particles (c) impact parameter (d) none of these (iv) In the Geiger-Marsden scatteririg experiment, in case of head-on collision, the impact parameter should be
(a) maximum (b) minimum (c) infinite (d) zero (v) The fact only a small fraction of the number of incident particles rebound back in Rutherford scattering indicates that
(a) number of a-particles undergoing head-on-collision is small (b) mass of the atom is concentrated in a small volume (c) mass of the atom is concentrated in a large volume (d) both (a) and (b). (a) -
At room temperature, most of the H-atoms are in ground state. When an atom receives some energy (i.e., by electron collisions), the atom may acquire sufficient energy to raise electron to higher energy state. In this condition, the atom is said to be in excited state. From the excited state, the electron can fall back to a state of lower energy emitting a photon equal to the energy difference of the orbit.
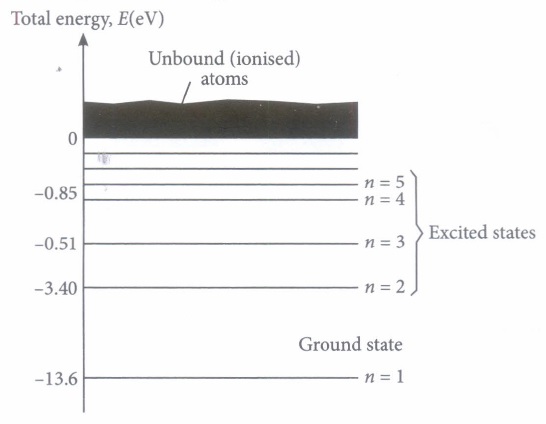
In a mixture of H-He+ gas (He+ is single ionized He atom), H-atoms and He+ ions are excited to their respective first excited states. Subsequently, H-atoms transfer their total excitation energy to He+ ions (by collisions).
(i) The quantum number n of the state finally populated in He+ ions is(a) 2 (b) 3 (c) 4 (d) 5 (ii) The wavelength of light emitted in the visible region by He+ ions after collisions with H-atoms is
(a) 6.5 x 10-7 m (b) 5.6 x 10-7 m (c) 4.8 x 10-7 m (d) 4.0 x 10-7 m (iii) The ratio of kinetic energy of the electrons for the H-atoms to that of He+ ion for n = 2 is
\(\text { (a) } \frac{1}{4}\) \(\text { (b) } \frac{1}{2}\) (c) 1 (d) 2 (iv) The radius ofthe ground state orbit of H-atoms is
\(\text { (a) } \frac{\varepsilon_{0}}{h \pi m e^{2}}\) \(\text { (b) } \frac{h^{2} \varepsilon_{0}}{\pi m e^{2}}\) \(\text { (c) } \frac{\pi m e^{2}}{h}\) \(\text { (d) } \frac{2 \pi h \varepsilon_{0}}{m e^{2}}\) (v) Angular momentum of an electron in H-atom in first excited state is
\(\text { (a) } \frac{h}{\pi}\) \(\text { (b) } \frac{h}{2 \pi}\) \(\text { (c) } \frac{2 \pi}{h}\) \(\text { (d) } \frac{\pi}{h}\) (a) -
Bohr's model explains the spectral lines of hydrogen atomic emission spectrum. While the electron of the atom remains in the ground state, its energy is unchanged. When the atom absorbs one or more quanta of energy, the electrons moves from the ground state orbit to an excited state orbit that is further away.
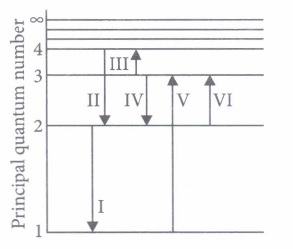
The given figure shows an energy level diagram of the hydrogen atom. Several transitions are marked as I, II, III and so on. The diagram is only indicative and not to scale.
(i) In which transition is a Balmer series photon absorbed?(a) II (b) III (c) IV (d) VI (ii) The wavelength of the radiation involved in transition II is
(a) 291 nm (b) 364 nm (c) 487 nm (d) 652 nm (iii) Which transition will occur when a hydrogen atom is irradiated with radiation of wavelength 103 nm?
(a) I (b) II (c) IV (d) V (iv) The electron in a hydrogen atom makes a transition from n = n1 to n = n2 state. The time period of the electron in the initial state is eight times that in the final state. The possible values of n1 and n2 are
(a) n1 = 4, n2 = 2 (b) n1 = 8, n2 = 2 (c) n1 = 8, n2 = 3 (d) n1 = 6, n2 = 2 (v) The Balmer series for the H-atom can be observed
(a) if we measure the frequencies of light emitted when an excited atom falls to the ground state (b) if we measure the frequencies of light emitted due to transitions between excited states and the first excited state. (c) in any transition in a H-atom (d) none of these. (a) -
The spectral series of hydrogen atom were accounted for by Bohr using the relation \(\bar{v}=R\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right)\),
where R = Rydberg constant = 1.097 x 107 m.
Lyman series is obtained when an electron jumps to first orbit from any subsequent orbit. Similarly, Balmer series is obtained when an electron jumps to 2nd orbit from any subsequent orbit, Paschen series is obtained when an electron jumps to 3rd orbit from any subsequent orbit. Whereas Lyman series lies in U.V. region, Balmer series is in visible region and Paschen series lies in infrared region. Series limit is obtained when n2 = \(\infty\)
(i) The wavelength of first spectral line of Lyman series is(a) 1215.4 \(\dot A\) (b) 1215.4 crn (c) 1215.4 m (d) 1215.4 mm (ii) The wavelength limit of Lyman series is
(a) 1215.4 \(\dot A\) (b) 511.9 \(\dot A\) (c) 951.6 \(\dot A\) (d) 911.6 \(\dot A\) (iii) The frequency of first spectral line of Balmer series is
(a) 1.097 x 107 Hz (b) 4.57 x 1014 Hz (c) 4.57 x 1015 Hz (d) 4.57 x 1016 Hz (iv) Which of the following transitions in hydrogen atoms emit photons of highest frequency?
(a) n = 1 to n = 2 (b) n = 2 to n = 6 (c) n = 6 to n = 2 (d) n = 2 to n = 1 (v) The ratio of minimum to maximum wavelength in Balmer series is
(a) 5:9 (b) 5:36 (c) 1:4 (d) 3: 4 (a) -
Hydrogen is the simplest atom of nature. There is one proton in its nucleus and an electron moves around the nucleus in a circular orbit. According to Niels Bohr, this electron moves in a stationary orbit. When this electron & I is in the stationary orbit, it emits no electromagnetic radiation. The angular momentum of the electron is quantized, i.e., mvr = \((n h / 2 \pi)\) where m = mass of the electron, v = velocity of the electron in the orbit, r = radius of the orbit and n = 1,2, 3, .... When transition takes place from Kth orbit to Jth orbit, energy photon is emitted. If the wavelength of the emitted photon is \(\lambda\)y we find that \(\frac{1}{\lambda}=R\left[\frac{1}{J^{2}}-\frac{1}{K^{2}}\right]\) where R is Rydberg's constant.
On a different planet, the hydrogen atom's structure was somewhat different from ours. The angular momentum of electron was P = 2n(h/2\(\pi\) ), i.e., an even multiple of (h/2\(\pi\)).
(i) The minimum permissible radius of the orbit will be\(\text { (a) } \frac{2 \varepsilon_{0} h^{2}}{m \pi e^{2}}\) \(\text { (b) } \frac{4 \varepsilon_{0} h^{2}}{m \pi e^{2}}\) \(\text { (c) } \frac{\varepsilon_{0} h^{2}}{m \pi e^{2}}\) \(\text { (d) } \frac{\varepsilon_{0} h^{2}}{2 m \pi e^{2}}\) (ii) In our world, the velocity of electron is V0 when the hydrogen atom is in the ground state. The velocity of electron in this state on the other .planet should be
(a) V0 (b) V0/2 (c) V0/4 (d) V0/8 (iii) In our world, the ionization potential energy of a hydrogen atom is 13.6 ev On the other planet, this ionization potential energy will be
(a) 13.6 eV (b) 3.4 eV (c) 1.5 eV (d) 0.85 eV (iv) Check the correctness of the following statements about the Bohr model of hydrogen atom
(i) The acceleration of the electron in n = 2 orbit is more than that in n = 1 orbit.
(ii) The angular momentum of the electron in n = 2 orbit is more than that in n = 1 orbit.
(iii) The kinetic energy of the electron in n = 2 orbit is less than that in n = 1 orbit.(a) Only (iii) and (i) are correct. (b) Only (i) and (ii) are correct. (c) Only (ii) and (iii) are correct. (d) All the statements are correct (v) In Bohr's model of hydrogen atom, let PE represent potential energy and TE the total energy. In going to a higher orbit
(a) PE increases, TE decreases (b) PE decreases, TE increases (c) PE increases, TE increases (d) PE decreases, TE decreases (a) -
Hydrogen spectrum consists of discrete bright lines in a dark background and it is specifically known as hydrogen emission spectrum. There is one more type of hydrogen spectrum that exists where we get dark lines on the bright background, it is known as absorption spectrum. Balmer found an empirical formula by the observation of a small part of this spectrum and it is represented by \(\frac{1}{\lambda}=R\left(\frac{1}{2^{2}}-\frac{1}{n^{2}}\right), \text { where } n=3,4,5, \ldots .\)
For Lyman series, the emission is from first state to nth state, for Paschen series, it is from third state to nth state, for Brackett series, it is from fourth state to nth state and for Pfund series, it is from fifth state to nth state,
(i) Number of spectral lines in hydrogen atom is(a) 8 (b) 6 (c) 15 (d) \(\infty\) (ii) Which series of hydrogen spectrum corresponds to ultraviolet region?
(a) Balmer series (b) Brackett series (c) Paschen series (d) Lyman series (iii) Which of the following lines of the H-atom spectrum belongs to the Balmer series?
(a) 1025 \(\dot A\) (b) 1218 \(\dot A\) (c) 4861 \(\dot A\) (d) 18751 \(\dot A\) (iv) Rydberg constant is
(a) a universal constant (b) same for same elements (c) different for different elements (d) none of these (v) Hydrogen atom is excited from ground state to another state with principal quantum number equal to 4. Then the number of spectral lines in the emission spectra will be
(a) 3 (b) 5 (c) 6 (d) 2 (a)
*****************************************
CBSE 12th Standard Physics Subject Atoms Case Study Questions With Solution 2021 Answer Keys
-
(i) (c)
(ii) (d): \(\omega=\frac{v}{r} . \text { Further } v \propto \frac{1}{n} \text { and } r \propto n^{2},\)
\(\text { Hence } \omega \propto\left(1 / n^{3}\right)\)
(iii) (c)
(iv) (b): The energy of nth Bohr orbit in hydrogen atom is
\(E_{n}=-\frac{13.6}{n^{2}} \mathrm{eV}\)
For lowest orbit, n = 1
\(\therefore \quad E_{1}=-13.6 \mathrm{eV}\)
Thus, the lowest Bohr orbit in hydrogen atom has the least energy
(v) (b) -
(i) (d) : Rutherford's atom had a positively charged centre and electrons were revolving outside it.It is also called the planetary model of the atom as in option (d).
(ii) (d): As the gold foil is very thin, it can be assumed that a-particles will suffer not more than one scattering during their passage through it. Therefore, computation of the trajectory of an a-particle scattered by a single nucleus is enough.
(iii) (c) : Trajectory of a-particles depends on impact parameter which is the perpendicular distance of the initial velocity vector of the a particles from the centre of the nucleus. For small impact parameter a particle close to the nucleus suffers larger scattering.
(iv) (b): At minimum impact parameter, a particles rebound back (\(\theta\) = \(\pi\)) and suffers large scattering.
(v) (d): In case of head-on-collision, the impact parameter is minimum and the a-particle rebounds back. So, the fact that only a small fraction of the number of incident particles rebound back indicates that the number of a-particles undergoing head-on collision is small. This in turn implies that the mass of the atom is concentrated in a small volume. Hence, option (a) and (b) are correct. -
(i) (c) : \(E_{n}=\frac{-13.6}{n^{2}}\left(Z^{2}\right)\)
In first excited state \(E_{\mathrm{H}_{2}}=3.4 \mathrm{eV} \text { and } E_{\mathrm{He}}=-13.6 \mathrm{eV}\)
So, H2 atom gives excitation energy
(13.6- 3.4 = 10.2 eV) to helium atom
Now, energy of He ion = -13.6 + 10.2 = -3.4 eV
Again, \(E=\frac{-13.6}{n^{2}} \times Z^{2}\)
\(\Rightarrow \quad-3.4=\frac{-13.6}{n^{2}} \times(2)^{2} \Rightarrow n=4\)
(ii) (c): \(\frac{1}{\lambda}=\frac{13.6 Z^{2}}{h c}\left[\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right]\)
Here, \(n_{1}=3 \text { and } n_{2}=4 \Rightarrow \lambda=4.8 \times 10^{-7} \mathrm{~m}\)
(iii) (a): \(\text { Kinetic energy, } K \propto \frac{Z^{2}}{n^{2}}\)
\(\frac{K_{\mathrm{H}_{2}}}{K_{\mathrm{He}}}=\left(\frac{Z_{\mathrm{H}_{2}}}{Z_{\mathrm{He}}}\right)^{2}=\left(\frac{1}{2}\right)^{2}=\frac{1}{4}\)
(iv) (b): Radius of the permitted orbit is \(r=\frac{n^{2} h^{2} \varepsilon_{0}}{\pi m Z e^{2}}\) For hydrogen atom in ground state, i.e.,|
\(n=1, Z=1 \Rightarrow r=\frac{h^{2} \varepsilon_{0}}{\pi m e^{2}}\)
(v) (a): Angular momentum for hydrogen atom is
\(L=\frac{n h}{2 \pi}\)
For first excited state \(n=2, \quad L=\frac{h}{\pi}\) -
(i) (d): For Balmer series, n1 = 2; n2 = 3, 4, ...
(lower) (higher)
Therefore, in transition (VI), photon of Balmer series is absorbed.
(ii) (c): In transition II
\(E_{2}=-3.4 \mathrm{eV}, E_{4}=-0.85 \mathrm{eV}\)
\(\Delta E=2.55 \mathrm{eV} \Rightarrow \Delta E=\frac{h c}{\lambda} \Rightarrow \lambda=\frac{h c}{\Delta E}=487 \mathrm{nm}\)
(iii) (d): Wavelength of radiation = 1030 \(\dot A\)
\(\Delta E=\frac{12400}{1030 \dot A}=12.0 \mathrm{eV}\)
So, difference of energy should be 12.0 eV (approx.) Hence for n1 = 1 to n2 = 3.
\(E_{n_{3}}-E_{n_{1}}=-1.51 \mathrm{eV}-(-13.6 \mathrm{eV}) \approx 12 \mathrm{eV}\)
Therefore, transition V will occur.
(iv) (a): \(T^{2} \propto r^{3} \text { and } r \propto n^{2} \Rightarrow T^{2} \propto n^{6} \Rightarrow T \propto n^{3}\)
\(\frac{T_{1}}{T_{2}}=\left(\frac{n_{1}}{n_{2}}\right)^{3} \Rightarrow 8=\left(\frac{n_{1}}{n_{2}}\right)^{3} \text { or } \frac{n_{1}}{n_{2}}=2\)
(v) (b) -
(i) (a) : From, \(\bar{v}-\frac{1}{\lambda}-R\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right)\)
n1 = 1, n2 = 2 for first spectral line of Lyman series,
\(\frac{1}{\lambda}=1.097 \times 10^{7}\left(\frac{1}{1^{2}}-\frac{1}{2^{2}}\right)=\frac{3 \times 1.097 \times 10^{7}}{4} \mathrm{~m}^{-1}\)
\(\lambda=\frac{4 \times 10^{-7} \mathrm{~m}}{3 \times 1.097}=\frac{4000}{3 \times 1.097} \dot A=1215.4 \dot A\)
(ii) (d): For wavelength limit, we put \(n_{2}=\infty\)
\(\therefore \quad \frac{1}{\lambda}=1.097 \times 10^{7}\left(\frac{1}{1^{2}}-\frac{1}{\infty}\right)\)
\(\lambda=\frac{1}{1.097 \times 10^{7}} \mathrm{~m}=\frac{1000}{1.097} \dot A=911.6 \dot A\)
(iii) (b): For first line of Balmer series, n1 = 2, n2 = 3
\(\bar{v}=\frac{1}{\lambda}=R\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right)\)
\(v=\frac{c}{\lambda}=R c\left(\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right)\)
\(=1.097 \times 10^{7} \times 3 \times 10^{8}\left(\frac{1}{2^{2}}-\frac{1}{3^{2}}\right)\)
\(=1.097 \times 3 \times 10^{15} \times \frac{5}{36}=4.57 \times 10^{14} \mathrm{~Hz}\)
(iv) (d): \(h v_{2 \rightarrow 1}=-13.6\left(\frac{1}{2^{2}}-\frac{1}{1^{2}}\right) \mathrm{eV}=10.2 \mathrm{eV}\)
Emission is n = 2 \(\rightarrow\) n = 1 i.e., higher n to lower n.Transition from lower to higher levels are absorption lines.
\(-13.6\left(\frac{1}{6^{2}}-\frac{1}{2^{2}}\right)=+13.6 \times \frac{2}{9}\)
This is \(
(v) (a): \(\frac{1}{\lambda_{\max }}=R\left[\frac{1}{2^{2}}-\frac{1}{3^{2}}\right]=\frac{5 R}{36}\)
\(\frac{1}{\lambda_{\min }}=R\left[\frac{1}{2^{2}}-\frac{1}{\infty}\right]=\frac{R}{4} \Rightarrow \frac{\lambda_{\min }}{\lambda_{\max }}=\frac{5 R}{36} \times \frac{4}{R}=\frac{5}{9}\)
-
(i) (b): \(\text { On other planet }: m v r=2 n \frac{h}{2 \pi} \Rightarrow v=\frac{n h}{\pi m r}\)
\(\frac{m v^{2}}{r}=\frac{1}{4 \pi \varepsilon_{0}} \frac{e^{2}}{r^{2}} \Rightarrow \frac{m n^{2} h^{2}}{n^{2} m^{2} r^{3}}=\frac{1}{4 \pi \varepsilon_{0}} \frac{e^{2}}{r^{2}}\)
Putting n = 1, we get r \( =\frac{4 h^{2} \varepsilon_{0}}{m \pi e^{2}}\)
(ii) (b): \(\text {On our planet: } v_{0}=\frac{e^{2}}{2 \varepsilon_{0} n h}\)
\(\text {On other planet : } v=\frac{e^{2}}{2 \varepsilon_{0}(2 n) h}=\frac{v_{0}}{2}\)
(iii) (b): On our planet \(E_{n}=-\frac{13.6}{n^{2}}\)
On other planet \(E_{n}^{\prime}=-\frac{13.6}{(2 n)^{2}}\)
\(\Rightarrow \quad E_{n}^{\prime}=\frac{E_{n}}{4}=-3.4 \mathrm{eV}\)
(iv) (c): Centripetal acceleration = mv2/r Further, as n increases, r also increases. Therefore, centripetal acceleration for n = 2 is less than that for n = 1. So, statement (i) is wrong. Statement (ii) and (iii) are correct.
(v) (c): Potential energy = -C/r2 and total energy = -Rhc/n2. With higher orbit, both rand n increase. So, both become less negative; hence both increase. -
(i) (d) : Number of spectral lines in hydrogen atom is \(\infty\)
(ii) (d): Lyman series lies in the ultraviolet region.
(iii) (c) : The shortest Balmer line has energy
\(=|(3.4-1.51)| \mathrm{eV}=1.89 \mathrm{eV}\)
and the highest energy = I(0 - 3.4)I = 3.4 eV The corresponding wavelengths are
\(\frac{12400 \mathrm{eV} \dot A}{1.89 \mathrm{eV}}=6561 \dot A \text { and } \frac{12400 \mathrm{eV} \dot A}{3.4 \mathrm{eV}}=3647 \dot A\)
Only 4861 \(\dot A\) is between the first and last line of the Balmer series.
(iv) (a)
(v) (c)






































