CBSE 12th Standard Physics Subject Atoms HOT Questions 3 Mark Questions With Solution 2021
By QB365 on 27 May, 2021
QB365 Provides the HOT Question Papers for Class 12 Physics, and also provide the detail solution for each and every HOT Questions. HOT Questions will help to get more idea about question pattern in every exams and also will help to get more marks in Exams
QB365 - Question Bank Software
CBSE 12th Standard Physics Subject Atoms HOT Questions 3 Mark Questions With Solution 2021
12th Standard CBSE
-
Reg.No. :
Physics
-
A point source of \(\gamma \)-radiation has a half life of 30 minutes. The initial count rate, recorded by Geiger counter placed 2 m from the source is \(360{ s }^{ -1 }.\) The distance between the counter and the source is altered. After 1.5 hours, the count rate recorded in \(5{ s }^{ -1 }.\) What is the new distance between the counter and the source?
(a) -
Consider a radioactive nucleus A which decays to a stable nucleus C through the following sequence:
A \(\longrightarrow \) B \(\longrightarrow \) C
where, B is an intermediate nuclei, which is also radioactive. Considering that there are N0 atoms of A initially, plot the graph showing the variation of number of atoms of A and B versus time.(a) -
At atomic power nuclear reactor can deliver 300 MW. The energy released due to fission of each nucleus of uranium atoms U238 is 170 MeV. What will be the number of atoms fissioned per hour?
(a) -
(i) Using Bohr's second postulate of quantisation of orbital angular momentum, show that the circumference of the electron in the nth orbital state in H-atom is n times the de-Broglie wavelength associated with it.
(ii) The electron in H-atom is initially in the third excited state. What is the maximum number of spectral lines which can be emitted when it finally moves to the ground state?(a) -
Nuclei with magic number of protons Z = 2,8,20,28,50,52 and magic number of neutrons N = 2,8,20,28,5082 and 126 are found to be very stable.
(i) Verify this by calculating the proton separation energy \({ S }_{ p }\) for \({ 120 }_{ Sn }(Z=50)\) and \({ 121 }_{ Sn }(Z=51)\) .The proton separation energy for a nuclide is the minimum energy required to separate the least tightly bound proton from a nucleus of that nuclide.It is given by
\({ S }_{ P }=({ M }_{ Z-1,N }+{ M }_{ H }-{ M }_{ Z,N }){ c }^{ 2 }\)
\(given,{ 119 }_{ In }=118.9058 \ u,\)
\( { 120 }_{ Sn }=119.902199 \ u,\)
\({ 121 }_{ Sb }=120.903824 \ u,\)
\( { 1 }_{ H }=1.0078252u\)
(ii) What does the existence of magic number indicate?(a)
*****************************************
CBSE 12th Standard Physics Subject Atoms HOT Questions 3 Mark Questions With Solution 2021 Answer Keys
-
Here, \(T=30 \ mins.=0.5h, \ t=1.5hr. \ \therefore \ n=\frac { t }{ T } =\frac { 1.5 }{ 0.5 } =3\)
When distance is changed, count rate after 3 half lives is \(5{ s }^{ -1 }.\) Therefore, count rate at the beginning of 1.5 hr. \(={ \left( 2 \right) }^{ 3 }\times 5=40{ s }^{ -1 },\) which is \(\frac { 1 }{ 9 } \times 360{ s }^{ -1 }=\frac { 1 }{ 9 } th\) of original count rate. i.e. Intensity has been reduced by a factor of 9. As intensity \(\propto \frac { 1 }{ { \left( dist \right) }^{ 2 } } ,\) therefore, distance of source from counter must have increased by a factor of 3 i.e. New distance \(=3\times 2=6m\) -
By considering the situation given in the question,
At t = 0, NA = N0 (maximum), while NB = 0. As time increases, NA decreases exponentially and the number of atoms of B increases. They become (NB) maximum and finally drop to zero exponentially by radioactive decay law. So, graph showing the variation of number of atoms of A and B will be shown as below:
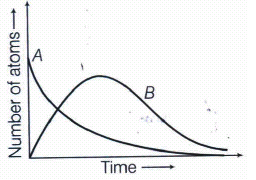
-
As, we know that
\(power=\frac { energy }{ time } =300\times { 10 }^{ 6 }W=3\times { 10 }^{ 8 }J/s\)
\( 170 \ MeV \ =170\times { 10 }^{ 6 }\times 1.6\times { 10 }^{ -19 }=27.2\times { 10 }^{ -12 }J\)
Number of atoms fissioned per second
\(=\frac { 3\times { 10 }^{ 8 } }{ 2.72\times { 10 }^{ -12 } } =\frac { 3\times { 10 }^{ 20 } }{ 27.2 } \)
Number of atoms fissioned per hour
\(=\frac { 3\times { 10 }^{ 20 }\times 3600 }{ 2.72 } =\frac { 3\times 36 }{ 27.2 } \times { 10 }^{ 22 }=4\times { 10 }^{ 22 }m\) -
(i) Bohr's second postulate states that the electron revolves around the nucleus in certain privileged orbit which satisfy certain quantum condition that angular momentum of an electron is an integral multiple of \(\cfrac { h }{ 2\pi } \), where h is Planck's constant. i.e \(L=mvr=\cfrac { nh }{ 2\pi } \)
Where, m = mass of electron, v = speed of electron and r = radius of orbit of electron.
\(\therefore\) Circumference of electron in nth orbit
= n \(\times\)de-Broglie wavelength associated with electron. \(\left[ \because \lambda =\cfrac { h }{ mv } \right] \)
(ii) Given, the electron in H-atom is initially in third excited state.
n = 4
And the total number of spectral lines of an atom that can exist is given by the relation
\(=\ \cfrac { n(n-1) }{ 2 } \)
Here, n = 4
So, number of spectral lines = \(\cfrac { 4(4-1) }{ 2 } =\cfrac { 4\times 3 }{ 2 } =6\)
Hence, when a H-atom moves from third excited state to ground state, it emits six spectral lines. -
(i) The proton separation energy is given by
\({ S }_{ P }(sn)=({ M }_{ 119,70 }+{ M }_{ H }-{ M }_{ 120,70 }){ c }^{ 2 }\)
\(=(118.902199+1.0078252-119.902199){ C }^{ 2 }\)
\(=0.0114262{ C }^{ 2 }\)
\(Similarly,{ S }_{ p }(sb)=({ M }_{ 120,70 }+{ M }_{ H }-{ M }_{ 121,70 }){ c }^{ 2 }\)
\(=(119.902199+1.0078252-120.903822){ C }^{ 2 }\)
\(=0.006202 \ { c }^{ 2 }\)
\(Since,{ S }_{ p }(sn)>{ S }_{ p }(sb), \) Sn nucleus is more stable than sb nucleus.
(ii) The existence of magic number indicates that the shell structure of nucleus is similar to the shell structure of an atom. This also explains the peaks in binding energy/nucleon curve.






































