Class 11th Applied Mathematics - Coordinate Geometry Case Study Questions and Answers 2022 - 2023
By QB365 on 09 Sep, 2022
QB365 provides a detailed and simple solution for every Possible Case Study Questions in Class 11 Applied Mathematics Subject - Coordinate Geometry, CBSE. It will help Students to get more practice questions, Students can Practice these question papers in addition to score best marks.
QB365 - Question Bank Software
Coordinate Geometry Case Study Questions With Answer Key
11th Standard CBSE
-
Reg.No. :
Applied Mathematics
-
A market is in the form of a triangle whose vertices are B(–2, 0), C(1, 12). The third vertex A of this trianagle lies on the mid point of the line joining the points (2, 1) and (4, 13).
On the basis of this information answer the following questions:
(i) What will be the coordinates of A ?(a) (5, 8) (b) (3, 7) (c) (3, 9) (d) (4, 8) (ii) Find the slope of the line joining the points B and C?
(a) 4 (b) 3 (c) 2 (d) 1 (iii) Equation of the line joining the points B and C?
(a) y – 2 = 4 x (b) y + 2 = 5x (c) y + 2 = 3x (d) y + 2 = x (iv) Does point A lies on the line BC?
(a) it lies on the line BC (b) it will not lie on the line BC (c) can’t say (d) None of these (v) If slope between two lines are – 2 and \(\frac{1}{2}\) then these lines are
(a) parallel (b) perpendicular (c) coincident lines (d) Perpendicular (a) -
A farming land is in the form of a quadrilateral. Vertices of this quadrilateral are A(1, 3), B(8, 3), C(8, 16), and D(16, 1).
On the basis of this information answer the following questions:
(i) Slope of side AB is(a) 1 (b) \(\frac{1}{2}\) (c) 0 (d) \(\frac{1}{3}\) (ii) Slope of side AC is
(a) \(\frac{1}{3}\) (b) \(\frac{13}{14}\) (c) \(\frac{13}{14}\) (d) \(\frac{2}{3}\) (iii) If the slope of the line is zero, then such line is
(a) parallel to x-axis (b) parallel to y-axis (c) none of these (iv) Equation of line AC is
(a) 13x + y = – 21 (b) 3x + y = – 11 (c) 3x – y = – 21 (d) 13x – 7y = –8 (v) If slope of line L1 is m1 and slope of line L2 is m2 then angle between two lines is
(a) \(\tan ^{-1}\left(\frac{m_{2}-m_{1}}{1+m_{1} m_{2}}\right)\) (b) \(\tan ^{-1}\left(\frac{m_{2}+m_{1}}{1+2 m_{1} m_{2}}\right)\) (c) \(\tan ^{-1}\left(\frac{m_{2}+m_{1}}{2-2 m_{1} m_{2}}\right)\) (d)\(\tan ^{-1}\left(\frac{m_{2}+m_{1}}{1-m_{1} m_{2}}\right)\) (a) -
A student was standing at point P (as shown in figure. From point P, he was looking at a sphere at two different points T and R. O is the centre of the sphere. Given ∠TPR = 60° and distance between point P and O is \(a \sqrt{3}\).
On the basis of the given information answer the following questions :
(i) What is the measure of the angle OTP?(a) 30° (b) 75° (c) 45° (d) 90° (ii) What is the measure of angle OPT?
(a) 45° (b) 35° (c) 30° (d) 60° (iii) The value of OP in terms of a is
(a) 3a (b) 2a (c) 4a (d) a (iv) The value of PT in terms of a is
(a) \(\sqrt{2} a\) (b) a (c) \(\sqrt{3} a\) (d) \(\sqrt{4} a\) (v) If OT = 2 units and coordinates of centre are (0,0). then equation of circle is
(a) x2 + y2 = 6 (b) x2 – y2 = 4 (c) 2x2 + 2y2 = 9 (d) x2 + y2 = 4 (a) -
A farmer has a circular field in which a square area is there. In that square area, he was able to crop the field and rest of the area outside the square was not good for crops.
On the basis of this information, answer the following questions:
(i) If the coordinates of centre of the circle are (2, – 3), then which of the following equation of the diameter will pass through the centre?(a) x + 2y = –1 (b) x + y = – 1 (c) x + y = 2 (d) x – y = – 1 (ii) If the centre of the circle is (2, –3) and radius is 8 then equation of circle is
(a) x2 – y2 – x + 3y – 41 = 0 (b) x2 + y2 – x + y – 51 = 0 (c) x2 + y2 – 4x – 5y – 51 = 0 (d) x2 + y2 – 4x + 6y – 51 = 0 (iii) If radius of circle is double, then its area will
(a) remain same (b) double (c) three times (d) four times (iv) If the square has side 2m, then difference between the area of circle and square is
(a) \(2 \pi+4\) (b) \(2 \pi-4\) (c) \(2 \pi-1\) (d) \(2 \pi-2\) (v) Line which is perpendicular to the tangent will always pass through the centre of the circle, this statement is
(a) true (b) false (c) can’t say (d) none of these (a) -
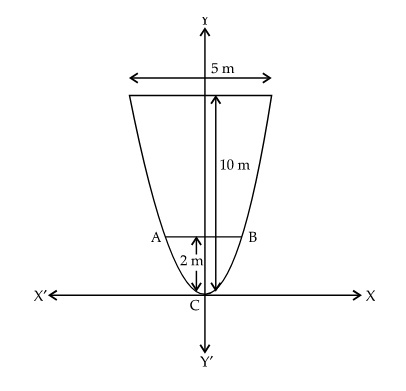
Rahul is playing with long string, he hang the ends of the string at two points on the wall. Now, it is in the form of parabola with its vertical axis and is 10m high and 5 m wide at its base as shown in the following figure:
On the basis of the above information answer the following questions:
(i) What is the standard equation of parabola in this case ?(a) y2 = 4ax (b) y2 = - 4ax (c) x2 = 4 ay (d) x2 = - 4 ay (ii) Parabola passes through the point :
(a) \(\left(\frac{5}{2}, 10\right)\) (b) \(\left(10, \frac{5}{2}\right)\) (c) \(\left(0, \frac{5}{2}\right)\) (d) \(\left(\frac{5}{2}, 0\right)\) (iii) Find the value of a in the standard equation
(a) \(\frac{32}{5}\) (b) \(\frac{5}{32}\) (c) \(\frac{5}{2}\) (d) \(\frac{2}{5}\) (iv) What is particular equation of parabola ?
(a) x2 = 5y (b) x2 = 8y (c) \(x^{2}=\frac{5}{8} y\) (d) x2 = y (v) How wide is it 2 m from the vertex of the parabola ?
(a) 2m (b) 3m (c) 2.23 m (d) 2.5 m (a) -
The cable of a uniformly loaded suspension bridge hangs in the form of a parabola. The roadway which is horizontal and 100 m long is supported by vertical wires attached to the cable, the longest wire being 30 m and the shortest being 6 m. A supporting wire is also attached to the roadway 18 meter from the middle. (see figure below)
On the basis of the above information answer the following questions:
(i) What is the standard equation of parabola in this case ?(a) y2 = 4ax (b) y2 = - 4ax (c) x2 = 4 ay (d) x2 = - 4 ax (ii) What are the coordinates of point A ?
(a) (50, 30) (b) (50, 24) (c) (30,50) (d) (24, 50) (iii) Find the value of a in the standard equation
(a) \(\frac{24}{625}\) (b) \(\frac{18}{625}\) (c) \(\frac{625}{24}\) (d) \(\frac{625}{18}\) (iv) What is particular equation of parabola ?
(a) x2 = 25y (b) x2 = -24y (c) x2 = 625y (d) 6x2 = 625y (v) Find the length of a supporting wire attached to the roadway 18 m from the middle
(a) 3.11 m (b) 6 m (c) 9.11 m (d) none of these (a)
Case Study
*****************************************
Answers
Coordinate Geometry Case Study Questions With Answer Key Answer Keys
-
(i) (b): (3, 7)
Midpoint of (2, 1) and (4, 13) is given by (3, 7).
(ii) (a): 4
\(\text { Slope }=\frac{12-0}{1-(-2)}=\frac{12}{3}=4\)
(iii) (a): y – 2 = 4x
Equation of BC: = (y – 0) = 4(x + 2)
= y – 2 = 4x
(iv) (b): it will not lie on the line BC.
Equation of BC is y – 2 – 4 x = 0. Putting coordinates of A (3, 7) in this equation, we get: 7 – 2 – 12 ≠ 0, therefore we can say that point A will not lie on the line BC.
(v) (b): perpendicular
The product of the slopes is \(-2 \times \frac{1}{2}=-1\) -
(i) (c): 0
\(\text { Slope of } A B=\frac{3-3}{8-1}=0\)
(ii) (c): \(\frac{13}{14}\)
\(\text { Slope of } A C=\frac{16-3}{8-1}=\frac{13}{7}\)
(iii) (a): parallel to x-axis
If slope of a line is zero, then the line is parallel to x-axis.
(iv) (d): 13x – 7y = –8
\(\text { Equation of line } \mathrm{AC}: y-3=\frac{16-3}{8-1}(x-1)\)
⇒ 7y – 21 = 13x – 13
⇒ 13 x – 7y = – 8
(v) (a): \(\tan ^{-1}\left(\frac{m_{2}-m_{1}}{1+m_{1} m_{2}}\right)\)
Angle between two lines, then
\(\tan \theta=\frac{m_{2}-m_{1}}{1+m_{1} m_{2}}\)
\(\Rightarrow \theta=\tan ^{-1}\left(\frac{m_{2}-m_{1}}{1+m_{1}+m_{2}}\right)\) -
(i) (d): 90°
Angle formed from the center to the tangent of the circle is 90°.
(ii) (c):30°
Since, triangle OPT and OPRare congruent, therefore OP is angle bisector of angle TPR. Therefore, angle OPT = 30°.
(iii) (b): 2a
In triangle OPT,
\(\sin 30^{\circ}=\frac{O T}{O P}\)
\(\Rightarrow \frac{1}{2}=\frac{a}{O P}\)
⇒ OP = 2a
(iv) (c): \(\sqrt{3} a\)
By using pythagorus theorem in triangle OPT, we get,
OP2 = OT2 + PT2
\(\Rightarrow P T=\sqrt{O P^{2}-O T^{2}}\)
= \(\sqrt{(2 a)^{2}-a^{2}}\)
= \(\sqrt{3} a\)
(v) (d): x2 + y2 = 4
Equation of circle: (x – 0)2 + (y – 0)2 = 22
⇒x2 + y2 = 4 -
(i) (b): x + y = – 1
Coordinates which satisfy the equation of the line is x + y = –1.
(ii) (d): x2 + y2 – 4 x + 6 y – 51 = 0
Equation of circle : (x – 2)2 + (y + 3)2 = 82x2 + y2 – 4x + 6y – 51 = 0.
(iii) (d): four times
Let the radius = a, then area = \(\pi a^{2}\) If the radius be double, then area = \(\pi(2 a)^{2}=4(\pi 4 a)^{2}\). Therefore change in area is four times.
(iv) (b): \(2 \pi-4\)
Area of square = 4 m2
Area of circle = \(\pi(\sqrt{2})^{2}=2 \pi\)
Required area = \(2 \pi-4\)
(v) (a): Any line perpendicular to the tangent of the circle will always pass through the centre of the circle. -
(i) (c): x2 = 4 ay
The equation of the parabola is of the form x2 = 4 ay (as it opening upwards).
(ii) (a): \(\left(\frac{5}{2}, 10\right)\)
It can be clearly seen from the given figure that the parabola passes through point \(\left(\frac{5}{2}, 10\right)\)
(iii) (b): \(\frac{5}{32}\)
It can be clearly seen that the parabola passes through point \(\left(\frac{5}{2}, 10\right)\)
\( \left(\frac{5}{2}\right)^{2} =4 a(10) \)
\(\Rightarrow \ a =\frac{25}{4 \times 4 \times 10} \)
\(=\frac{5}{32} \)
(iv) (c): \(x^{2}=\frac{5}{8} y\)
The equation of parabola is
x2 = 4ay
\(x^{2}=4\left(\frac{5}{32}\right) y=\left(\frac{5}{8}\right) y\)
(v) (c): 2.23 m
Since, the equation of parabola is
\(x^{2}=\frac{5}{8} y\)
\( \Rightarrow \ \mathrm{AB} =2 \times \sqrt{\frac{5}{4}} \mathrm{~m} \)
\(=\sqrt{5} \mathrm{~m} \)
= 2.23 m -
(i) (c): x2 = 4ay
The equation of the parabola is of the form x2 = 4ay(as it is upwards).
(ii) (b): (50, 24)
Here, AB = 30 m, OC = 6 m, and BC = \(=\frac{100}{2}=50 \mathrm{~m}\)
The coordinate of point A are (50, 30 – 6)
= (50, 24)
(iii) (c): \(\frac{625}{24}\)
Since A (50, 24) is a point on the parabola.
(50)2 = 4a(24)
\( \Rightarrow \ a =\frac{50 \times 50}{4 \times 24} \)
\(=\frac{625}{24}\)
(iv) (d): 6x2 = 625y
Equation of the parabola,
\(x=4 \times \frac{625}{24} \times y\)
6x2 = 625y
(v) (c): 9.11 m
The x-coordinate of point D is 18.
Hence, at x = 18,
6(18)2 = 625y
\(\Rightarrow \ y=\frac{6 \times 18 \times 18}{625}\)
⇒ y = 3.11 (approx.)
DE = 3.11 m
DF = DE + EF
= 3.11 m + 6 m
= 9.11 m
Case Study



































