Class 12th Physics - Ray Optics and Optical Instruments Case Study Questions and Answers 2022 - 2023
By QB365 on 09 Sep, 2022
QB365 provides a detailed and simple solution for every Possible Case Study Questions in Class 12th Physics Subject - Ray Optics and Optical Instruments, CBSE. It will help Students to get more practice questions, Students can Practice these question papers in addition to score best marks.
QB365 - Question Bank Software
Ray Optics and Optical Instruments Case Study Questions With Answer Key
12th Standard CBSE
-
Reg.No. :
Physics
-
A convex or converging lens is thicker at the centre than at the edges. It converges a parallel beam of light on refraction through it. It has a real focus. Convex lens is of three types:
(i) Double convex lens
(ii) Plano-convex lens
(iii) Concavo-convex lens. Concave lens is thinner at the centre than at the edges. It diverges a parallel beam of light on refraction through it. It has a virtual focus.
(i) A point object 0 is placed at a distance of 0.3 m from a convex lens (focal length 0.2 m) cut into two halves each of which is displaced by 0.0005 m as shown in figure.What will be the location of the image?
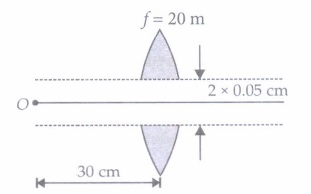
(a) 30 cm right of lens (b) 60 ern right of lens (c) 70 ern left of lens (d) 40 cm left oflens (ii) Two thin lenses are in contact and the focal length of the combination is 80 cm. If the focal length of one lens is 20 cm, the focal length of the other would be
(a) -26.7 cm (b) 60 crn (c) 80 cm (d) 20 cm (iii) A spherical air bubble is embedded in a piece of glass. For a ray of light passing through the bubble, it behaves like a
(a) converging lens (b) diverging lens (c) plano-converging lens (d) plano-diverging lens (iv) Lens used in magnifying glass is
(a) Concave lens (b) Convex lens (c) Both (a) and (b) (d) None of the above (v) The magnification of an image by a convex lens is positive only when the object is placed
(a) at its focus F (b) between F and 2F (c) at 2F (d) between F and optical centre (a) -
Power (P) of a lens is given by-reciprocal of focal length (f) of the lens i.e., \(P=\frac{1}{f}\) where fis in metre and P is in dioptre. For a convex lens, power is positive and for a concave lens, power is negative. When a number of thin lenses of powers P1, P2, P3, .....are held in contact with one another, the power of the combination is given by algebraic sum of the powers of all the lenses i.e., P = P1 + P2 + P3 + ....
(i) A convex and a concave lens separated by distance d are then put in contact. The focal length of the combination(a) becomes 0 (b) remains the same (c) decreases (d) increases. (ii) If two lenses of power +1.5D and +1.0D are placed in contact, then the effective power of combination will be
(a) 2.5 D (b) 1.5D (c) 0.5 D (d) 3.25 D (iii) If the power of a lens is +5 dioptre, what is the focal length of the lens?
(a) 10 crn (b) 20 cm (c) 15 crn (d) 5 cm (iv) Two thin lenses offocallengths +10 cm and -5 em are kept in contact. The power of the combination is
(a) -10 D (b) -20 D (c) 10 D (d) 15 D (v) A convex lens of focal length 25 cm is placed coaxially in contact with a concave lens of focal length 20 cm. The system will be
(a) converging in nature (b) diverging in nature (c) can be converging or diverging (d) None of the above (a) -
Total internal reflection is the phenomenon of reflection of light into denser medium at the interface of denser medium with a rarer medium. For this phenomenon to occur necessary condition is that light must travel from denser to rarer and angle of incidence in denser medium must be greater than critical angle (C) for the pair of media in contact. Critical angle depends on nature of medium and wavelength of light. We can show that
\(\mu=\frac{1}{\sin C} .\)
(i) Critical angle for glass air interface, where \(\mu\) of glass is \(\frac{3}{2}\) is(a) 41.8° (b) 60° (c) 30° (d) 15° (ii) Critical angle for water air interface is 48.6°. What is the refractive index of water?
(a) 1 (b) \(\frac{3}{2}\) (c) \(\frac{4}{3}\) (d) \(\frac{3}{4}\) (iii) Critical angle for air water interface for violet colour is 49°. Its value for red colour would be
(a) 49° (b) 50° (c) 48° (d) cannot say (iv) Which of the following is not due to total internal reflection?
(a) Working of optical fibre. (b) Difference between apparent and real depth of a pond. (c) Mirage on hot summer days. (d) Brilliance of diamond (v) Critical angle of glass is \(\theta\)1 and that of water is \(\theta\)2, The critical angle for water and glass surface would be \(\left(\mu_{g}=3 / 2, \mu_{w}=4 / 3\right)\)
(a) less than \(\theta\)2 (b) between \(\theta\)1 and \(\theta\)2 (c) greater than \(\theta\)2 (d) less than \(\theta\)1 (a) -
The lens maker's formula is a relation that connects focal length of a lens to radii of curvature of two surfaces of the lens and refractive index of the material of the lens. It is \(\frac{1}{f}=(\mu-1)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)\) where \(\mu\) is refractive index oflens material w.r.t. the medium in which lens is held.As ,\(\mu_{v}>\mu_{r}\) therefore \(f_{r}>f_{v^{\prime}}\) Mean focal length of lens for yellow colour is \(f=\sqrt{f_{r} \times f_{v}}\).
(i) Focal length of a equiconvex lens of glass \(\mu=\frac{3}{2}\) in air is 20 cm. The radius of curvature of each surface is(a) 10cm (b) -10 crn (c) 20 crn (d) -20 cm (ii) A substance is behaving as convex lens in air and concave in water, then its refractive index is
(a) greater than air but less than ater (b) greater than both air and water (c) smaller than air (d) almost equal to water (iii) For a thin lens with radii of curvatures R1 and R2, refractive index nand focal length f, the factor \(\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)\) is equal to
\((a) \frac{1}{f(n-1)}\) \((b) f(n-1)\) \((c) \frac{(n-1)}{f}\) \((d) \frac{n}{f(n-1)}\) (iv) A given convex lens of glass \(\left(\mu=\frac{3}{2}\right)\) can behave as concave when it is held in a medium of \(\mu\) equal to
(a) 1 \(\text { (b) } \frac{3}{2}\) \(\text { (c) } \frac{2}{3}\) \(\text { (d) } \frac{7}{4}\) (v) The radii of curvature of the surfaces of a double convex lens are 20 cm and 40 cm respectively, and its focal length is 20 cm. What is the refractive index of the material of the lens?
\(\text { (a) } \frac{5}{2}\) \(\text { (b) } \frac{4}{3}\) \(\text { (c) } \frac{5}{3}\) \(\text { (d) } \frac{5}{4}\) (a) -
An astronomical telescope is an optical instrument which is used for observing distinct images of heavenly bodies libe stars, planets etc. It consists of two lenses. In normal adjustment of telescope, the final image is formed at infinity. Magnifying power of an astronomical telescope in normal adjustment is defined as the ratio of the angle subtended at the eye by the angle subtended at the eye by the final image to the angle subtended at the eye, by the object directly, when the final image and the object both lie at infinite distance from the eye. It is given by,\(m=\frac{f_{0}}{f_{e}}\) To increase magnifying power of an astronomical telescope in normal adjustment, focal length of objective lens should be large and focal length of eye lens should be small.
(i) An astronomical telescope of magnifying power 7 consists of the two thin lenses 40 cm apart, in normal adjustment. The focal lengths of the lenses are(a) 5cm,35cm (b) 7cm,35cm (c) 17cm,35cm (d) 5cm,30cm (ii) An astronomical telescope has a magnifying power of 10. In normal adjustment, distance between the objective and eye piece is 22 cm. The focal length of objective lens is
(a) 25 cm (b) 10 cm (c) 15 cm (d) 20 cm (iii) In astronomical telescope compare to eye piece, objective lens has
(a) negative focal length (b) zero focal length (c) small focal length (d) large focal length (iv) To see stars, use
(a) simple microscope (b) compound microscope (c) endoscope (d) astronomical telescope (v) For large magnifying power of astronomical telescope
\((a) f_{v}< \((b) f_{v}=f_{\mathrm{e}}\) \((c) f_{o}>>f_{\mathrm{e}}\) (d) none of these (a) -
Refraction of light is the change in the path oflight as it passes obliquely from one transparent medium to another medium. According to law of refraction \(\frac{\sin i}{\sin r}={ }^{1} \mu_{2}\) where \({ }^{1} \mu_{2}\) is called refractive index of second medium with respect to first medium. From refraction at a convex spherical surface, we have \(\frac{\mu_{2}}{v}-\frac{\mu_{1}}{u}=\frac{\mu_{2}-\mu_{1}}{R}\) Similarly from refraction at a concave spherical surface when object lies in the rarer medium, we have \(\frac{\mu_{2}}{v}-\frac{\mu_{1}}{u}=\frac{\mu_{2}-\mu_{1}}{R}\) and when object lies in the denser medium, we have \(\frac{\mu_{1}}{v}-\frac{\mu_{2}}{u}=\frac{\mu_{1}-\mu_{2}}{R}\).
(i) Refractive index of a medium depends upon(a) nature of the medium (b) wavelength of the light used (c) temperature (d) all of these (ii) A ray of light of frequency 5 x 1014 Hz is passed through a liquid. The wavelength of light measured inside the liquid is found to be 450 x 10-9 m. The refractive index of the liquid is
(a) 1.33 (b) 2.52 (c) 2.22 (d) 0.75 (iii) A ray of light is incident at an angle of 60° on one face of a rectangular glass slab of refractive index 1.5. The angle of refraction is
(a) sin-1(0.95) (b) sin-1(0.58) (c) sin-1(0.79) (d) sin-1(0.86) (iv) A point object is placed at the centre of a glass sphere of radius 6 cm and refractive index 1.5. The distance of the virtual image from the surface of sphere is
(a) 2 cm (b) 4 cm (c) 6 cm (d) 12 cm (v) In refraction, light waves are bent on passing from one medium to the second medium because in the second medium
(a) the frequency is different (b) the co-efficient of elasticity is different (c) the speed is different (d) the amplitude is smaller. (a) -
A compound microscope is an optical instrument used for observing highly magnified images of tiny objects. Magnifying power of a compound microscope is defined as the ratio of the angle subtended at the eye by the final image to the angle subtended at the eye by the object, when both the final image and the object are situated at the least distance of distinct vision from the eye. It can be given that:\(m=m_{e} \times m_{o}\) where me is magnification
produced by eye lens and mo is magnification produced by objective lens. Consider a compound microscope that consists of an objective lens of focal length 2.0 cm and an eyepiece of focal length 6.25 cm separated by a distance of 15 cm.
(i) The object distance for eye-piece, so that final image is formed at the least distance of distinct vision, will be(a) 3.45 cm (b) 5.cm (c) 1.29 cm (d) 2.59 cm (ii) How far from the objective should an object be placed in order to obtain the condition described in part(i)?
(a) 4.5 cm (b) 2.5 cm (c) 1.5 cm (d) 3.0 cm (iii) What is the magnifying power of the microscope in case ofleast distinct vision?
(a) 20 (b) 30 (c) 40 (d) 10 (iv) The intermediate image formed by the objective of a compound microscope is
(a) real, inverted and magnified (b) real, erect, and magnified (c) virtual, erect and magnified (d) virtual, inverted and magnified (v) The magnifying power of a compound microscope increases with
(a) the focal length of objective lens is increased and that of eye lens is decreased (b) the focal length of eye lens is increased and that of objective lens is decreased (c) focal lengths of both objects and eye-piece are increased (d) focal lengths of both objects and eye-piece are decreased. (a) -
The lens maker's formula relates the focal length of a lens to the refractive index of the lens material and the radii of curvature of its two surfaces. This formula is called so because it is used by manufacturers to design lenses of
required focal length from a glass of given refractive index. If the object is placed at infinity, the image will be formed at focus for both double convex lens and double concave lens
Therefore, lens maker's formula is \(\frac{1}{f}=\left[\frac{\mu_{2}-\mu_{1}}{\mu_{1}}\right]\left[\frac{1}{R_{1}}-\frac{1}{R_{2}}\right]\)
When lens is placed in air, \(\mu\) 1 = 1 and \(\mu\)2 = \(\mu\). The lens maker formula takes the form \(\frac{1}{f}=(\mu-1)\left[\frac{1}{R_{1}}-\frac{1}{R_{2}}\right]\)
(i) The radius of curvature of each face of biconcave lens with refractive index 1.5 is 30 cm. The focal length of the lens in air is(a) 12 cm (b) 10 cm (c) 20 cm (d) 30 cm (ii) The radii of curvature of the faces of a double convex lens are 10 cm and 1.5 cm. If focal length is 12 cm, then refractive index of glass is
(a) 1.5 (b) 1.78 (c) 2.0 (d) 2.52 (iii) An under-water swimmer cannot see very clearly even in absolutely clear water because of
(a) absorption oflight in water (b) scattering of light in water (c) reduction of speed of light in water (d) change in the focal length of eye-lens (iv) A thin lens of glass (\(\mu\) = 1.5) offocallength 10 cm is immersed in water (\(\mu\) = 1.33). The new focal length is
(a) 20 cm (b) 40 cm (c) 48 cm (d) 12 cm (v) An object is immersed in a fluid. In order that the object becomes invisible, it should
(a) behave as a perfect reflector (b) absorb all light falling on it (c) have refractive index one (d) have refractive index exactly matching with that of the surrounding fluid. (a) -
A prism is a portion of a transparent medium bounded by two plane faces inclined to each other at a suitable angle. A ray of light suffers two refractions on passing through a prism and hence deviates through a certain angle from its original path. The angle of deviation of a prism is, \(\delta\) = (\(\mu\)- 1) A, through which a ray deviates on passing through a thin prism of small refracting angle A.
If \(\mu\) is refractive index of the material of the prism, then prism formula is,\(\mu=\frac{\sin \left(A+\delta_{m}\right) / 2}{\sin A / 2}\)
(i) For which colour, angle of deviation is minimum?(a) Red (b) Yellow (c) Violet (d) Blue (ii) When white light moves through vacuum
(a) all colours have same speed (b) different colours have different speeds (c) violet has more speed than red (d) red has more speed than violet. (iii) The deviation through a prism is maximum when angle of incidence is
(a) 45° (b) 70° (c) 90° (d) 60° (iv) What is the deviation produced by a prism of angle 6°? (Refractive index of the material of the prism is 1.644).
(a) 3.864° (b): 4.595° (c) 7.259° (d) 1.252° (v) A ray of light falling at an angle of 50° is refracted through a prism and suffers minimum deviation. If the angle of prism is 60°, then the angle of minimum deviation is
(a) 45° (b) 75° (c) 50° (d) 40° (a) -
An optical fibre is a thin tube of transparent material that allows light to pass through, without being refracted into the air or another external medium. It make use of total internal reflection. These fibres are fabricated in such a way that light reflected at one side of the inner surface strikes the other at an angle larger than critical angle. Even, if fibre is bent, light can easily travel along the length.
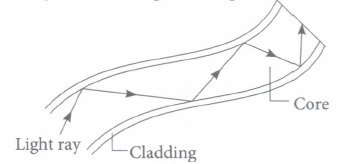
(i) Which of the following is based on the phenomenon of total internal reflection of light?(a) Sparkling of diamond (c) Instrument used by doctors for endoscopy (b) Optical fibre communication (d) All of these (ii) A ray of light will undergo rotal internal reflection inside the optical fibre, if it
(a) goes from rarer medium to denser medium (b) is incident at an angle less than the critical angle (c) strikes the interface normally (d) is incident at an angle greater than the critical angle (iii) If in core, angle of incidence is equal to critical angle, then angle of refraction will be
(a) 0° (b) 45° (c) 90 (d) 180° (iv) In an optical fibre (shown), correct relation for refractive indices of core and cladding is
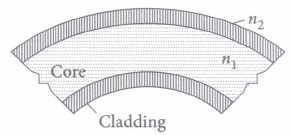
(a) n1 = n2 (b) n1 > n2 (c) n1 < n2 (d) n1 + n2 = 2 (v) If the value of critical angle is 30° for total internal reflection from given optical fibre, then speed of light in that fibre is
(a) 3 x 108 m S-1 (b) 1.5 x 108 m S-1 (c) 6 x 108 m s-1 (d) 4.5 x 108 m s-1 (a) -
(i) Explain what is meant by (a) total internal reflection (b) critical angle.
(ii) Figure below shows a ray of light, travelling in air, incident on a glass prism.
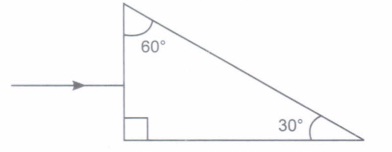
(a) The speed of light in air is 3.0 x 108 m/s. Its speed in the glass is 2.0 x 108 m/s. Calculate toe refractive index of the glass.
(b) On figure, draw carefully, without calculation, the continuation of the ray through the prism and into the air.
(c) Show that the critical angle for the glassair boundary is 42°.(a) -
A compound microscope consists of two convex lenses one acts as a magnifying lens and is known as an objective, and another lens is called an eyepiece. The two lenses work independently. Objective lens produces a magnified image of a tiny object O. This image is further modified by an eyepiece and final image is further magnified by an eyepiece and is seen at least distance of distinct vision.
(i) What type of image is produced by an objective?
(ii) Where would the first image have to be produced a by the objective relative to the eyepiece such that second magnified image is produced on the same side of the eyepiece as the first image? (First image distance = ue from the eyepiece).
(iii) If we have two microscopes with similar set of combination oflenses (i.e. for each fo = 1.25 cm; fe = 6.0 cm). But magnification produced by first microscope is higher than the second one. What might be the reason?(a)
Case Study
*****************************************
Answers
Ray Optics and Optical Instruments Case Study Questions With Answer Key Answer Keys
-
(i) (b): Each half lens will form an image in the same plane. The optic axes of the lenses are displaced
\(\frac{1}{v}-\frac{1}{(-30)}=\frac{1}{20} ; v=60 \mathrm{~cm}\)
(ii) (a): Here \(f_{1}=20 \mathrm{~cm} ; f_{2}=?\)
F= 80 cm
\(\text { As } \frac{1}{f_{1}}+\frac{1}{f_{2}}=\frac{1}{F} \Rightarrow \frac{1}{f_{2}}=\frac{1}{F}-\frac{1}{f_{1}}\)
\(\frac{1}{f_{2}}=\frac{1}{80}-\frac{1}{20}=\frac{-3}{80}\)
\(f_{2}=\frac{-80}{3}=-26.7 \mathrm{~cm}\)
(iii) (b): The bubble behaves libe a diverging lens
(iv) (b): Convex lens is used in magnifying glass.
(v) (d) -
(i) (d)
(ii) (a): \(P=P_{1}+P_{2}=1.5+1.0=2.5 \mathrm{D}\)
(iii) (b): \(f=\frac{1}{P}=\frac{1}{5} \mathrm{~m}=+20 \mathrm{~cm}\)
(iv) (a): \(P=P_{1}+P_{2}=\frac{1}{f_{1}}+\frac{1}{f_{2}}\)
\(=\frac{100}{10}+\frac{100}{-5}=-10 \mathrm{D}\)
(v) (b): \(P=P_{1}+P_{2}=\frac{100}{f_{1}}+\frac{100}{f_{2}}\)
\(P=\frac{100}{25}+\frac{100}{-20}=-1 \mathrm{D}\)
As the power is negative, the system will be diverging. -
(i) (a): \(\sin C=\frac{1}{\mu}=\frac{1}{3 / 2}=\frac{2}{3}=0.6667\)
\(C=\sin ^{-1}(0.6667)=41.8^{\circ}\)
(ii) (c): \(\mu=\frac{1}{\sin C}=\frac{1}{\sin 48.6}=\frac{1}{0.75}=\frac{4}{3}\)
(iii) (c): From \(\mu=\frac{1}{\sin C}, \sin C=\frac{1}{\mu}\)
\((\text { As } \mu_{v}>\mu_{r} \therefore C_{v}\)
The correct alternative may be (c).
(iv) (b): Difference between apparent and real depth of a pond is due to refraction. Other three are due to total internal reflection
(v) (c) :\(\text { As }^{w} \mu_{g}<^{a} \mu_{w}<^{a} \mu_{g} ; \therefore \theta>\theta_{2}>\theta_{1}\) -
(i) (C) : \(\frac{1}{f}=(\mu-1)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)\)
For equiconvex lens \(R_{1}=R, R_{2}=-R\)
\(\frac{1}{20}=\left(\frac{3}{2}-1\right)\left(\frac{2}{R}\right)=\frac{1}{R}\)
R = 20 cm
(ii) (a): When a lens is immersed in a medium whose refractive index is greater than that of the lens, its nature changes. Here the lens changes its nature when immersed in water it means its refractive index is less than that of water.
(iii) (a) : According to lens maker's formula
\(\frac{1}{f}=(n-1)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right) \therefore\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)=\frac{1}{f(n-1)}\)
(iv) (d): \(\frac{1}{f_{m}}=\left(\frac{\mu_{g}}{\mu_{m}}-1\right)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)\)
The given lens would behave as concave when fm becomes negative, for which \(\mu_{m}>\mu_{g}\)
Choice (d) is correct.
(v) (c): Here, R1 = 20 cm, R2 = -40 cm,f = 20 cm
Using lens maker's formula we get
\(\frac{1}{20}=(\mu-1)\left(\frac{1}{20}+\frac{1}{40}\right) ; \frac{1}{20}=(\mu-1) \frac{3}{40} \Rightarrow \mu=\frac{5}{3}\) -
(i) (a): \(m=\frac{f_{o}}{f_{e}}=7\)
\(f_{o}=7 f_{e}\)
In normal adjustment, distance between the lenses
\(f_{o}+f_{e}=40 \)
\(7 f_{0}+f_{e}=40 \Rightarrow f_{e}=\frac{40}{8}=5 \mathrm{~cm} \)
\(f_{o}=7 f_{e}=7 \times 5=35 \mathrm{~cm}\)
(ii) (d): \(m=-10 ; L=22 \mathrm{~cm}\)
\(\text { As } m=\frac{-f_{o}}{f_{e}} \Rightarrow-10=-\frac{f_{o}}{f_{e}}\)
\(f_{o}=10 f_{\mathrm{e}} \)
\(\text { As } L=f_{o}+f_{e} \)
\(22=10 f_{e}+f_{e}=11 f_{e} \)
\(\text { or } f_{e}=\frac{22}{11}=2 \mathrm{~cm}\)
\(f_{o}=10 f_{e}=20 \mathrm{~cm}\)
(iii) (d): Objective lens has larger focal length than eye-piece.
(iv) (d): Astronomial telescope is used to see stars, sun etc.
(v) (c) :f0>>fe -
(i) (d): Refractive index ofa medium depends upon nature and temperature of the medium, wavelength of light.
(ii) (a): Here \(v=5 \times 10^{14} \mathrm{~Hz} ; \lambda=450 \times 10^{-9} \mathrm{~m}\)
\(c=3 \times 10^{8} \mathrm{~m} \mathrm{~s}^{-1}\)
Refractive index of the liquid,
\(\mu=\frac{c}{v}=\frac{c}{v \lambda}=\frac{3 \times 10^{8}}{5 \times 10^{14} \times 450 \times 10^{-9}} \)
\(\mu=1.33\)
(iii) (b): Here i = 60° ; \(\mu\)= 1.5
By snell's law, \(\mu=\frac{\sin i}{\sin r}\)
\(\sin r=\frac{\sin i}{\mu}=\frac{\sin 60^{\circ}}{1.5}=\frac{0.866}{1.5} \)
\(\sin r=0.5773 \text { or } r=\sin ^{-1}(0.58)\)
(iv) (c): As object is at the centre of the sphere, the image must be at the centre only.
\(\therefore\) Distance of virtual image from centre of sphere = 6cm.
(v) (c): Speed of light in second medium is different than that in first medium. -
(i) (b): Here, \(f_{0}=2.0, f_{e}=6.25 \mathrm{~cm}, u_{0}=?\)
When the final image is obtained at the least distance of distinct vision:
Ve = - 25 cm
\(\text {As } \frac{1}{v_{e}}-\frac{1}{u_{e}}=\frac{1}{f_{e}} \)
\(\therefore \ \frac{1}{u_{e}}=\frac{1}{v_{e}}-\frac{1}{f_{e}}=\frac{1}{-25}-\frac{1}{6.25} \)
\(=\frac{-1-4}{25}=\frac{-5}{25}=-\frac{1}{5} \)
\(\text {or } u_{e}=-5 \mathrm{~cm}\)
(ii) (b): Distance between objective and eye-piece = 15cm
\(\therefore\) Distance of the image from objective is
\(v_{0}=15-5=10 \mathrm{~cm} \)
\(\therefore \quad \frac{1}{u_{0}}=\frac{1}{v_{0}}-\frac{1}{f_{0}}=\frac{1}{10}-\frac{1}{2}=\frac{1-5}{10}=-\frac{2}{5} \)
\(\text {or } \ u_{0}=-\frac{5}{2}=-2.5 \mathrm{~cm}\)
\(\therefore\) Distance of object from objective = 2.5 cm
(iii) (a): Magnifying power
\(m=m_{0} \times m_{e}=\frac{v_{0}}{u_{0}}\left(1+\frac{D}{f_{e}}\right)=\frac{10}{2.5}\left(1+\frac{25}{6.25}\right)=20\)
(iv) (a): The intermediate image formed, by the objective of a compound microscope is real, inverted and magnified.
(v) (d) -
(i) (d): Here, \(\mu=1.5 ; R_{1}=30 \mathrm{~cm}\)
R2 = -30 cm
\(\text { As } \frac{1}{f}=(\mu-1)\left[\frac{1}{R_{1}}-\frac{1}{R_{2}}\right] \)
\(=(1.5-1)\left[\frac{1}{30}-\frac{1}{-30}\right]=-0.5 \times \frac{2}{30}-\frac{-1}{30}\)
\(f=-30 \mathrm{~cm}\)
(ii) (a): Here, f= 12 cm ; R1 = 10 cm
R2 = -15 cm
\(\text { As } \frac{1}{f}=(\mu-1)\left[\frac{1}{R_{1}}-\frac{1}{R_{2}}\right] \)
\(\frac{1}{12}=(\mu-1)\left[\frac{1}{10}+\frac{1}{15}\right] \)
\(\mu=1.5\)
(iii) (d): The eye-lens is surrounded by a different medium than air. This will change the focal length of the eye-lens. The eye cannot accommodate all images as it would do in air.
(iv) (b): \(\frac{1}{f}=(1.5-1)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)\)
\(\text { and } \frac{1}{f_{w}}=\left(\frac{1.5}{1.33}-1\right)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right) \)
\(\frac{f_{w}}{f}=\frac{0.5 \times 1.33}{0.17}=4 \)
\(f_{w}=4 f=4 \times 10=40 \mathrm{~cm}\)
(v) (d): If the refractive index of two media are same,the surface of separation does not produce refraction or reflection which helps in visibility. -
(i) (a): Angle of deviation is minimum for the red colour.
(ii) (a): In vacuum all colours have same speed, because there is no dispersion of light in vacuum.
(iii) (c): The deviation is maximum when angle is 90°.
(iv) (a): \(A=6^{\circ} ; \mu=1.644\)
\(f=(\mu-1) A \)
\(f=(1.644-1) 6=0.644 \times 6 \)
\(\delta=3.864^{\circ}\)
(v) (d): \(i_{1}=50^{\circ} ; A=60^{\circ}, \delta_{m}=?\)
\(A+\delta_{m}=i_{1}+i_{2}=50^{\circ}+50^{\circ}=100^{\circ} \)
\(\delta_{m}=100^{\circ}-A=100-60^{\circ}=40^{\circ}\) -
(i) (d): Total internal reflection is the basis for following phenomenon:
(a) Sparkling of diamond.
(b) Optical fibre communication.
(c) Instrument used by doctors for endoscopy.
(ii) (d): Total internal reflection (TIR) is the phenomenon that involves the reflection of all the incident light off the boundary. TIR only takes place when both of the following two conditions are met:The light is in the more denser medium and approaching the less denser medium.The angle of incidence is greater than the critical angle.
(iii) (c) : If incidence of angle, i = critical angle e, then angle of refraction, r = \(90^{\circ}\)
(iv) (b): In optical fibres, core is surrounded by cladding, where the refractive index of the material of the core is higher than that of cladding to bound the light rays inside the core.
(v) (b): From Snell's law, \(\sin C={ }_{1} n_{2}=\frac{v_{1}}{v_{2}}\)
where, c = critical angle = 30° and V1 and V2 are speed oflight in medium and vacuum, respectively.
We know that, v2 = 3 x 108 m s-l
\(\therefore \quad \sin 30^{\circ}=\frac{v_{1}}{3 \times 10^{8}} \)
\(\Rightarrow \quad v_{1}=3 \times 10^{8} \times \frac{1}{2} \Rightarrow v_{1}=1.5 \times 10^{8} \mathrm{~ms}^{-1} \) -
(i) (a) Important Terms and Concepts,
Total Internal Reflection
When the angle of incidence is increased then for some angle of incidence ie called the critical angle, the angle of refraction will be 90°. If the angle of incidence is increased beyond ie., the incident ray is reflected back in the denser medium and obeys the laws of reflection. This is known as total internal reflection. The following are the necessary conditions for total internal reflection to take place.
(b) Critical angle: It is the angle of incidence in denser medium for which angle of refraction in rarer medium is 90°
(ii) (a) \(\because \text { R.I. of glass }=\frac{\text { Speed of light in air }}{\text { Speed of light in glass }}\)
\(=\frac{3 \times 10^{8}}{2 \times 10^{8}}=1.5\)
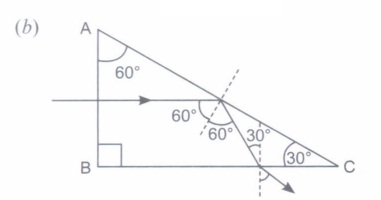
\(\because\) i = 60° is greater than critical angle = 42°
\(\because\) Total internal reflection will occur on face AC.
But on face BC of a prism; angle of incidence is 30°; therefore, ray of light will undergo refraction and bends away from normal.
(c) \(\because \ \text { R.I }=n=\frac{1}{\sin i_{c}}\)
here ic = critical angle
\(\therefore \ \sin i_{c}=\frac{1}{n}=\frac{1}{1.5}\)
\(\therefore \ \sin i_{c}=\frac{2}{3}=0.67\)
\(\because\) sin 42° = 0.67 (app.)
\(\therefore\) ic = 42° -
(i) Real, magnified and inverted image.
(ii) The image produced by the objective lens should either be formed at focus of eyepiece or between focus and eyepiece.
(iii) If image formed by the object is placed between focus of eyepiece and an eyepiece; then magnifying power is m1 = \(\frac{v_{0}}{-u_{0}} \cdot\left(1+\frac{D}{f_{e}}\right)\) which the case of first microscope.
But in case of second microscope, if image formed by objective is formed at the focus of eyepiece, then final image is seen at infinity and angular magnification produced will be \(m_{2}=\frac{v_{0}}{-u_{0}} \cdot \frac{D}{f_{e}}\) [ m2 < m1].






































